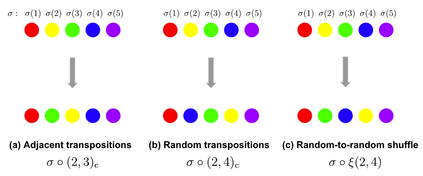
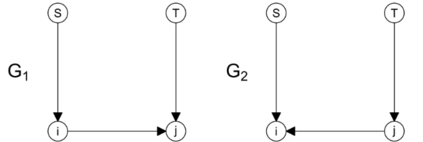
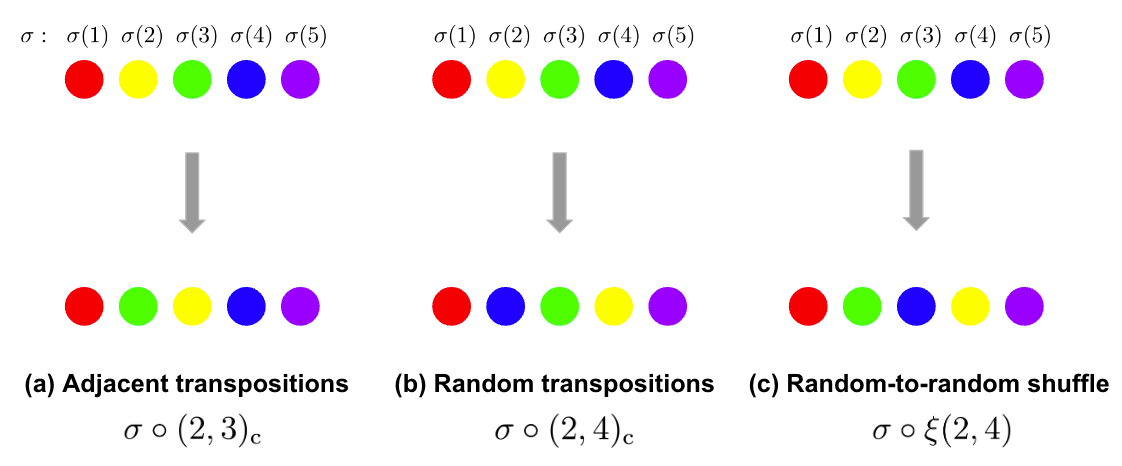
We propose an empirical Bayes formulation of the structure learning problem, where the prior specification assumes that all node variables have the same error variance, an assumption known to ensure the identifiability of the underlying causal directed acyclic graph (DAG). To facilitate efficient posterior computation, we approximate the posterior probability of each ordering by that of a best DAG model, which naturally leads to an order-based Markov chain Monte Carlo (MCMC) algorithm. Strong selection consistency for our model in high-dimensional settings is proved under a condition that allows heterogeneous error variances, and the mixing behavior of our sampler is theoretically investigated. Further, we propose a new iterative top-down algorithm, which quickly yields an approximate solution to the structure learning problem and can be used to initialize the MCMC sampler. We demonstrate that our method outperforms other state-of-the-art algorithms under various simulation settings, and conclude the paper with a single-cell real-data study illustrating practical advantages of the proposed method.
翻译:暂无翻译