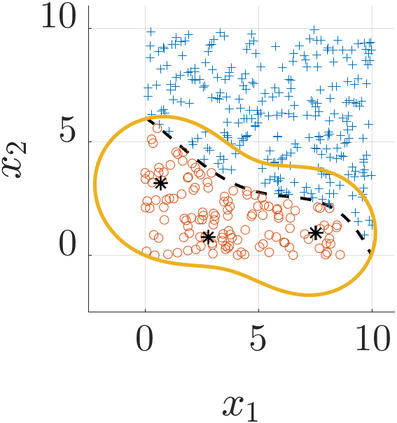
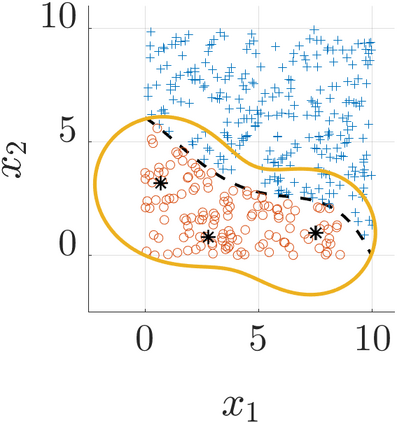
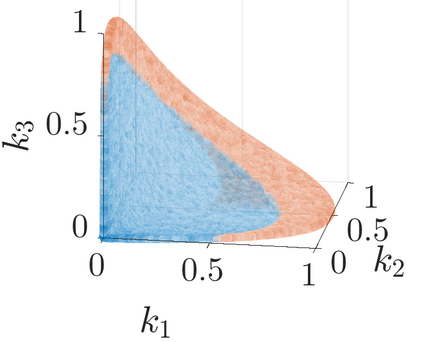
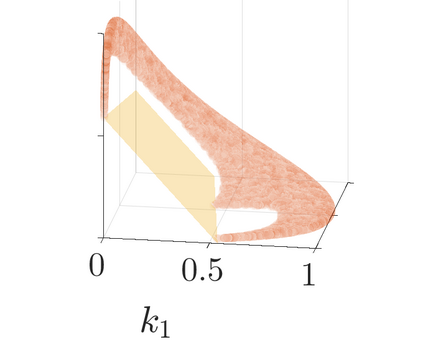
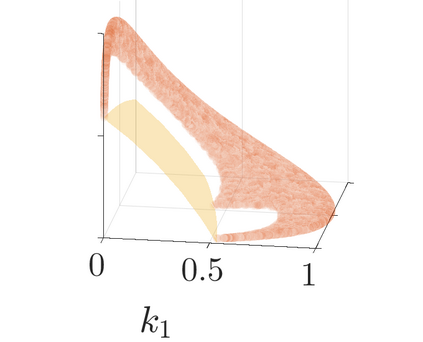
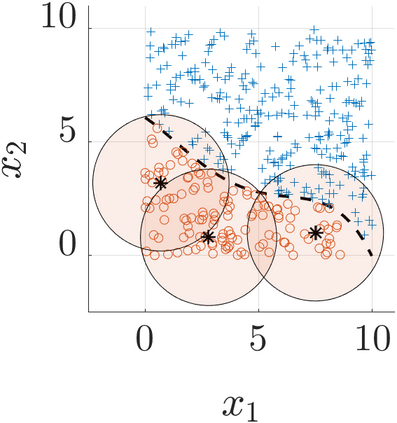
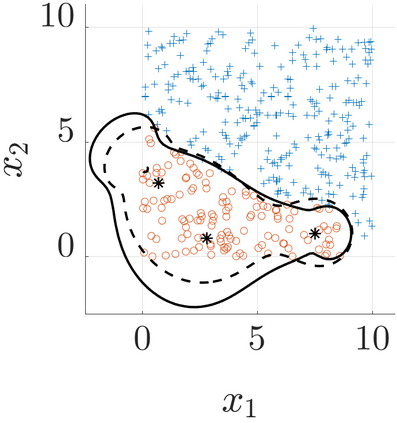
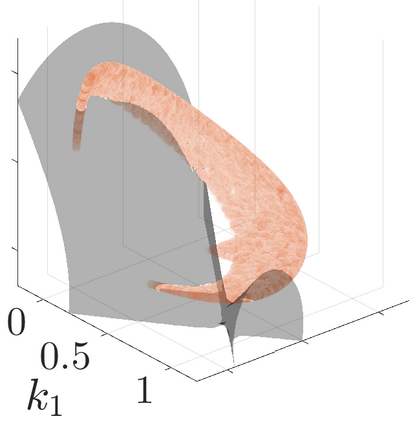
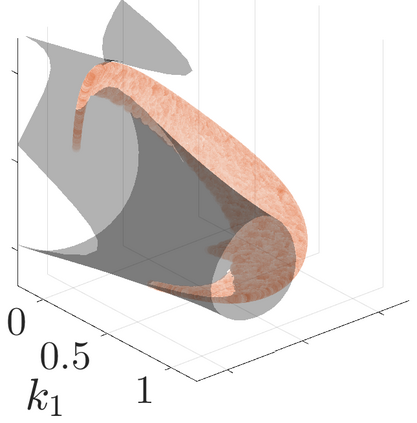
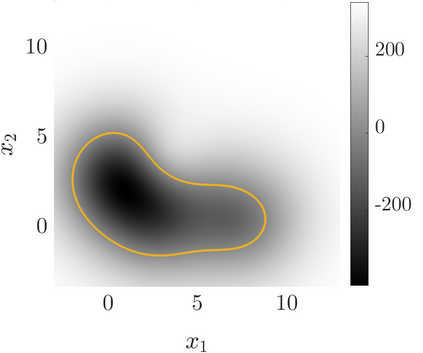
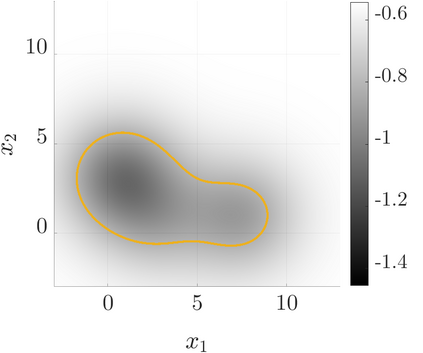
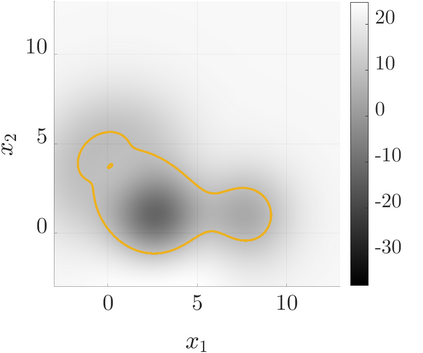
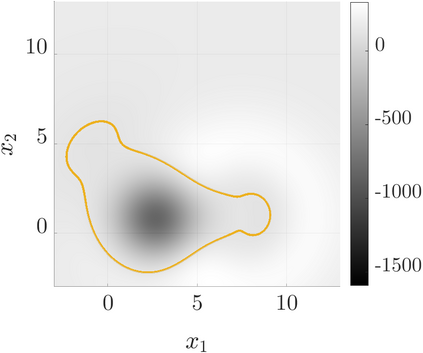
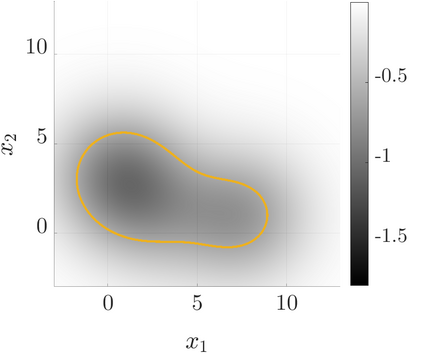
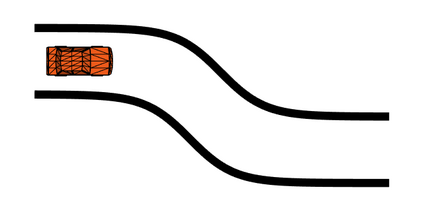
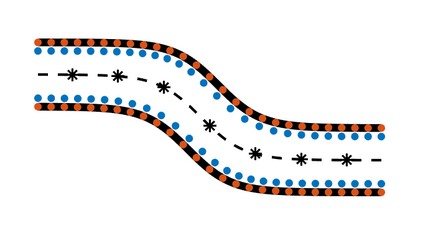
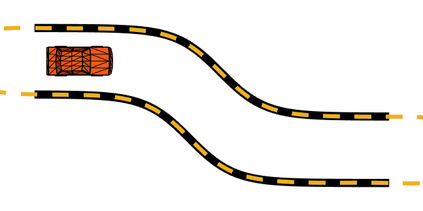
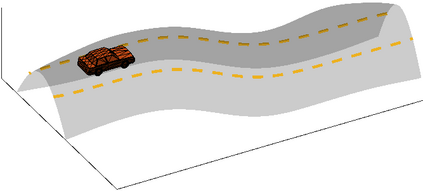
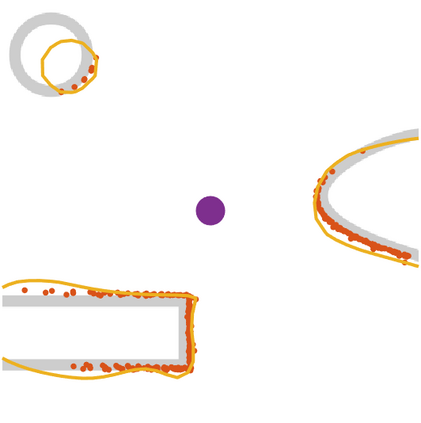
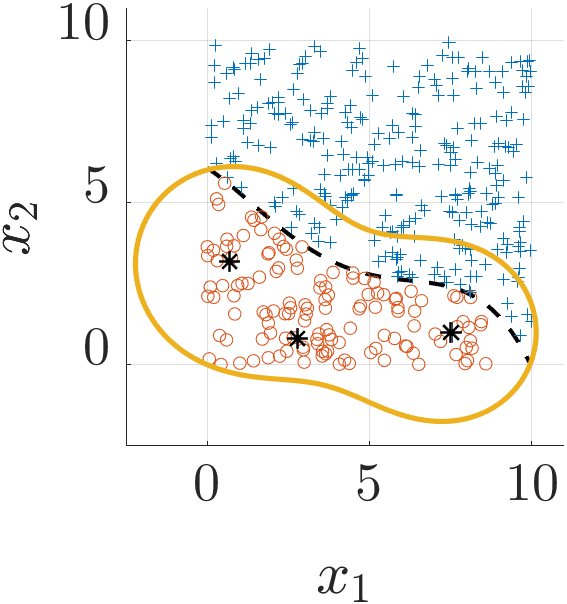
Barrier function-based inequality constraints are a means to enforce safety specifications for control systems. When used in conjunction with a convex optimization program, they provide a computationally efficient method to enforce safety for the general class of control-affine systems. One of the main assumptions when taking this approach is the a priori knowledge of the barrier function itself, i.e., knowledge of the safe set. In the context of navigation through unknown environments where the locally safe set evolves with time, such knowledge does not exist. This manuscript focuses on the synthesis of a zeroing barrier function characterizing the safe set based on safe and unsafe sample measurements, e.g., from perception data in navigation applications. Prior work formulated a supervised machine learning algorithm whose solution guaranteed the construction of a zeroing barrier function with specific level-set properties. However, it did not explore the geometry of the neural network design used for the synthesis process. This manuscript describes the specific geometry of the neural network used for zeroing barrier function synthesis, and shows how the network provides the necessary representation for splitting the state space into safe and unsafe regions.
翻译:基于障碍函数的不等式约束是实现控制系统安全规范的一种手段。当与凸优化程序结合使用时,它们为控制亚纳系统的一般类提供了强制安全性的计算效率高的方法。在接近未知环境的情况下,当本地安全区随时间变化时,这种方法的一个主要假设是先验知识障碍函数本身,即安全集的知识。在该文中,我们针对基于安全和不安全样本测量(例如导航应用中来自感知数据的测量)的零障碍函数综合,致力于构建描述安全集的零障碍函数。先前的工作制定了一种监督机器学习算法,其解决方案保证了具有特定级别集属性的零障碍函数的构造。然而,它没有探索用于综合过程的神经网络设计的几何性质。该文介绍了用于零障碍函数综合的神经网络的特定几何形状,并展示了该网络如何提供必要的表示,以将状态空间划分为安全和不安全的区域。