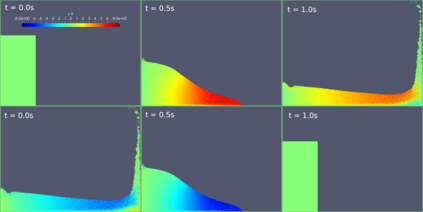
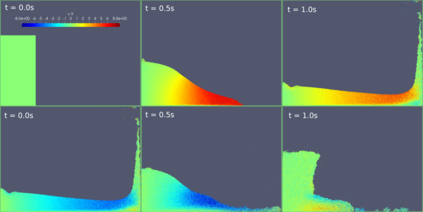
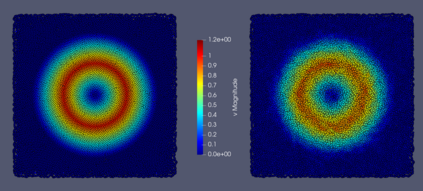
This paper describes an energy-preserving and globally time-reversible code for weakly compressible smoothed particle hydrodynamics (SPH). We do not add any additional dynamics to the Monaghan's original SPH scheme at the level of ordinary differential equation, but we show how to discretize the equations by using a corrected expression for density and by invoking a symplectic integrator. Moreover, to achieve the global-in-time reversibility, we have to correct the initial state, implement a conservative fluid-wall interaction, and use the fixed-point arithmetic. Although the numerical scheme is reversible globally in time (solvable backwards in time while recovering the initial conditions), we observe thermalization of the particle velocities and growth of the Boltzmann entropy. In other words, when we do not see all the possible details, as in the Boltzmann entropy, which depends only on the one-particle distribution function, we observe the emergence of the second law of thermodynamics (irreversible behavior) from purely reversible dynamics.
翻译:本文描述了一种节能和全球时间可逆代码,用于微弱压缩的平流粒体流体动力学(SPH) 。 我们没有在普通差分方程水平上为Monaghan原SPH计划增加任何额外的动态,但我们展示了如何通过对密度进行校正的表达方式和通过调试整合器将方程式分解。 此外,为了实现全球在时间上的可逆性,我们必须纠正初始状态,实施保守的流体-墙互动,并使用固定点算术。 尽管数字方法可以及时(在恢复初始条件时可以逆向), 我们观察了Boltzmann entropy 粒子速度和生长的热化。 换句话说, 当我们看不到所有可能的细节时, 如在Boltzmann entropy, 仅仅取决于单粒子分布功能, 我们观察到了热力学(不可逆性行为)第二定律(不可逆性行为)的出现, 来自于完全可逆的动态。