Optimal geometric multigrid preconditioners for HDG-P0 schemes for the reaction-diffusion equation and the generalized Stokes equations

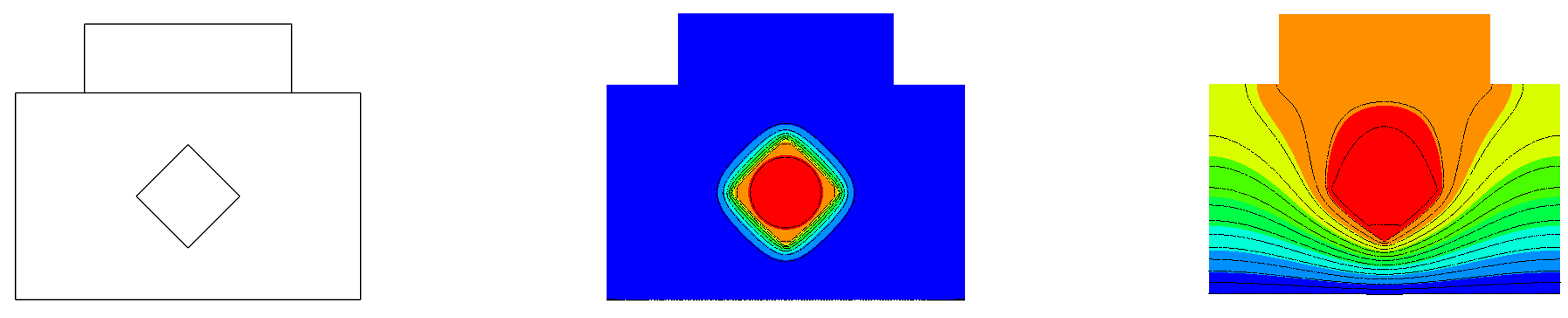
We present the lowest-order hybridizable discontinuous Galerkin schemes with numerical integration, denoted as HDG-P0, for the reaction-diffusion equation and the generalized Stokes equations in two- and three-dimensions. Here by lowest order, we mean that the (hybrid) finite element space for the global HDG facet degrees of freedom (DOFs) is the space of piecewise constants on the mesh skeleton. We give the optimal a priori error analysis of the proposed HDG-P0 schemes, which hasn't appeared in the literature yet for HDG discretizations as far as numerical integration is concerned. Moreover, we propose optimal geometric multigrid preconditioners for the statically condensed HDG-P0 linear systems. In both cases, we first establish the equivalence of the statically condensed HDG system with a (slightly modified) nonconforming Crouzeix-Raviart (CR) discretization, where the global (piecewise-constant) HDG finite element space on the mesh skeleton has a natural one-to-one correspondence to the nonconforming CR (piecewise-linear) finite element space that live on the whole mesh. This equivalence then allows us to use the well-established nonconforming geometry multigrid theory to precondition the condensed HDG system. Numerical results in two- and three-dimensions are presented to verify our theoretical findings.