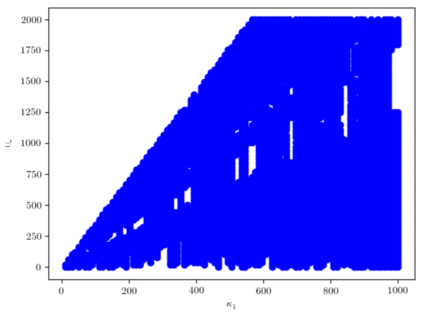
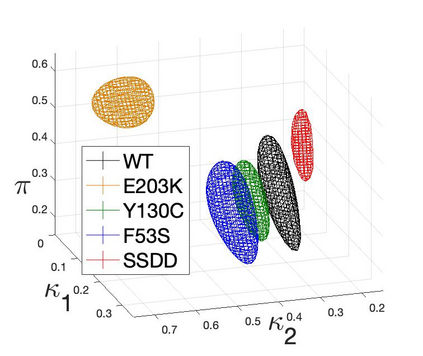
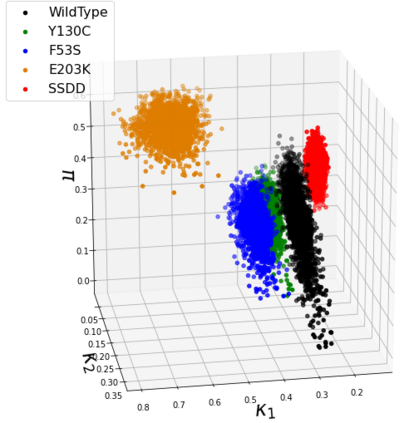
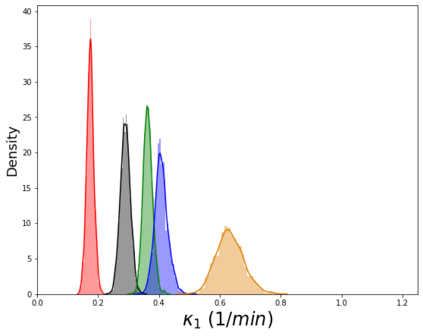
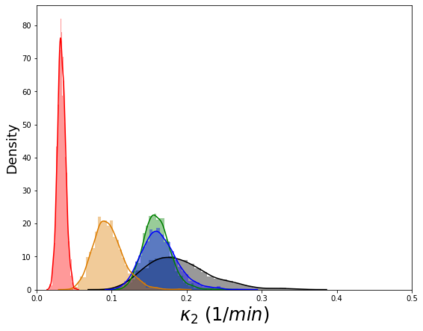
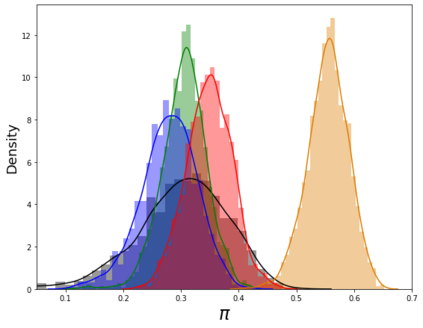
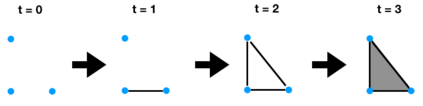
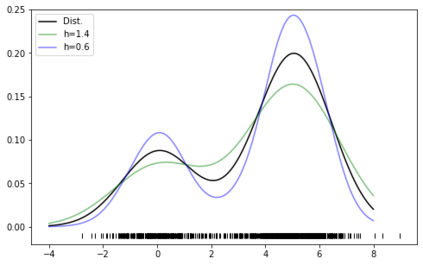
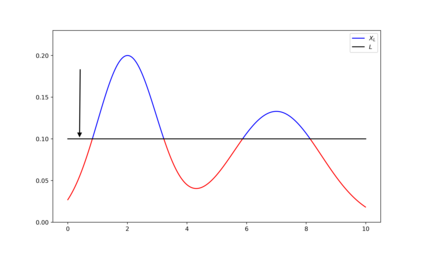
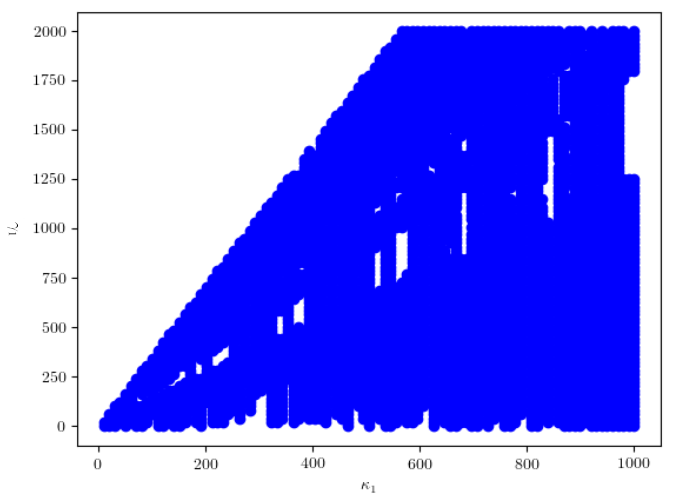
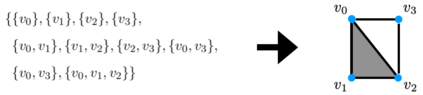The MEK/ERK signalling pathway is involved in cell division, cell specialisation, survival and cell death. Here we study a polynomial dynamical system describing the dynamics of MEK/ERK proposed by Yeung et al. with their experimental setup, data and known biological information. The experimental dataset is a time-course of ERK measurements in different phosphorylation states following activation of either wild-type MEK or MEK mutations associated with cancer or developmental defects. We demonstrate how methods from computational algebraic geometry, differential algebra, Bayesian statistics and computational algebraic topology can inform the model reduction, identification and parameter inference of MEK variants, respectively. Throughout, we show how this algebraic viewpoint offers a rigorous and systematic analysis of such models.
翻译:MEK/ERK 信号传输路径涉及细胞分裂、细胞专业化、生存和细胞死亡。在这里,我们研究由Yeung 等人提议的描述MEK/ERK动态的多元动态系统及其实验设置、数据和已知生物信息。实验数据集是不同磷酸化状态中ERK测量的时间过程,在激活与癌症或发育缺陷有关的野型MEK或MEK突变后进行测量。我们演示了计算代数几何、差位代数、巴耶西亚统计和计算代数表层学的方法如何分别为MEK变异模型的减少、识别和参数推断提供参考。我们始终展示了这种代数观点如何为此类模型提供严格和系统的分析。