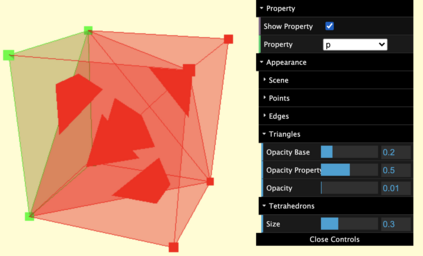
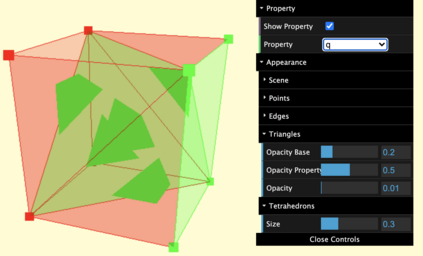
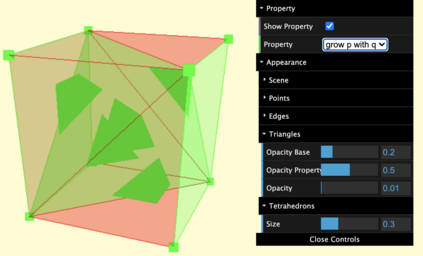
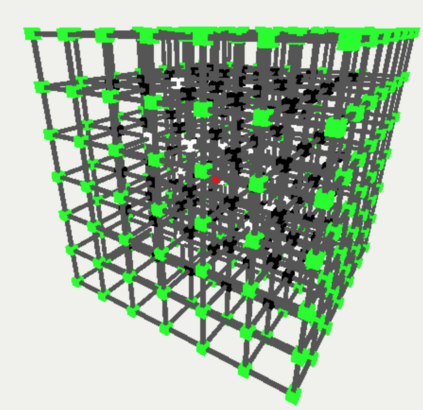
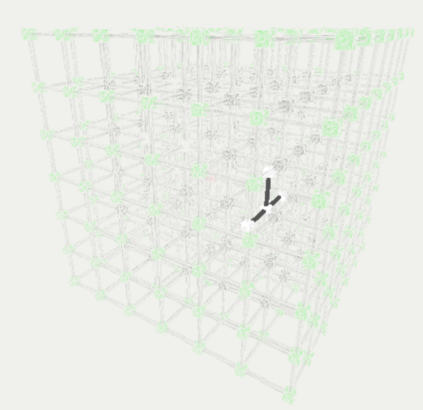
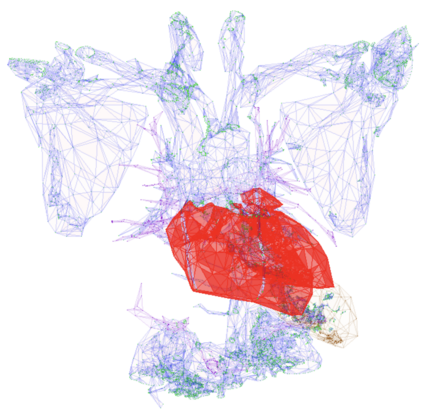
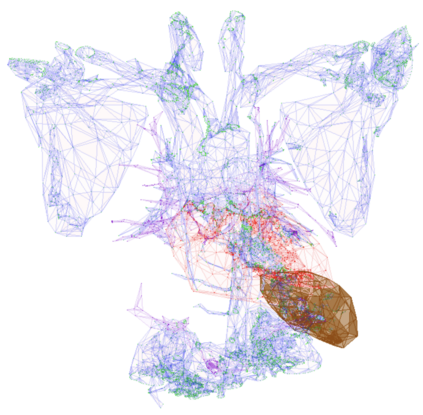
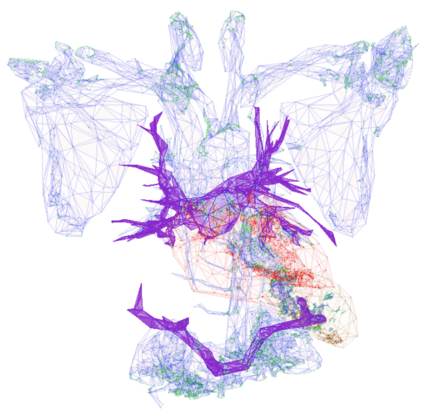
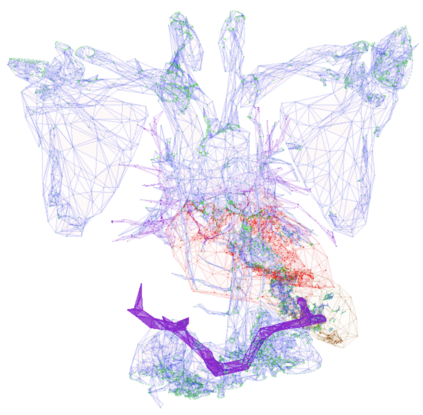
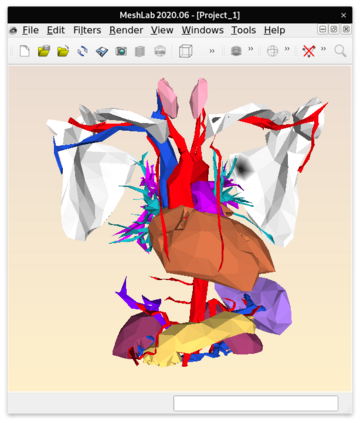
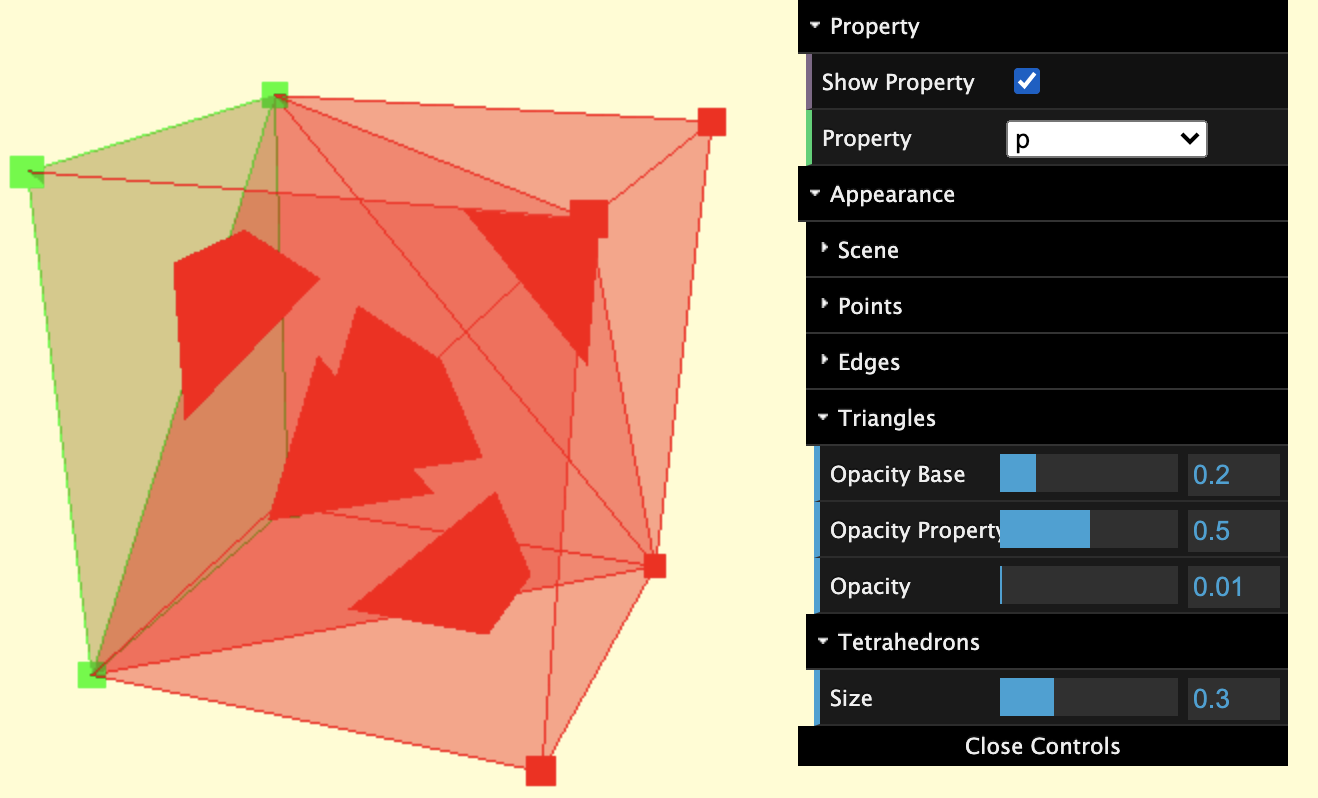
Topological Spatial Model Checking is a recent paradigm where model checking techniques are developed for the topological interpretation of Modal Logic. The Spatial Logic of Closure Spaces, SLCS, extends Modal Logic with reachability connectives that, in turn, can be used for expressing interesting spatial properties, such as "being near to" or "being surrounded by". SLCS constitutes the kernel of a solid logical framework for reasoning about discrete space, such as graphs and digital images, interpreted as quasi discrete closure spaces. Following a recently developed geometric semantics of Modal Logic, we propose an interpretation of SLCS in continuous space, admitting a geometric spatial model checking procedure, by resorting to models based on polyhedra. Such representations of space are increasingly relevant in many domains of application, due to recent developments of 3D scanning and visualisation techniques that exploit mesh processing. We introduce PolyLogicA, a geometric spatial model checker for SLCS formulas on polyhedra and demonstrate feasibility of our approach on two 3D polyhedral models of realistic size. Finally, we introduce a geometric definition of bisimilarity, proving that it characterises logical equivalence.
翻译:地形空间模型检查是最近为对摩达尔逻辑进行地形解释而开发模型检查技术的一种范例。封闭空间空间的空间逻辑(SLCS)扩展了具有可达性连接的模型逻辑(Modal Lologic),扩展了具有可达性连接的模型逻辑(modal Lologic),反过来,这些模型逻辑可以用来表达有趣的空间特性,例如“接近”或“被环绕着的”空间特性。SLCS构成关于离散空间推理的坚实逻辑框架的核心,例如图表和数字图像,被解释为准离散封闭空间。在最近开发了摩达尔逻辑的几何语义之后,我们建议对连续空间的SLCS进行解释,采用基于多元赫德拉的模型的几何空间模型检查程序。由于最近开发了3D扫描和视觉技术,利用网状处理,这种空间的表达方式在许多应用领域变得日益相关。我们引入了三维扫描和可视化技术。我们引入了PolyLogicA(PollyLogicA),这是对多元体积尺寸的两种3D多边模型的测试,并展示了我们的方法的可行性。最后我们引入了对等等的逻辑定义。
相关内容
Source: Apple - iOS 8