Proper Selection of Obreshkov-Like Numerical Integrators Used as Numerical Differentiators

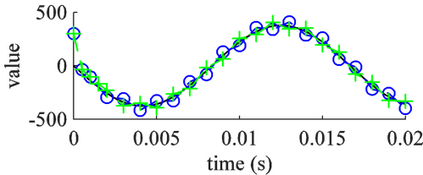
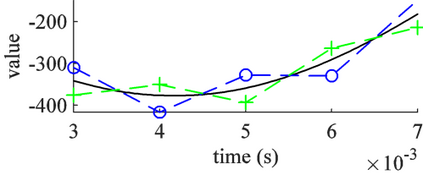
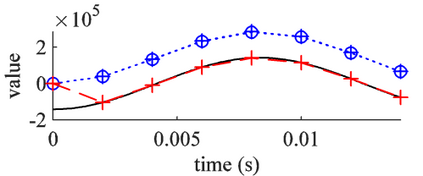
Criteria for Obreshkov-like numerical integrators to be used as numerical differentiators are proposed in this paper. The coefficients of a numerical integrator for the highest order derivative turn out to determine its suitability and potential hazards such as numerical oscillation and bias. The suitability of some existing Obreshkov-like numerical integrators is examined. It is revealed that the notorious numerical oscillations induced by the implicit trapezoidal method cannot always be eliminated by using the backward Euler method for a few time steps. Guided by the proposed criteria, a frequency response optimized integrator considering second order derivative is put forward which is suitable to be used as a numerical differentiator. Theoretical observations are verified in time domain via case studies.