A Bayesian approach for inverse potential problem with topological-Gaussian prior

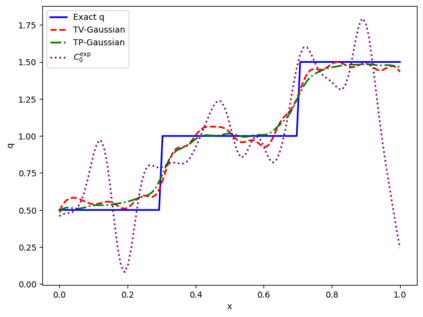
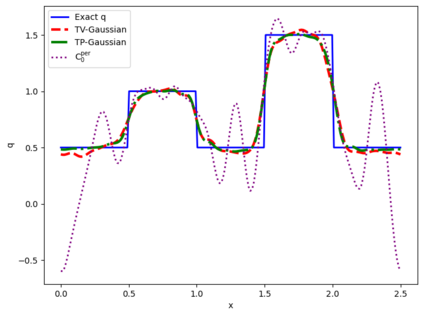
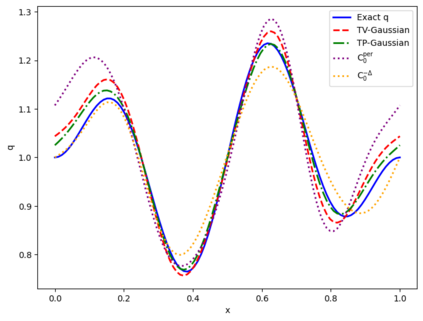
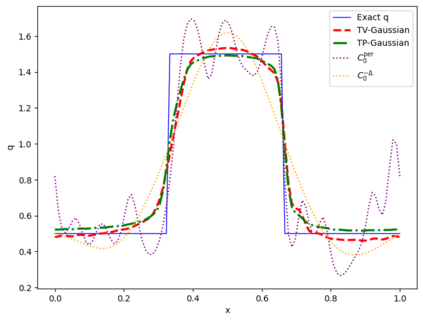
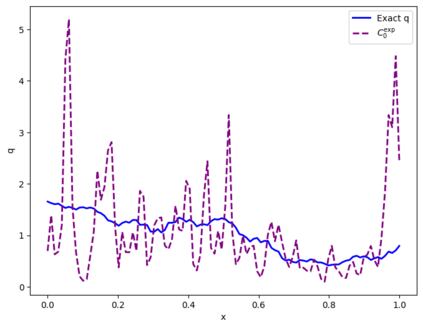
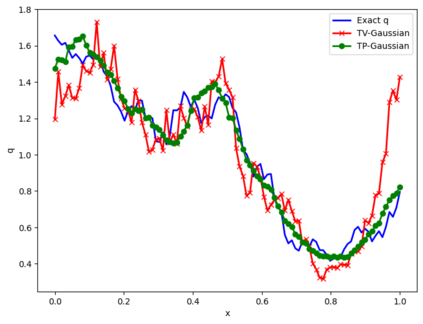
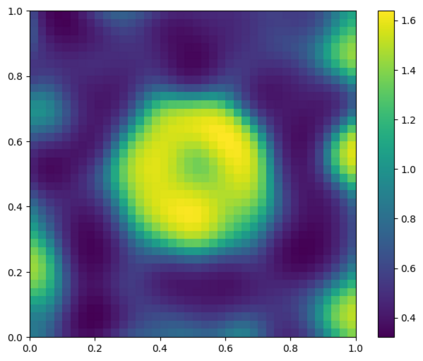
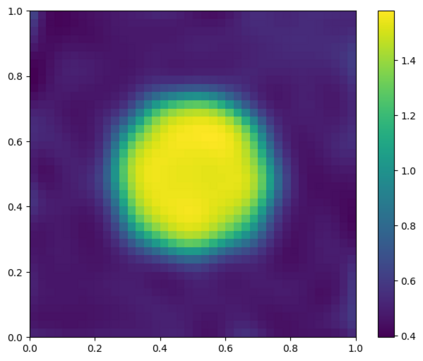
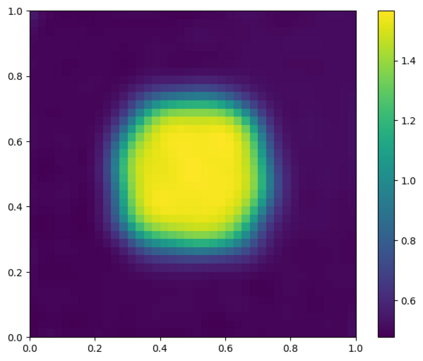
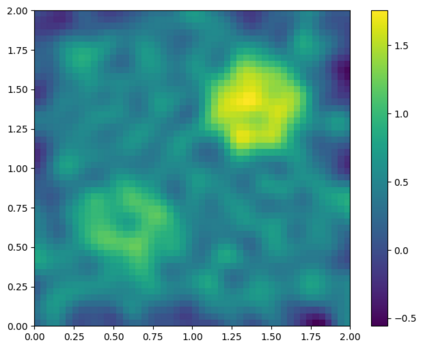
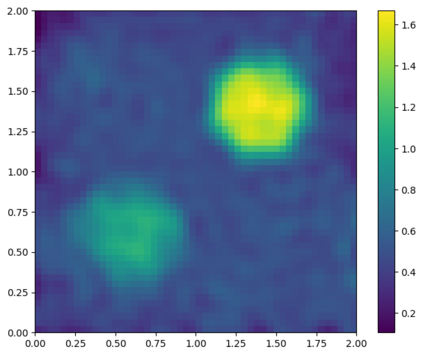
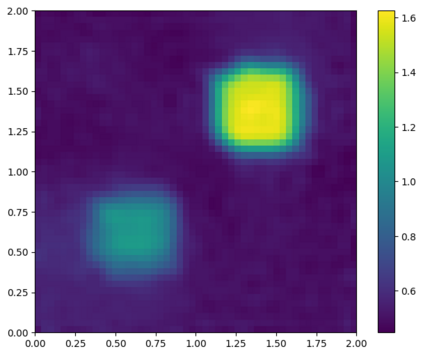
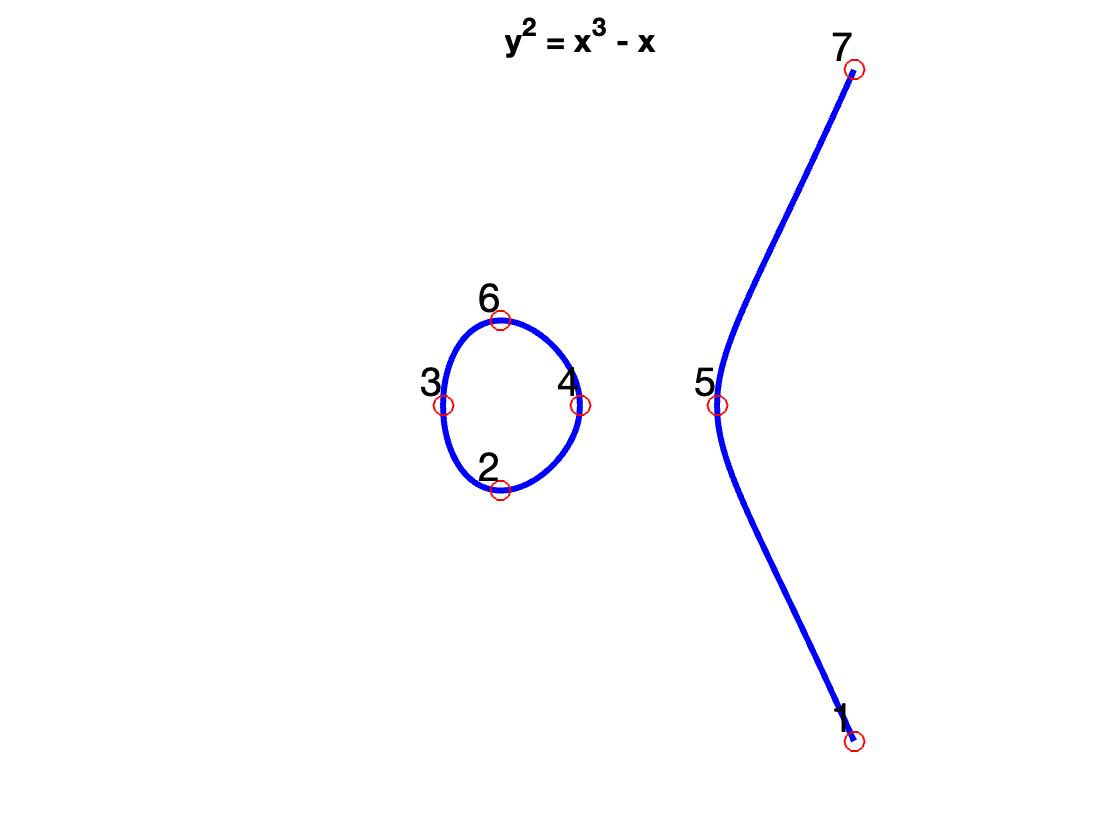
This paper addresses the reconstruction of a potential coefficient in an elliptic problem from distributed observations within the Bayesian framework. In such problems, the selection of an appropriate prior distribution is crucial, particularly when the function to be inferred exhibits sharp discontinuities, as traditional Gaussian priors often prove inadequate. To tackle this challenge, we develop the topological prior (TP), a new prior constructed using persistent homology. The proposed prior utilizes persistent pairs to characterize and record the topological variations of the functions under reconstruction, thereby encoding prior information about the structure and discontinuities of the function. The TP prior, however, only exists in a discretized formulation, which leads to the absence of a well-defined posterior measure in function spaces. To resolve this issue, we propose a TP-Gaussian hybrid prior, where the TP component detects sharp discontinuities in the function, while the Gaussian distribution acts as a reference measure, ensuring a well-defined posterior measure in the function space. The proposed TP prior demonstrates effects similar to the classical total variation (TV) prior but offers greater flexibility and broader applicability due to three key advantages. First, it is defined on a general topological space, making it easily adaptable to a wider range of applications. Second, the persistent distance captures richer topological information compared to the discrete TV prior. Third, it incorporates more adjustable parameters, providing enhanced flexibility to achieve robust numerical results. These features make the TP prior a powerful tool for addressing inverse problems involving functions with sharp discontinuities.