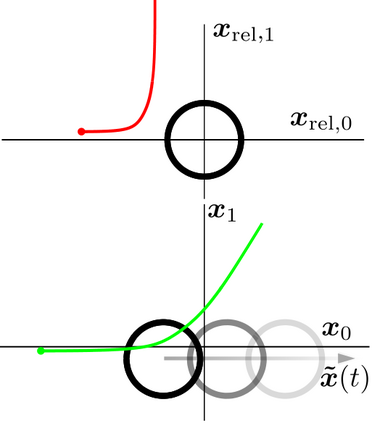
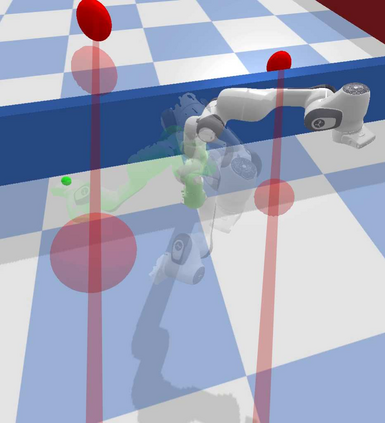
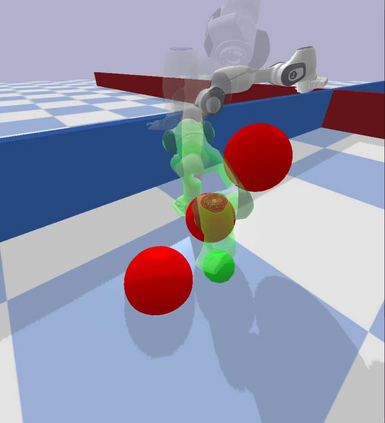
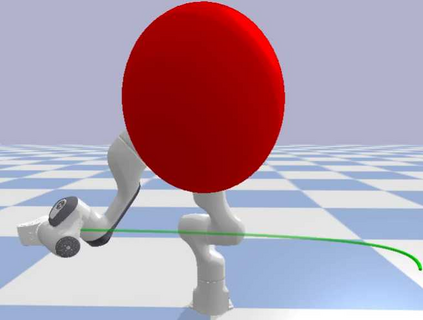
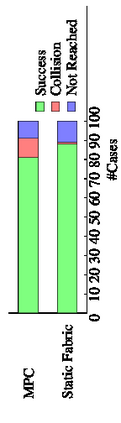
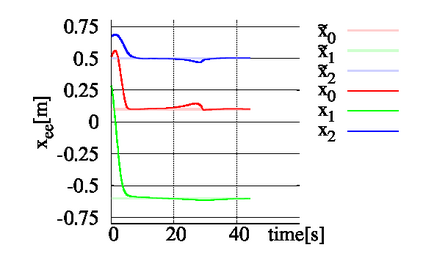
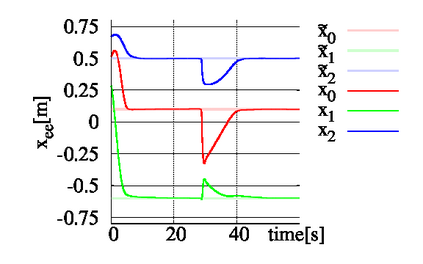
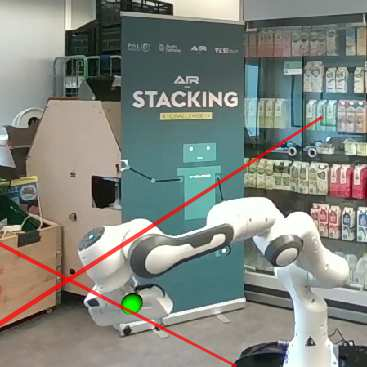
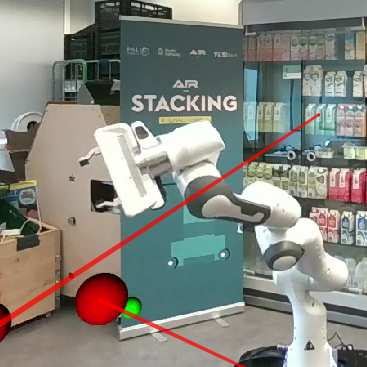
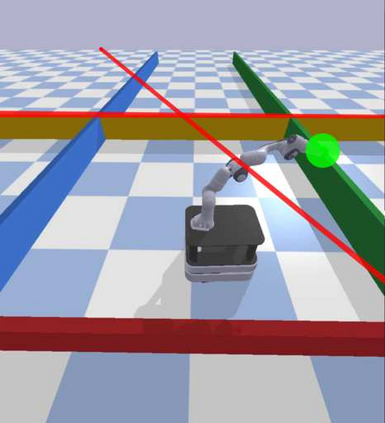
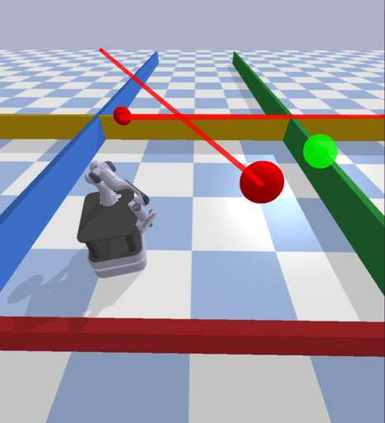
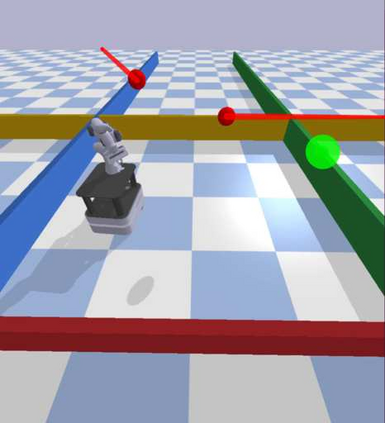
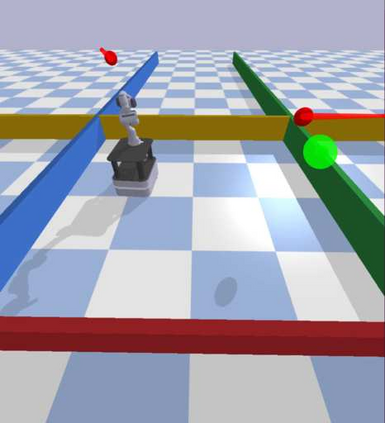
Optimization fabrics represent a geometric approach to real-time motion planning, where trajectories are designed by the composition of several differential equations that exhibit a desired motion behavior. We generalize this framework to dynamic scenarios and prove that fundamental properties can be conserved. We show that convergence to trajectories and avoidance of moving obstacles can be guaranteed using simple construction rules of the components. Additionally, we present the first quantitative comparisons between optimization fabrics and model predictive control and show that optimization fabrics can generate similar trajectories with better scalability, and thus, much higher replanning frequency (up to 500 Hz with a 7 degrees of freedom robotic arm). Finally, we present empirical results on several robots, including a non-holonomic mobile manipulator with 10 degrees of freedom, supporting the theoretical findings.
翻译:优化织物是实时运动规划的一种几何方法,在这种方法中,轨迹是由几个不同方程式构成而设计的,这些方程式表现出一种理想的运动行为。我们将这一框架推广到动态假设中,并证明基本属性是可以保护的。我们表明,使用部件的简单构造规则可以保证与轨迹的趋同和避免移动障碍。此外,我们介绍了优化织物和模型预测控制之间的第一次定量比较,并表明优化织物可以产生类似的轨迹,其可更便于伸缩,因此,再规划频率要高得多(最多500赫兹,拥有7度自由机器人臂 )。最后,我们介绍了数个机器人的经验结果,包括一个10度自由的非热缩移动操纵器,支持理论发现。