The Greggs-Pret Index: a Machine Learning analysis of consumer habits as a metric for the socio-economic North-South divide in England

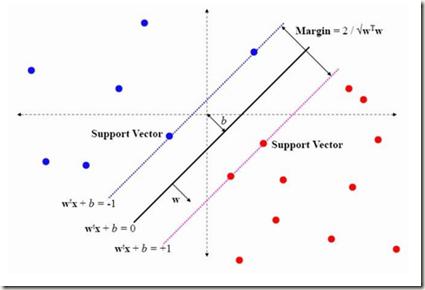
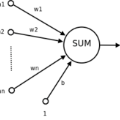
In England, it is anecdotally remarked that the number of Greggs bakeries to be found in a town is a reliable measure of the area's 'Northern-ness'. Conversely, a commercial competitor to Greggs in the baked goods and sandwiches market, Pret-a-Manger, is reputed to be popular in more 'southern' areas of England. Using a Support Vector Machine and an Artificial Neural Network (ANN) Regression Model, the relative geographical distributions of Greggs and Pret have been utilised for the first time to quantify the North-South divide in England. The calculated dividing lines were each compared to another line, based on Gross Domestic Household Income (GDHI). The lines match remarkably well, and we conclude that this is likely because much of England's wealth is concentrated in London, as are most of England's Pret-a-Manger shops. Further studies were conducted based on the relative geographical distributions of popular supermarkets Morrisons and Waitrose, which are also considered to have a North-South association. This analysis yields different results. For all metrics, the North-South dividing line passes close to the M1 Watford Gap services. As a common British idiom, this location is oft quoted as one point along the English North-South divide, and it is notable that this work agrees. This tongue-in-cheek analysis aims to highlight more serious factors highlighting the North-South divide, such as life expectancy, education, and poverty.