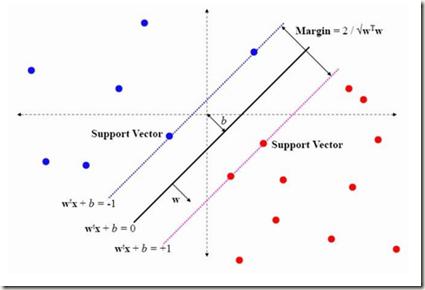
Let $A$ be a real $n\times n$ matrix and $z,b\in \mathbb R^n$. The piecewise linear equation system $z-A\vert z\vert = b$ is called an absolute value equation. It is equivalent to the general linear complementarity problem, and thus NP hard in general. Concerning the latter problem, three solvers are presented: One direct, one semi-iterative and one discrete variant of damped Newton. Their previously proved ranges of correctness and convergence, respectively, are extended. Their performance is compared on instances of the XOR separation problem for support vector machines which can be reformulated as an absolute value equation.
翻译:让$A 成为真实的$n\timen n$ 矩阵和 $z,b\ in\mathb R ⁇ n$。 prapwise 线性方程系统 $z-A\vert z\vert = b$ 被称为绝对值方程。它相当于一般的线性互补问题,因此一般来说是NP硬的。关于后一个问题,提出了三个解答者: 被压断的牛顿的一个直接的、 一个半印式的和一个独立的变异体。它们先前证明的正确性和趋同范围分别被扩大。它们的性能可以比较支持矢量机的 XOR 分离问题, 支持矢量机可以重新拟订为绝对值方程。