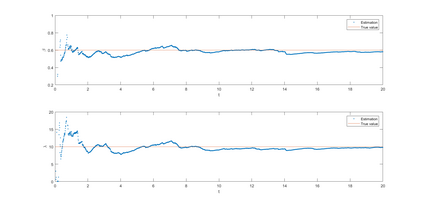
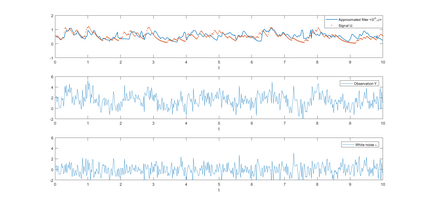
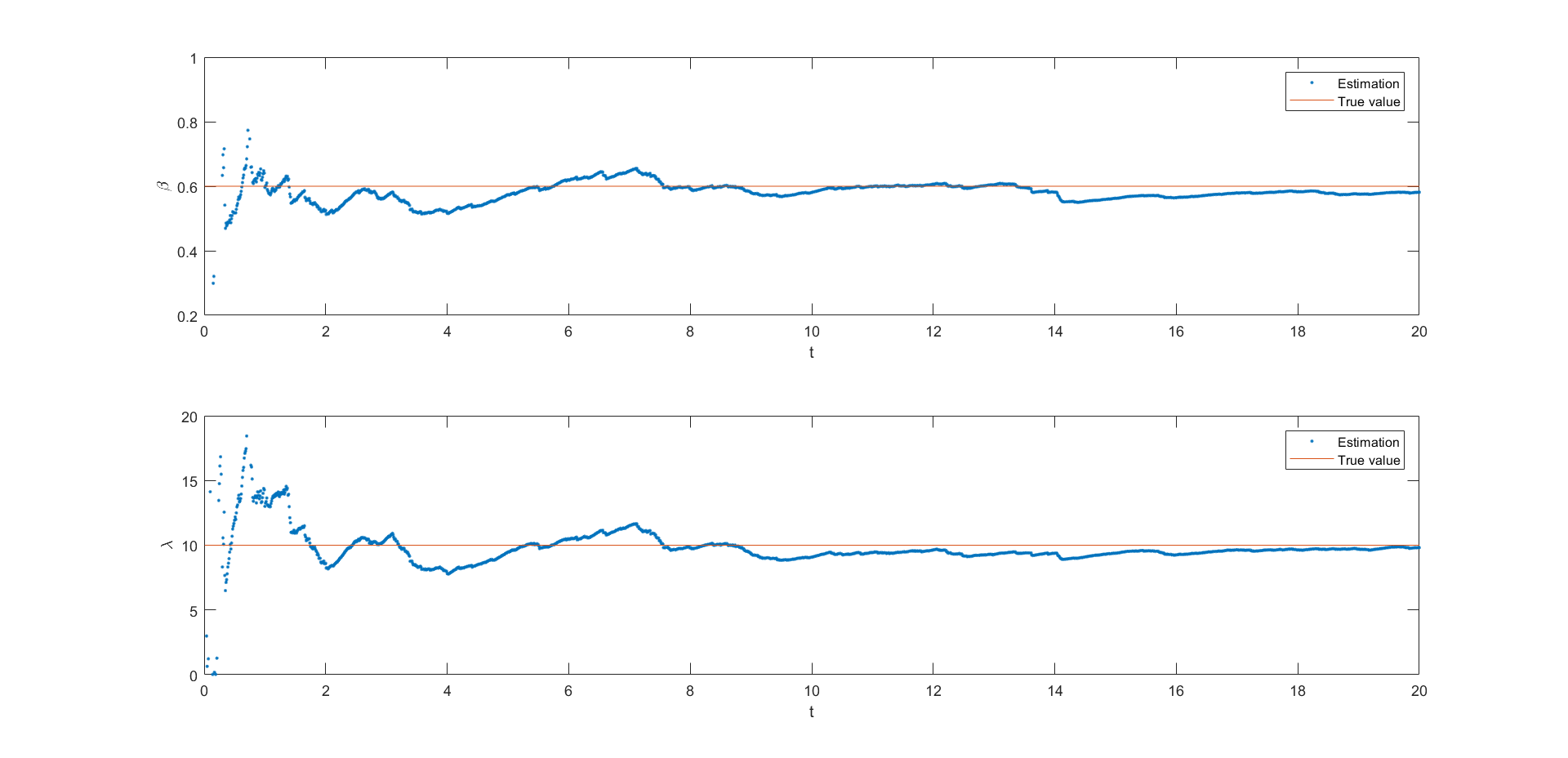
This paper studies a nonlinear filtering problem over an infinite time interval. The signal to be estimated is driven by a stochastic partial differential equation involves unknown parameters. Based on discrete observation, strongly consistent estimators of the parameters are derived at first. With the optimal filter given by Bayes formula, the uniqueness of invariant measure for the signal-filter pair has been verified. The paper then establishes approximation to the optimal filter, showing that the pathwise average distance, per unit time, of the computed approximating filter from the optimal filter converges to zero in probability. Simulation results are presented at last.
翻译:本文研究的是非线性过滤问题的无限时间间隔。 要估计的信号是由随机偏差部分方程式驱动的, 包含未知的参数。 根据离散观测, 开始得出强烈一致的参数估计器。 有了贝斯公式给出的最佳过滤器, 信号过滤器对面的异性测量特性已经得到验证。 本文随后将近似定位到最佳过滤器, 显示从最佳过滤器计算出的近似过滤器的路径平均距离, 以单位时间为单位时间为单位, 概率为零。 模拟结果最终被显示 。