A Decorrelating and Debiasing Approach to Simultaneous Inference for High-Dimensional Confounded Models

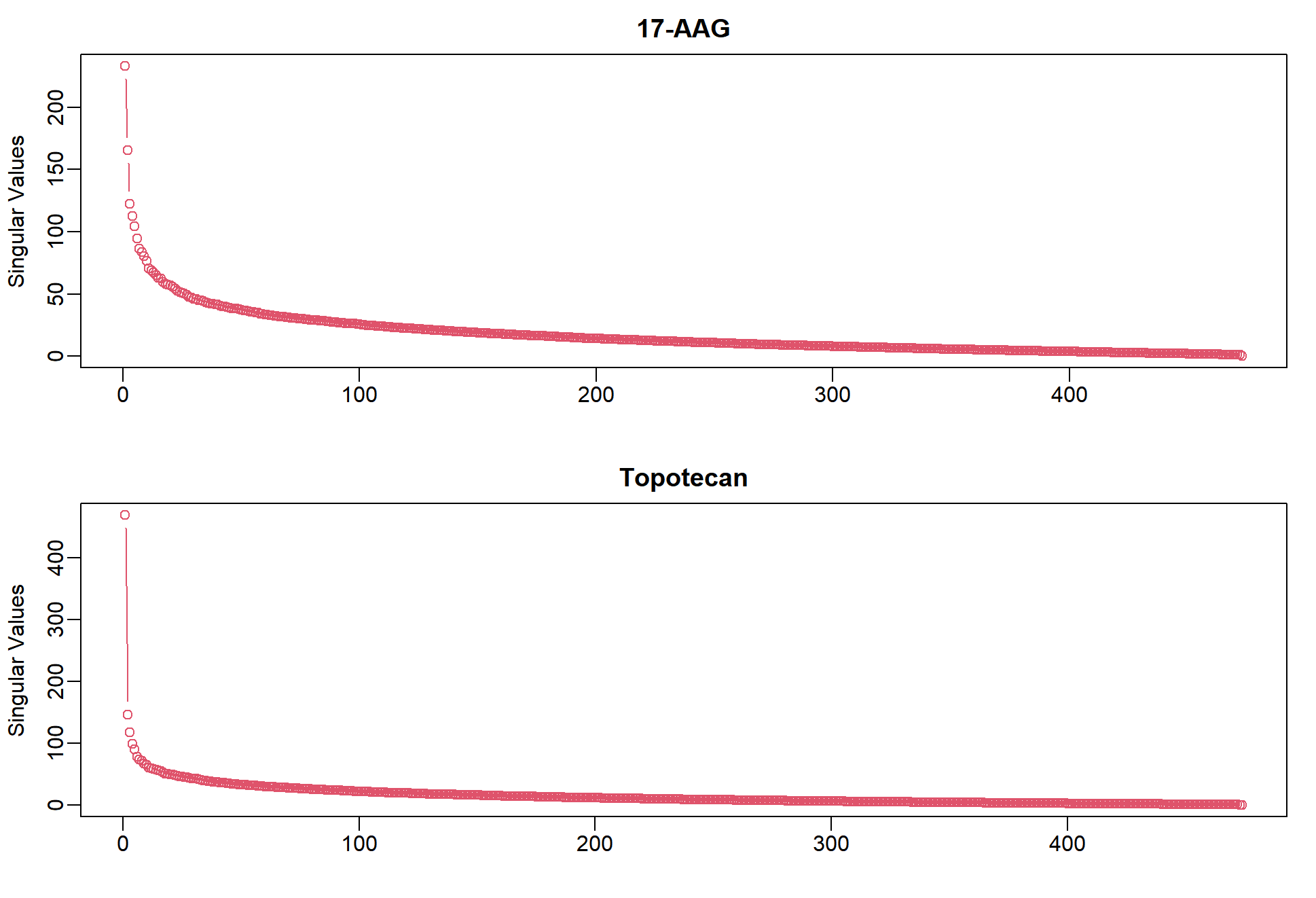
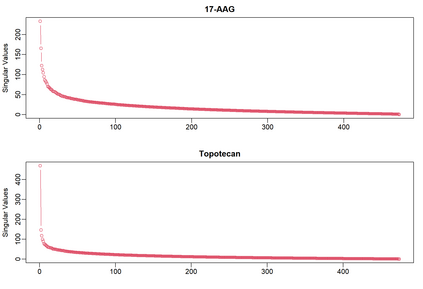
Motivated by the simultaneous association analysis with the presence of latent confounders, this paper studies the large-scale hypothesis testing problem for the high-dimensional confounded linear models with both non-asymptotic and asymptotic false discovery control. Such model covers a wide range of practical settings where both the response and the predictors may be confounded. In the presence of the high-dimensional predictors and the unobservable confounders, the simultaneous inference with provable guarantees becomes highly challenging, and the unknown strong dependency among the confounded covariates makes the challenge even more pronounced. This paper first introduces a decorrelating procedure that shrinks the confounding effect and weakens the correlations among the predictors, then performs debiasing under the decorrelated design based on some biased initial estimator. Standardized test statistics are then constructed and the corresponding asymptotic normality property is established. Furthermore, a simultaneous inference procedure is proposed to identify significant associations, and both the finite-sample and asymptotic false discovery bounds are provided. The non-asymptotic result is general and model-free, and is of independent interest. We also prove that, under minimal signal strength condition, all associations can be successfully detected with probability tending to one. Simulation studies are carried out to evaluate the performance of the proposed approach and compare it with other competing methods. The proposed procedure is further applied to detect the gene associations with the anti-cancer drug sensitivities.