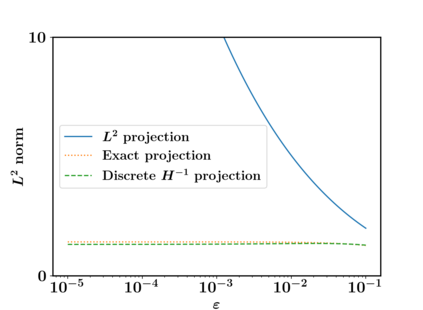
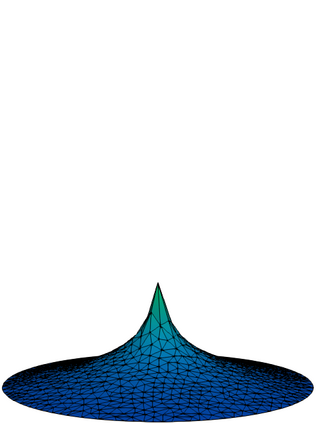
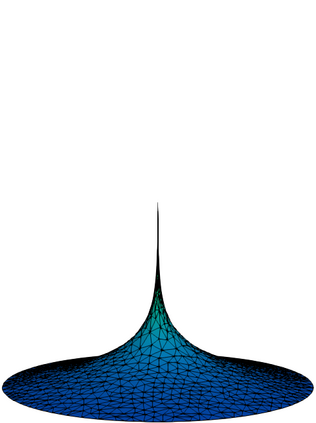
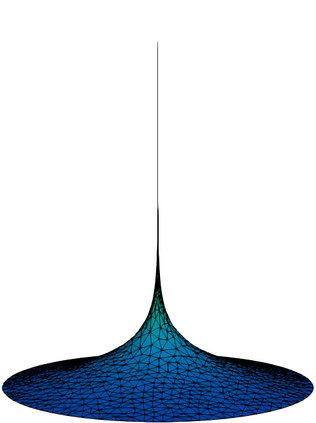
In order to construct regularizations of continuous linear functionals acting on Sobolev spaces such as $W_0^{1,q}(\Omega)$, where $1<q<\infty$ and $\Omega$ is a Lipschitz domain, we propose a projection method in negative Sobolev spaces $W^{-1,p}(\Omega)$, $p$ being the conjugate exponent satisfying $p^{-1} + q^{-1} = 1$. Our method is particularly useful when one is dealing with a rough (irregular) functional that is a member of $W^{-1,p}(\Omega)$, though not of $L^1(\Omega)$, but one strives for a regular approximation in $L^1(\Omega)$. We focus on projections onto discrete finite element spaces $G_n$, and consider both discontinuous as well as continuous piecewise-polynomial approximations. While the proposed method aims to compute the best approximation as measured in the negative (dual) norm, for practical reasons, we will employ a computable, discrete dual norm that supremizes over a discrete subspace $V_m$. We show that this idea leads to a fully discrete method given by a mixed problem on $V_m\times G_n$. We propose a discontinuous as well as a continuous lowest-order pair, prove that they are compatible, and therefore obtain quasi-optimally convergent methods. We present numerical experiments that compute finite element approximations to Dirac delta's and line sources. We also present adaptively generate meshes, obtained from an error representation that comes with the method. Finally, we show how the presented projection method can be used to efficiently compute numerical approximations to partial differential equations with rough data.
翻译:为了在 Sobolev 空间( 如 $W_ 0 ⁇ 1,q} (\ OMEGA) 上建立连续线性功能的正规化, 例如 $W_ 0 ⁇ 1,q}( OMEGA) 美元, $1<q ⁇ infty$ 和$\ OMEGA$ 是一个 Lipschitz 域, 我们提议了一个在负 Sobolev 空间( $_ 1, p} (\ OMEGA) $, 美元是符合 $p ⁇ 1, 1, q} (\ OMEGA) 的连续线性功能中, 我们的方法特别有用, 是一个粗( 不固定) 的( 不固定的) 平面性功能, 而一个不固定的离心机, 我们用一个不固定的直线性数据方法 显示一个不连续的基数值。