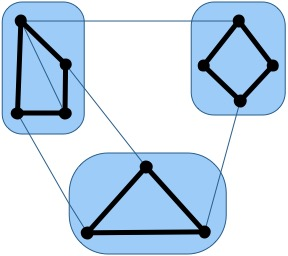
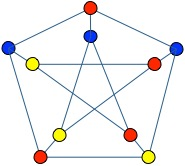
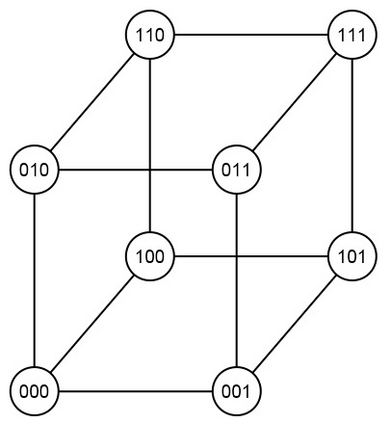
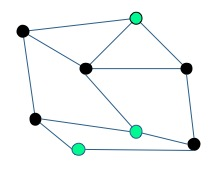
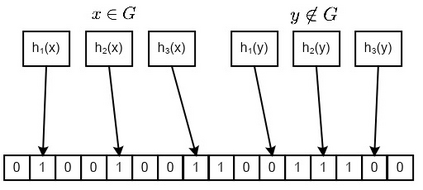
We provide bounds on the compression size of the solutions to 22 problems in computer science. For each problem, we show that solutions exist with high probability, for some simple probability measure. Once this is proven, derandomization can be used to prove the existence of a simple solution.
翻译：我们为计算机科学的22个问题提供了解决方案压缩大小的界限。 对于每一个问题,我们都显示解决方案存在概率很高,以某种简单的概率衡量。 一旦证明这一点,解密可以用来证明存在一个简单的解决方案。
相关内容
Arxiv
0+阅读 · 2022年10月17日
Arxiv
0+阅读 · 2022年10月12日
相关VIP内容
相关资讯
相关论文
Arxiv
0+阅读 · 2022年10月17日
Arxiv
0+阅读 · 2022年10月12日
微信扫码咨询专知VIP会员