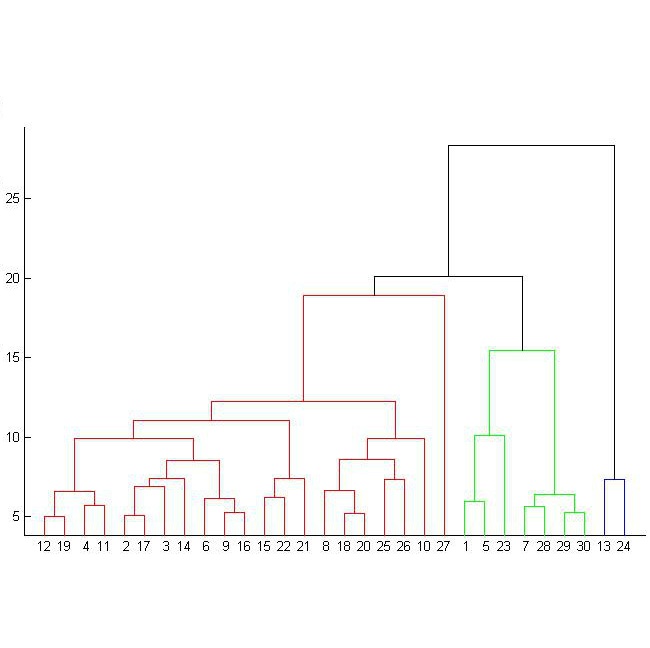
Clustering of mixed-type datasets can be a particularly challenging task as it requires taking into account the associations between variables with different level of measurement, i.e., nominal, ordinal and/or interval. In some cases, hierarchical clustering is considered a suitable approach, as it makes few assumptions about the data and its solution can be easily visualized. Since most hierarchical clustering approaches assume variables are measured on the same scale, a simple strategy for clustering mixed-type data is to homogenize the variables before clustering. This would mean either recoding the continuous variables as categorical ones or vice versa. However, typical discretization of continuous variables implies loss of information. In this work, an agglomerative hierarchical clustering approach for mixed-type data is proposed, which relies on a barycentric coding of continuous variables. The proposed approach minimizes information loss and is compatible with the framework of correspondence analysis. The utility of the method is demonstrated on real and simulated data.
翻译:混合类型数据集的分组可能是一项特别艰巨的任务,因为它需要考虑到具有不同测量水平的变量之间的关联,即名义、正弦和/或间隔。在某些情况下,等级分组被视为一种适当的办法,因为它对数据及其解决办法的假设很少,而且很容易想象到。由于大多数等级分组办法假定变量是在同一尺度上测量的,因此混合类型数据的组合简单战略是在组合之前将变量同质化。这意味着要么将连续变量重新编码为绝对变量,要么反之亦然。然而,连续变量的典型离散意味着信息损失。在这项工作中,提议对混合类型数据采用集中等级分组办法,这种办法依赖于连续变量的中心编码。拟议办法最大限度地减少信息损失,并与通信分析框架相兼容。该方法的实用性在真实和模拟数据上得到证明。