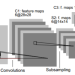
Polyphonic Piano Transcription has recently experienced substantial progress, driven by the use of sophisticated Deep Learning approaches and the introduction of new subtasks such as note onset, offset, velocity and pedal detection. This progress was coupled with an increased complexity and size of the proposed models, typically relying on non-realtime components and high-resolution data. In this work we focus on onset and velocity detection, showing that a substantially smaller and simpler convolutional approach, using lower temporal resolution (24ms), is still competitive: our proposed ONSETS&VELOCITIES model achieves state-of-the-art performance on the MAESTRO dataset for onset detection (F1=96.78%) and sets a good novel baseline for onset+velocity (F1=94.50%), while having ~3.1M parameters and maintaining real-time capabilities on modest commodity hardware. We provide open-source code to reproduce our results and a real-time demo with a pretrained model.
翻译:暂无翻译