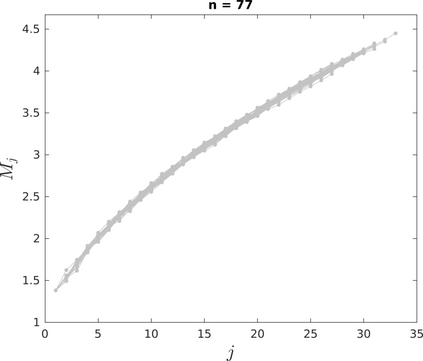
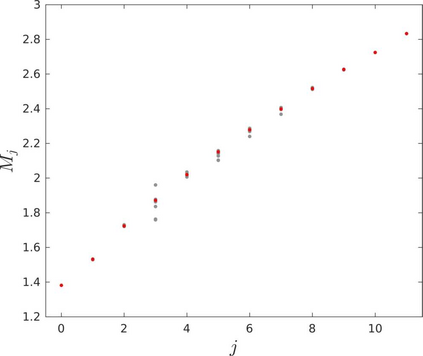
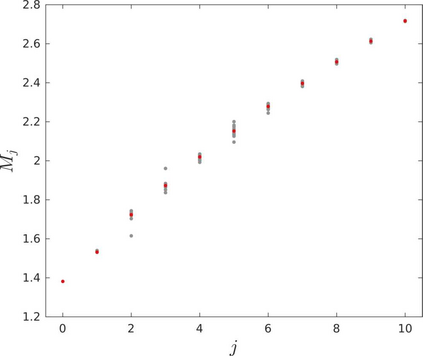
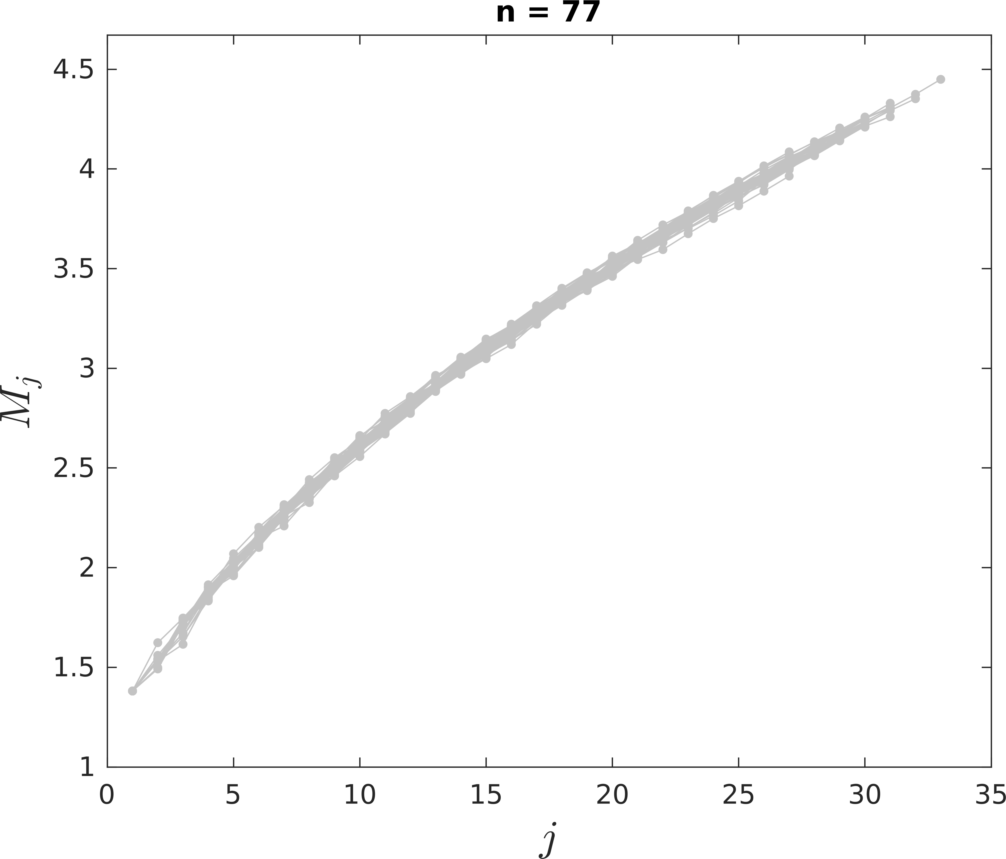
For $\beta > 1$ a real algebraic integer ({\it the base}), the finite alphabets $\mathcal{A} \subset \mathbb{Z}$ which realize the identity $\mathbb{Q}(\beta) = {\rm Per}_{\mathcal{A}}(\beta)$, where ${\rm Per}_{\mathcal{A}}(\beta)$ is the set of complex numbers which are $(\beta, \mathcal{A})$-eventually periodic representations, are investigated. Comparing with the greedy algorithm, minimal and maximal alphabets are defined. The maximal alphabets are shown to be correlated to the asymptotics of the Pierce numbers of the base $\beta$ and Lehmer's problem. The notion of rewriting trail is introduced to construct intermediate alphabets associated with small polynomial values of the base. Consequences on the representations of neighbourhoods of the origin in $\mathbb{Q}(\beta)$, generalizing Schmidt's theorem related to Pisot numbers, are investigated. Applications to Galois conjugation are given for convergent sequences of bases $\gamma_s := \gamma_{n, m_1 , \ldots , m_s}$ such that $\gamma_{s}^{-1}$ is the unique root in $(0,1)$ of an almost Newman polynomial of the type $-1+x+x^n +x^{m_1}+\ldots+ x^{m_s}$, $n \geq 3$, $s \geq 1$, $m_1 - n \geq n-1$, $m_{q+1}-m_q \geq n-1$ for all $q \geq 1$. For $\beta > 1$ a reciprocal algebraic integer close to one, the poles of modulus $< 1$ of the dynamical zeta function of the $\beta$-shift $\zeta_{\beta}(z)$ are shown, under some assumptions, to be zeroes of the minimal polynomial of $\beta$.
翻译:对于 $\ beta > 1美元 一个真实的代数整数( 基数 ), 调查固定的字母 $\ mathcal{A}\ subset\ mathb} 美元, 使身份 $\ mathb{A\\ (\ beta) =\ prm Per\ mathcal{A\\\ (\beta) 美元, $\ 美元, 美元, 美元, 美元, 美元, 美元, 美元, 美元, 美元, 美元, 美元, 固定的字母与贪婪的算法, 最小的和最高值 。 最大值与基数 $\ qetax 和莱赫默尔的印数值有关系。 重写线索的概念是构建与基数小的中间字母, 美元- == * 美元, 美元源的直径的表示结果是 美元, 美元, 普通的Schem_ ligx 应用到 Pial_ 。
相关内容
Alphabet is mostly a collection of companies. This newer Google is a bit slimmed down, with the companies that are pretty far afield of our main internet products contained in Alphabet instead.https://abc.xyz/