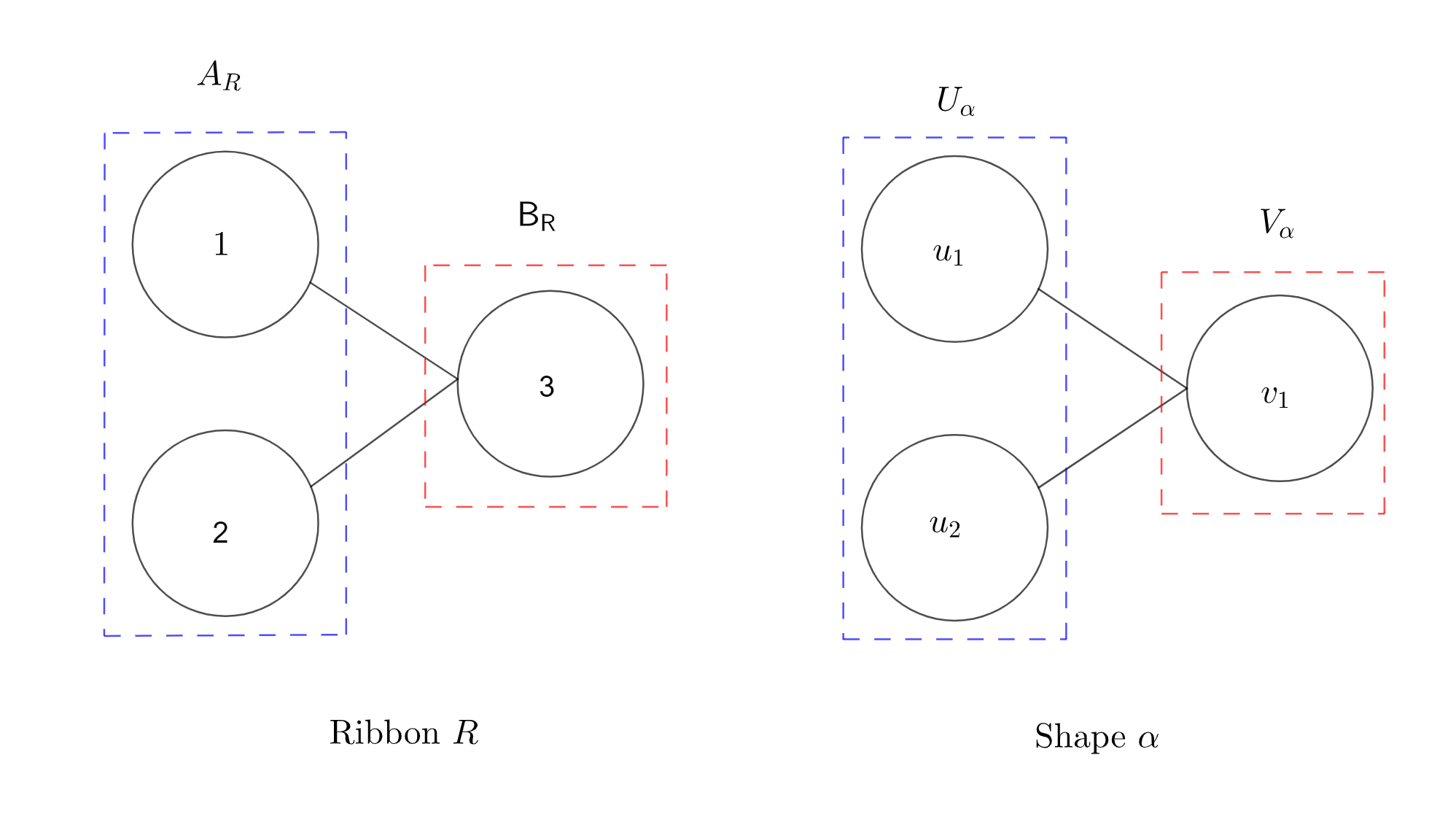
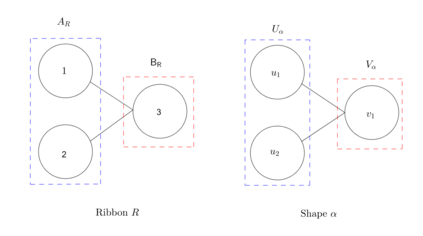
The Sum-of-Squares (SoS) hierarchy of semidefinite programs is a powerful algorithmic paradigm which captures state-of-the-art algorithmic guarantees for a wide array of problems. In the average case setting, SoS lower bounds provide strong evidence of algorithmic hardness or information-computation gaps. Prior to this work, SoS lower bounds have been obtained for problems in the "dense" input regime, where the input is a collection of independent Rademacher or Gaussian random variables, while the sparse regime has remained out of reach. We make the first progress in this direction by obtaining strong SoS lower bounds for the problem of Independent Set on sparse random graphs. We prove that with high probability over an Erdos-Renyi random graph $G\sim G_{n,\frac{d}{n}}$ with average degree $d>\log^2 n$, degree-$D_{SoS}$ SoS fails to refute the existence of an independent set of size $k = \Omega\left(\frac{n}{\sqrt{d}(\log n)(D_{SoS})^{c_0}} \right)$ in $G$ (where $c_0$ is an absolute constant), whereas the true size of the largest independent set in $G$ is $O\left(\frac{n\log d}{d}\right)$. Our proof involves several significant extensions of the techniques used for proving SoS lower bounds in the dense setting. Previous lower bounds are based on the pseudo-calibration heuristic of Barak et al [FOCS 2016] which produces a candidate SoS solution using a planted distribution indistinguishable from the input distribution via low-degree tests. In the sparse case the natural planted distribution does admit low-degree distinguishers, and we show how to adapt the pseudo-calibration heuristic to overcome this. Another notorious technical challenge for the sparse regime is the quest for matrix norm bounds. In this paper, we obtain new norm bounds for graph matrices in the sparse setting.
翻译:半确定性程序 Sum- square (SoS) 的半确定性等级是一个强大的算法范式, 它可以捕捉到对一系列广泛问题的最先进的算法保障。 在平均案例设置中, SoS 的下界提供了强力的算法硬性或信息算法差距的证据。 在此之前, 已经为“ 敏感” 输入系统中的问题获取了较低的界限, 输入是独立的 Rademacher 或 Gausian 随机变量的集合, 而稀薄的制度仍然无法达到。 我们在这方面取得了第一个进展, 通过在稀释的随机图表中为独立集问题获取强大的 SoSS descretarial 范围。 我们证明, Erdos- Renyiotal 图形 $Gsim G ⁇ n,\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\