Estimation of Multivariate Discrete Hawkes Processes: An Application to Incident Monitoring

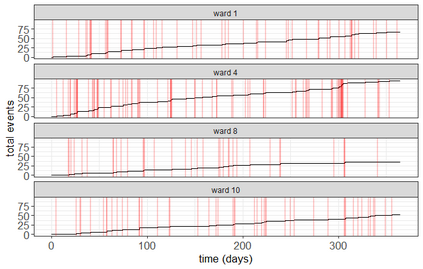
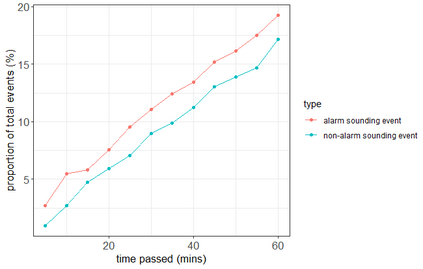
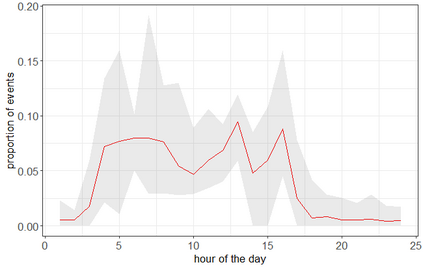
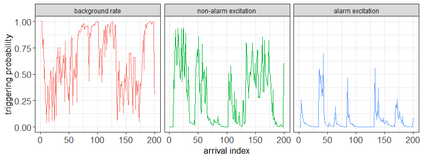
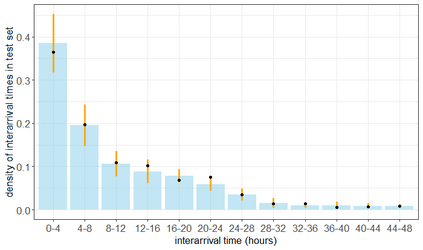
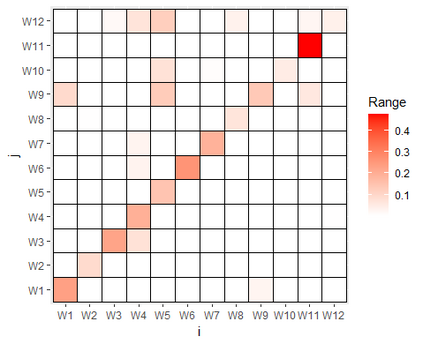
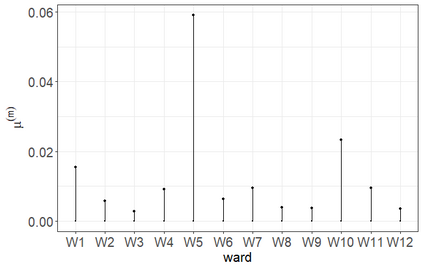
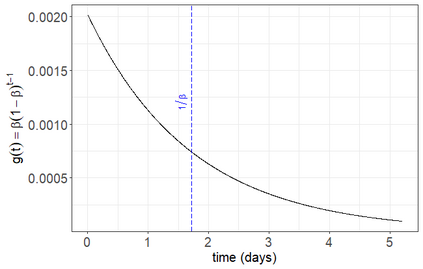
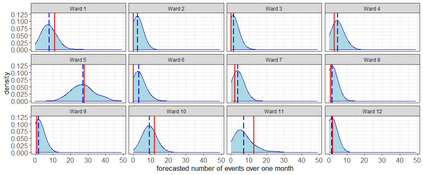
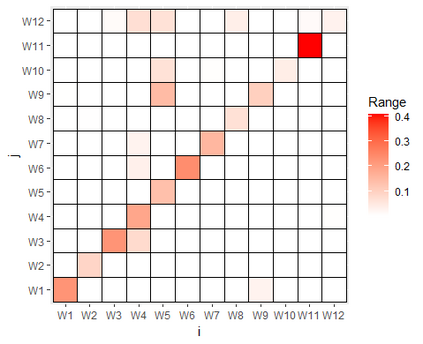
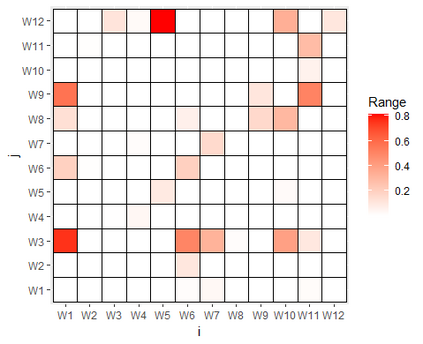
Hawkes processes are a class of self-exciting point processes that are used to model complex phenomena. While most applications of Hawkes processes assume that event data occurs in continuous-time, the less-studied discrete-time version of the process is more appropriate in some situations. In this work, we develop methodology for the efficient implementation of discrete Hawkes processes. We achieve this by developing efficient algorithms to evaluate the log-likelihood function and its gradient, whose computational complexity is linear in the number of events. We extend these methods to a particular form of a multivariate marked discrete Hawkes process which we use to model the occurrences of violent events within a forensic psychiatric hospital. A prominent feature of our problem, captured by a mark in our process, is the presence of an alarm system which can be heard throughout the hospital. An alarm is sounded when an event is particularly violent in nature and warrants a call for assistance from other members of staff. We conduct a detailed analysis showing that such a variant of the Hawkes process manages to outperform alternative models in terms of predictive power. Finally, we interpret our findings and describe their implications.