From $p$-Values to Posterior Probabilities of Hypothesis

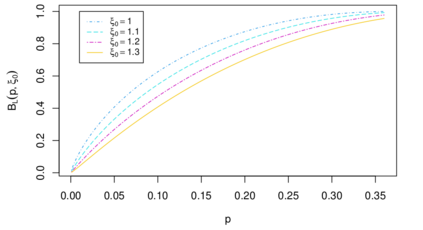
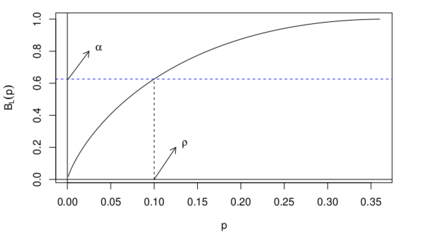
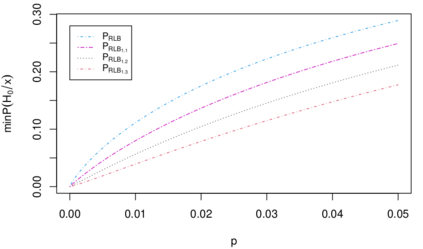
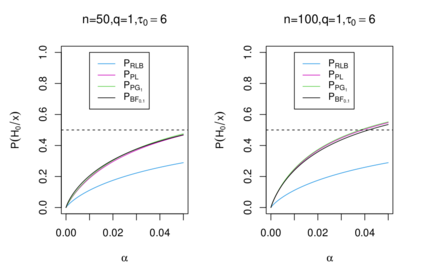
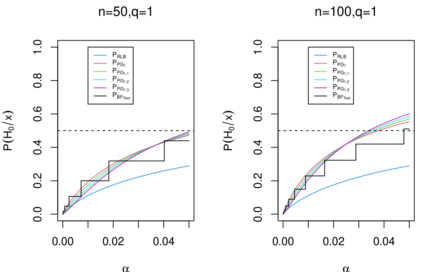
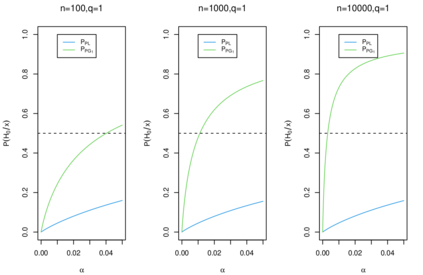
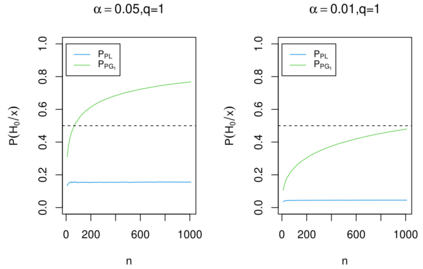
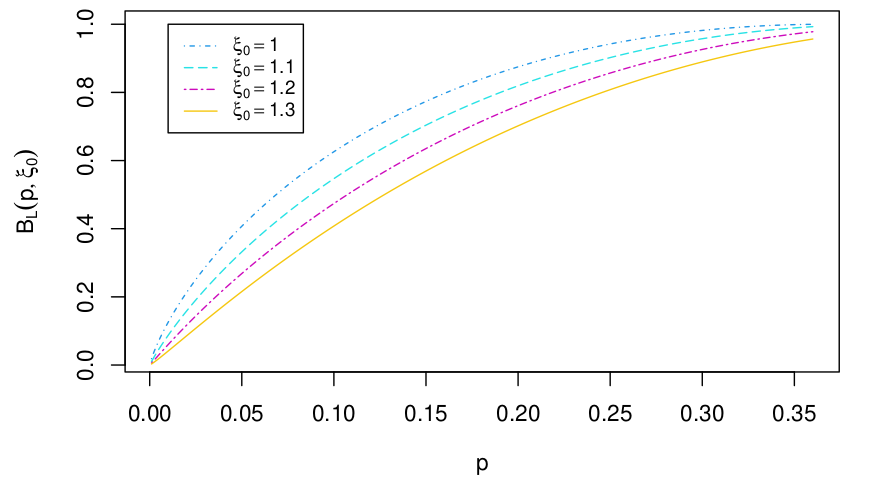
Minimum Bayes factors are commonly used to transform two-sided p-values to lower bounds on the posterior probability of the null hypothesis, as in Pericchi et al. (2017). In this article, we show posterior probabilities of hypothesis by transforming the commonly used -eplog(p), proposed by Vovk (1993) and Sellke et al. (2001). This is achieved after adjusting this minimum Bayes factor with the information to approximate it to an exact Bayes factor, not only when p is a p-value but also when p is a pseudo p-value in the sense of Casella and Berger (2001). Additionally, we show the fit to a refined version to linear models.