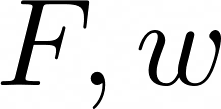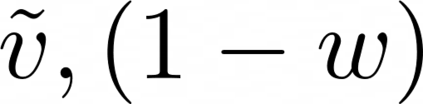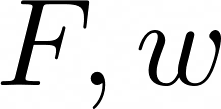WeightedPose: Generalizable Cross-Pose Estimation via Weighted SVD

We introduce a new approach for robotic manipulation tasks in human settings that necessitates understanding the 3D geometric connections between a pair of objects. Conventional end-to-end training approaches, which convert pixel observations directly into robot actions, often fail to effectively understand complex pose rela- tionships and do not easily adapt to new object configurations. To overcome these issues, our method focuses on learning the 3D geometric relationships, particularly how critical parts of one object relate to those of another. We employ Weighted SVD in our standalone model to analyze pose relationships both in articulated parts and in free-floating objects. For instance, our model can comprehend the spatial relationship between an oven door and the oven body, as well as between a lasagna plate and the oven. By concentrating on the 3D geometric connections, our strategy empowers robots to carry out intricate manipulation tasks based on object-centric perspectives