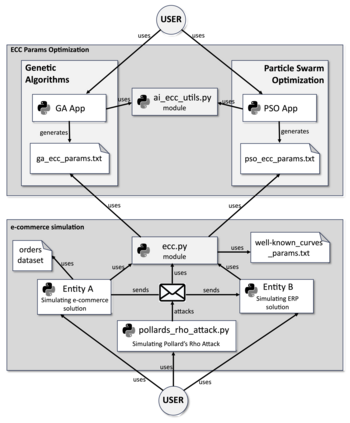
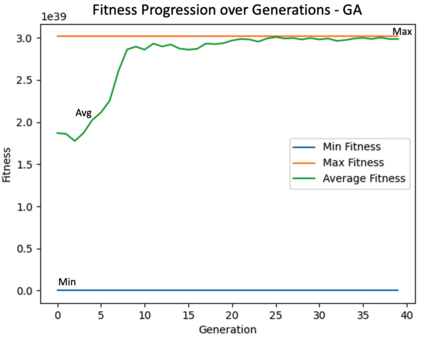
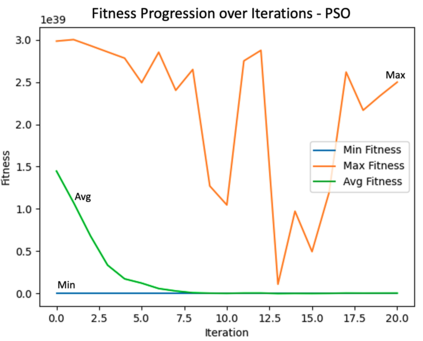
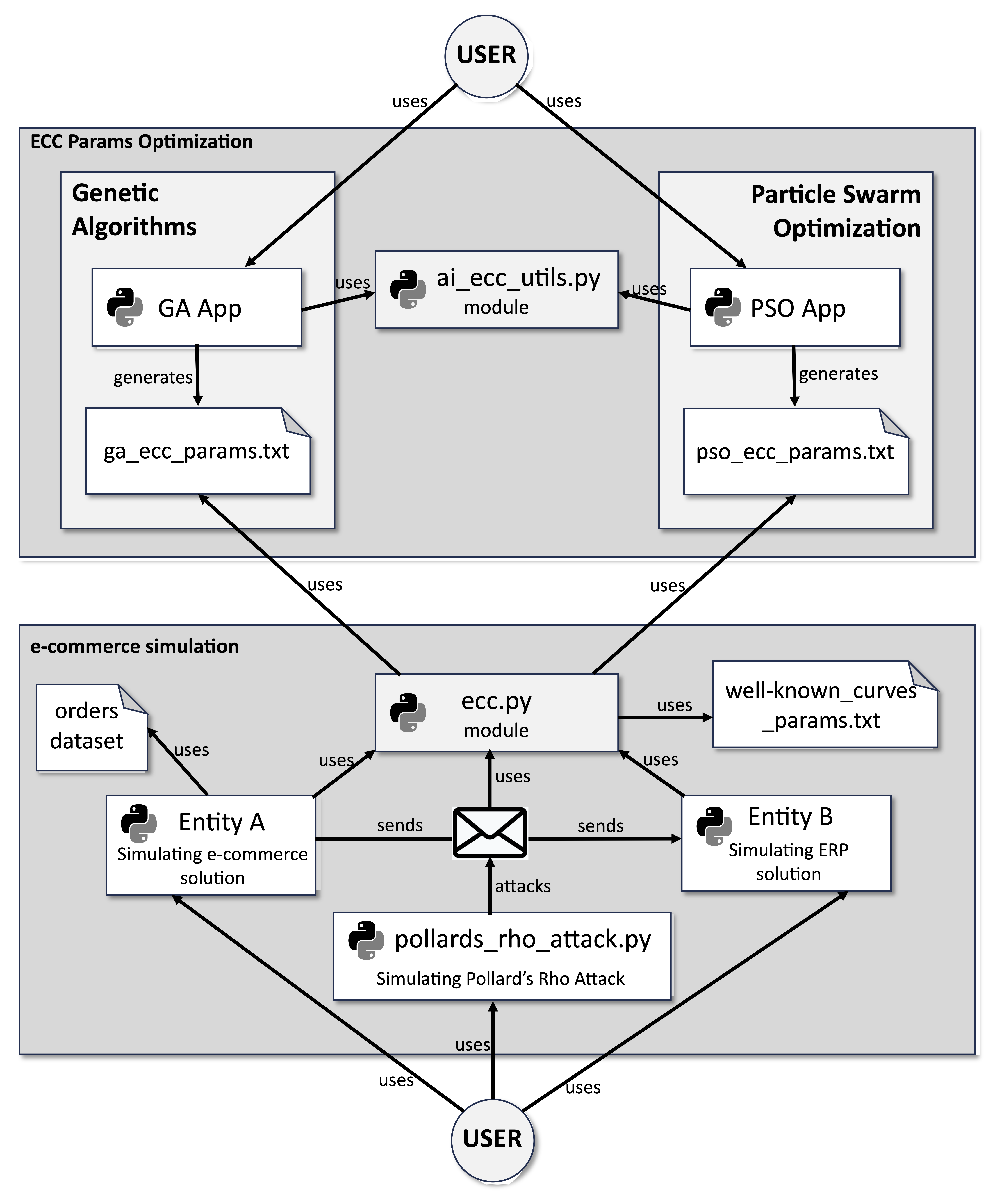
This paper presents a comparative analysis between the Genetic Algorithm (GA) and Particle Swarm Optimization (PSO), two vital artificial intelligence algorithms, focusing on optimizing Elliptic Curve Cryptography (ECC) parameters. These encompass the elliptic curve coefficients, prime number, generator point, group order, and cofactor. The study provides insights into which of the bio-inspired algorithms yields better optimization results for ECC configurations, examining performances under the same fitness function. This function incorporates methods to ensure robust ECC parameters, including assessing for singular or anomalous curves and applying Pollard's rho attack and Hasse's theorem for optimization precision. The optimized parameters generated by GA and PSO are tested in a simulated e-commerce environment, contrasting with well-known curves like secp256k1 during the transmission of order messages using Elliptic Curve-Diffie Hellman (ECDH) and Hash-based Message Authentication Code (HMAC). Focusing on traditional computing in the pre-quantum era, this research highlights the efficacy of GA and PSO in ECC optimization, with implications for enhancing cybersecurity in third-party e-commerce integrations. We recommend the immediate consideration of these findings before quantum computing's widespread adoption.
翻译:暂无翻译