Star-Graph Multimodal Matching Component Analysis for Data Fusion and Transfer Learning

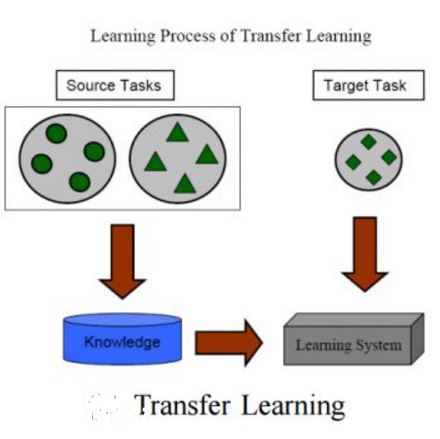
Previous matching component analysis (MCA) techniques map two data domains to a common domain for further processing in data fusion and transfer learning contexts. In this paper, we extend these techniques to the star-graph multimodal (SGM) case in which one particular data domain is connected to $m$ others via an objective function. We provide a particular feasible point for the resulting trace maximization problem in closed form and algorithms for its computation and iterative improvement, leading to our main result, the SGM maps. We also provide numerical examples demonstrating that SGM is capable of encoding into its maps more information than MCA when few training points are available. In addition, we develop a further generalization of the MCA covariance constraint, eliminating a previous feasibility condition and allowing larger values of the rank of the prescribed covariance matrix.