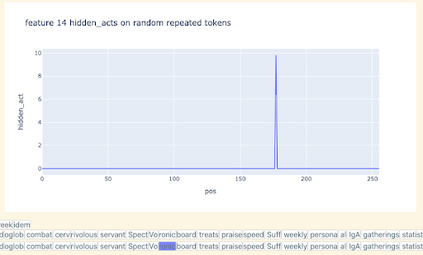
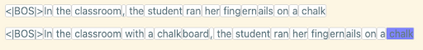
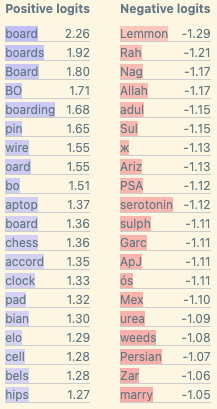
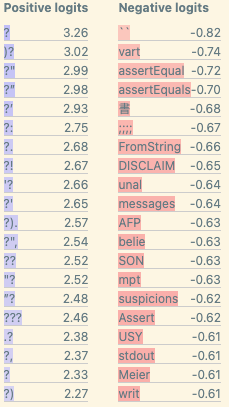
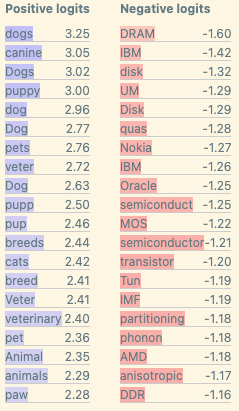
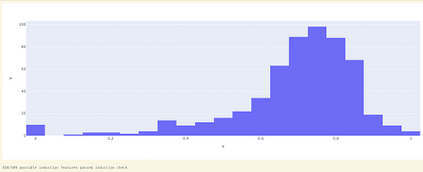
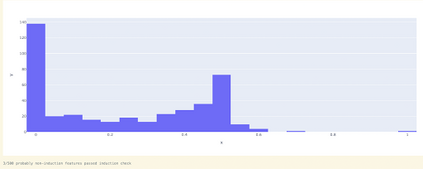
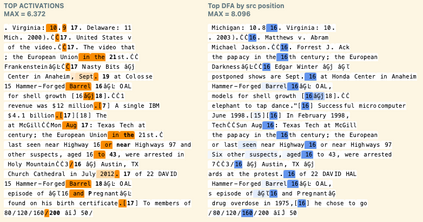
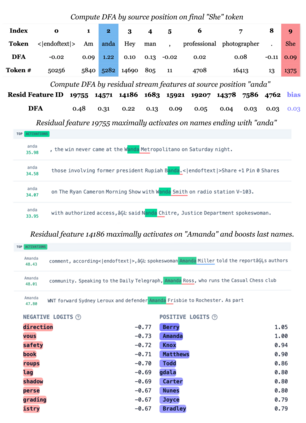
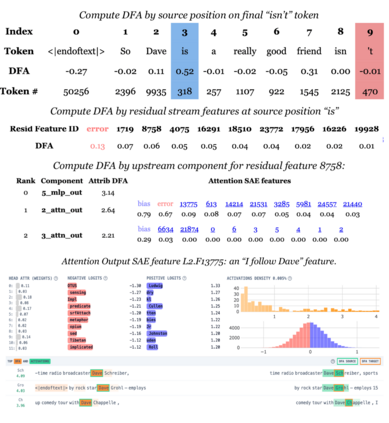
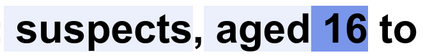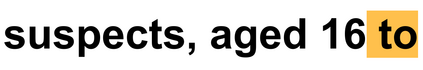Decomposing model activations into interpretable components is a key open problem in mechanistic interpretability. Sparse autoencoders (SAEs) are a popular method for decomposing the internal activations of trained transformers into sparse, interpretable features, and have been applied to MLP layers and the residual stream. In this work we train SAEs on attention layer outputs and show that also here SAEs find a sparse, interpretable decomposition. We demonstrate this on transformers from several model families and up to 2B parameters. We perform a qualitative study of the features computed by attention layers, and find multiple families: long-range context, short-range context and induction features. We qualitatively study the role of every head in GPT-2 Small, and estimate that at least 90% of the heads are polysemantic, i.e. have multiple unrelated roles. Further, we show that Sparse Autoencoders are a useful tool that enable researchers to explain model behavior in greater detail than prior work. For example, we explore the mystery of why models have so many seemingly redundant induction heads, use SAEs to motivate the hypothesis that some are long-prefix whereas others are short-prefix, and confirm this with more rigorous analysis. We use our SAEs to analyze the computation performed by the Indirect Object Identification circuit (Wang et al.), validating that the SAEs find causally meaningful intermediate variables, and deepening our understanding of the semantics of the circuit. We open-source the trained SAEs and a tool for exploring arbitrary prompts through the lens of Attention Output SAEs.
翻译:暂无翻译