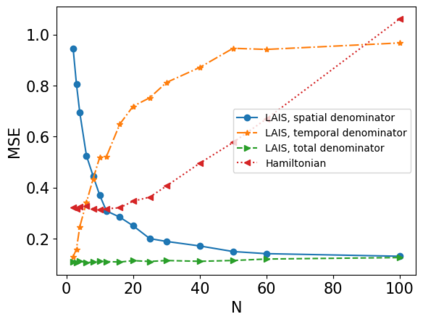
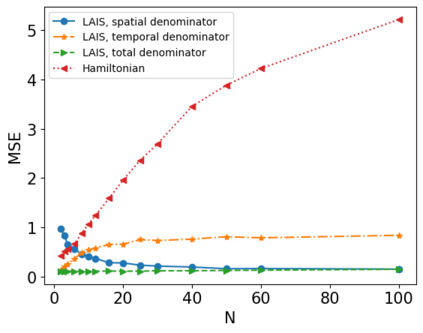
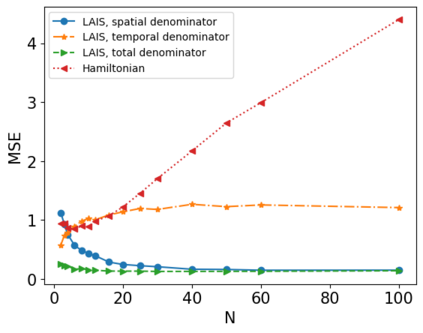
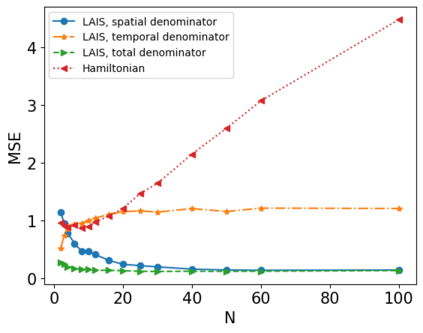
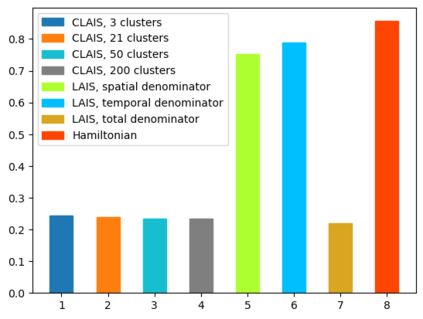
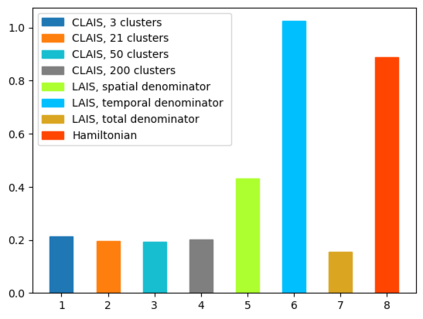
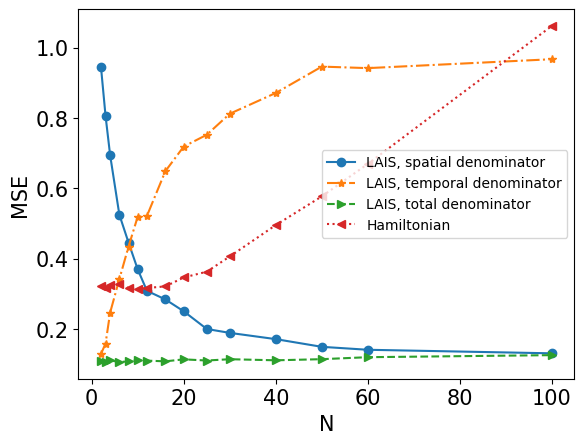
Monte Carlo sampling methods are the standard procedure for approximating complicated integrals of multidimensional posterior distributions in Bayesian inference. In this work, we focus on the class of Layered Adaptive Importance Sampling (LAIS) scheme, which is a family of adaptive importance samplers where Markov chain Monte Carlo algorithms are employed to drive an underlying multiple importance sampling scheme. The modular nature of LAIS allows for different possible implementations, yielding a variety of different performance and computational costs. In this work, we propose different enhancements of the classical LAIS setting in order to increase the efficiency and reduce the computational cost, of both upper and lower layers. The different variants address computational challenges arising in real-world applications, for instance with highly concentrated posterior distributions. Furthermore, we introduce different strategies for designing cheaper schemes, for instance, recycling samples generated in the upper layer and using them in the final estimators in the lower layer. Different numerical experiments, considering several challenging scenarios, show the benefits of the proposed schemes comparing with benchmark methods presented in the literature.
翻译:蒙特卡洛采样方法是贝叶西亚多维次子分布分布的复杂组成部分的标准程序。在这项工作中,我们侧重于多层适应重要性取样(LAIS)方案(LAIS),这是一个适应性重要取样器组,使用Markov连锁蒙特卡洛算法推动一个基本的多重重要取样方案。LAIS的模块性质允许不同可能的实施,产生各种不同的性能和计算成本。在这项工作中,我们建议对古典LAIS设置进行不同的改进,以提高上层和下层的效率并降低计算成本。不同的变式处理现实世界应用中出现的计算挑战,例如高度集中的外层分布。此外,我们引入了不同的战略来设计更便宜的计划,例如回收在上层产生的样品,并在下层的最后估测器中使用这些样品。不同的数字实验,考虑到一些具有挑战性的设想,显示了与文献中提出的基准方法相比较的拟议计划的好处。