A Note on the Computational Complexity of Selfmate and Reflexmate Chess Problems

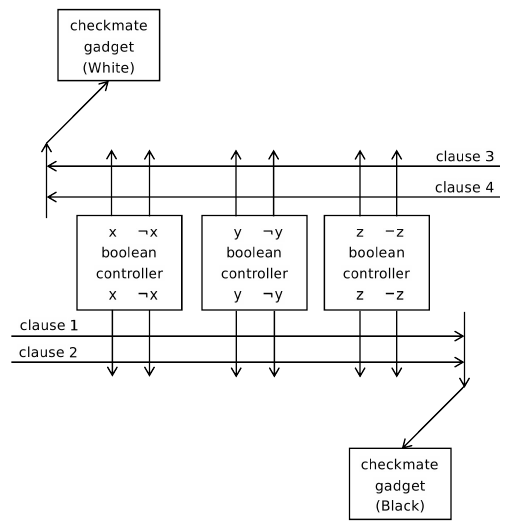
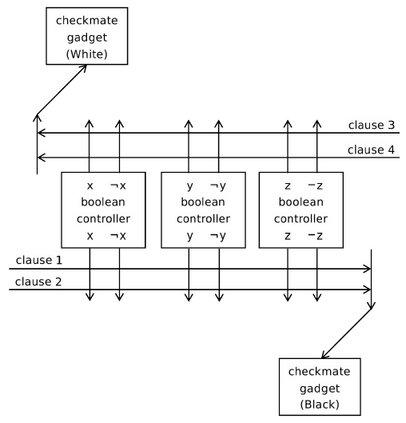
A selfmate is a Chess problem in which White, moving first, needs to force Black to checkmate within a specified number of moves. The reflexmate is a derivative of the selfmate in which White compels Black to checkmate with the added condition that if either player can checkmate, they must do that (when this condition applies only to Black, it is a semi-reflexmate). We slightly modify the reduction of EXPTIME-hardness of Chess and apply the reduction to these Chess problems. It is proved that selfmate, reflexmate, and semi-reflexmate are all EXPTIME-complete.