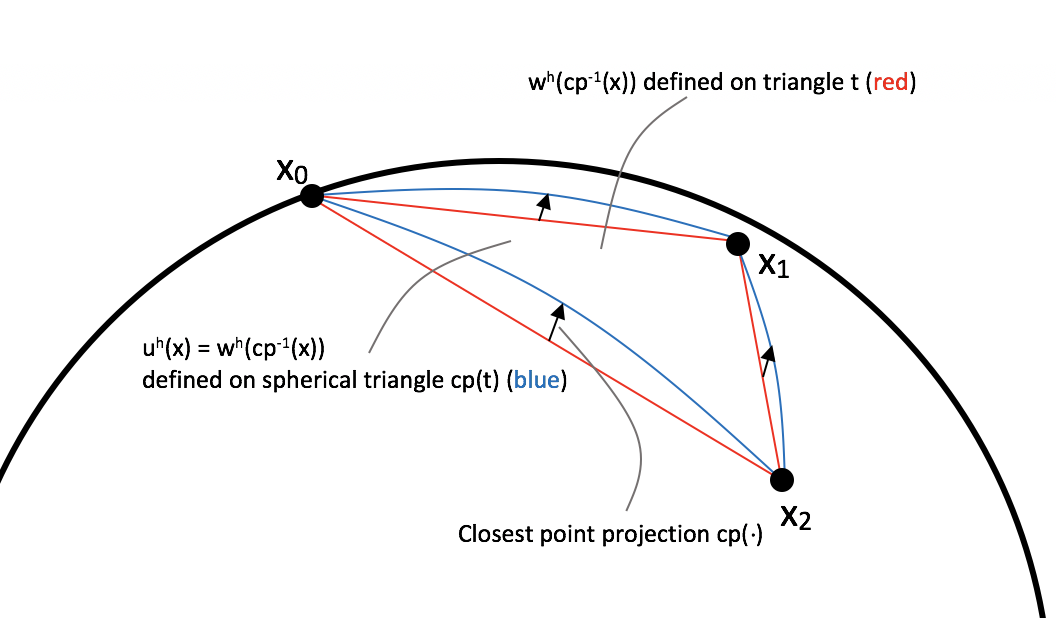
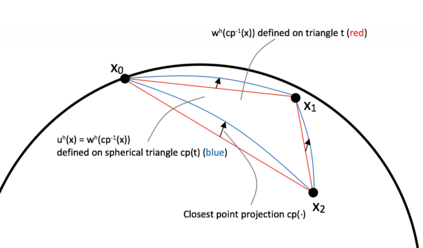
We consider a PDE approach to numerically solving the optimal transportation problem on the sphere. We focus on both the traditional squared geodesic cost and a logarithmic cost, which arises in the reflector antenna design problem. At each point on the sphere, we replace the surface PDE with a generalized Monge-Amp\`ere type equation posed on the tangent plane using normal coordinates. The resulting nonlinear PDE can then be approximated by any consistent, monotone scheme for generalized Monge-Amp\`ere type equations on the plane. Existing techniques for proving convergence do not immediately apply because the PDE lacks both a comparison principle and a unique solution, which makes it difficult to produce a stable, well-posed scheme. By augmenting this discretization with an additional term that constrains the solution gradient, we obtain a strong form of stability. A modification of the Barles-Souganidis convergence framework then establishes convergence to the mean-zero solution of the original PDE.
翻译:我们考虑用PDE方法从数字上解决球体上的最佳运输问题。 我们既关注传统的平方大地测量成本,也关注反射天线设计问题中产生的对数成本。 在球体上的每一点,我们用正常坐标在正切平面上以通用的蒙古-安普-安普-盖尔方程式取代表面PDE。由此产生的非线性PDE可以被飞机上通用蒙古-安普-安培-埃雷方程式的任何一致的单调方案所近似。 现有的证明趋同技术并不立即适用, 因为 PDE既缺乏比较原则,也缺乏独特的解决办法,因此难以产生稳定、良好的方案。 通过增加限制溶性梯度的附加术语,我们获得了一种强大的稳定性。 修改巴列斯-苏格尼迪斯的趋同框架之后, 就可以与原PDE的平均值- 零解决方案取得趋同。