$Γ$-Convergence of an Ambrosio-Tortorelli approximation scheme for image segmentation

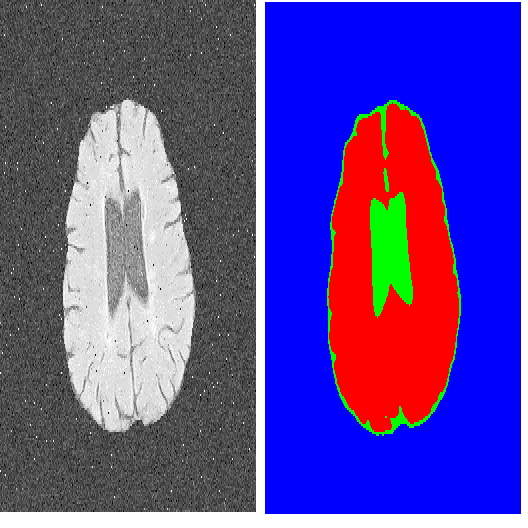
Given an image $u_0$, the aim of minimising the Mumford-Shah functional is to find a decomposition of the image domain into sub-domains and a piecewise smooth approximation $u$ of $u_0$ such that $u$ varies smoothly within each sub-domain. Since the Mumford-Shah functional is highly non-smooth, regularizations such as the Ambrosio-Tortorelli approximation can be considered which is one of the most computationally efficient approximations of the Mumford-Shah functional for image segmentation. Our main result is the $\Gamma$-convergence of the Ambrosio-Tortorelli approximation of the Mumford-Shah functional for piecewise smooth approximations. This requires the introduction of an appropriate function space. As a consequence of our $\Gamma$-convergence result, we can infer the convergence of minimizers of the respective functionals.