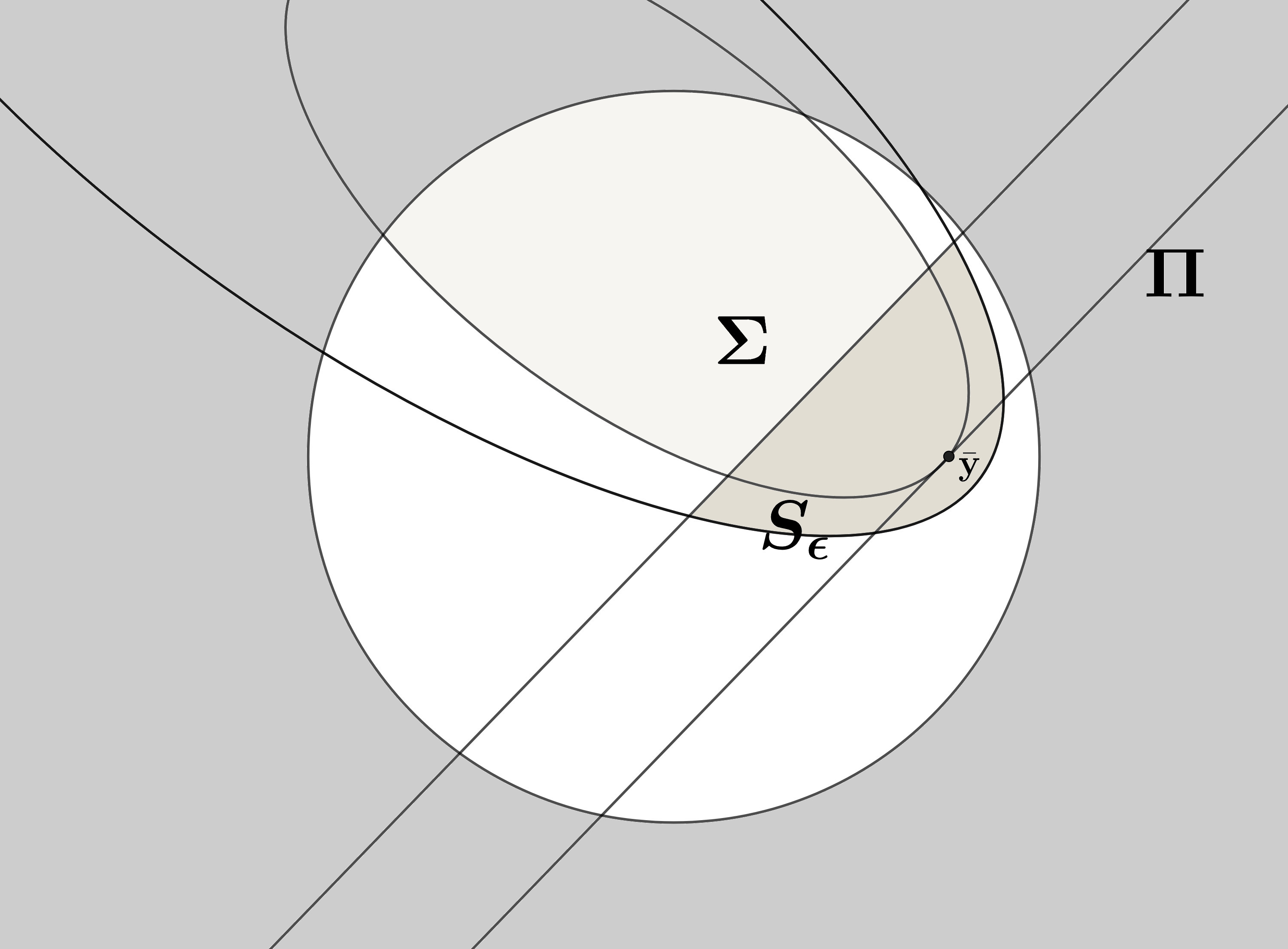
For a probability P in $R^d$ its center outward distribution function $F_{\pm}$, introduced in Chernozhukov et al. (2017) and Hallin et al. (2021), is a new and successful concept of multivariate distribution function based on mass transportation theory. This work proves, for a probability P with density locally bounded away from zero and infinity in its support, the continuity of the center-outward map on the interior of the support of P and the continuity of its inverse, the quantile, $Q_{\pm}$. This relaxes the convexity assumption in del Barrio et al. (2020). Some important consequences of this continuity are Glivenko-Cantelli type theorems and characterisation of weak convergence by the stability of the center-outward map.
翻译:对于概率P在$R^d$中,其中心外分布函数$F_{\pm}$是基于质量传输理论的一种新的成功的多元分布函数概念,由Chernozhukov等人(2017)和Hallin等人(2021)提出。本研究证明了对于具有在其支持中局部有界于零和无穷大的密度的概率P,在P的支持内部中心向外地映射的连续性以及它的逆,即分位数$Q_{\pm}$的连续性。这一结果放松了del Barrio等人(2020)中的凸性假设。这种连续性的一些重要推论是Glivenko-Cantelli定理类型的定理和通过中心向外映射的稳定性对弱收敛的表征。