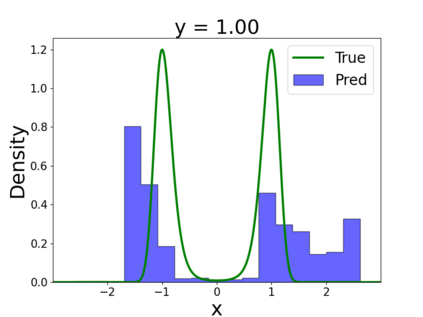
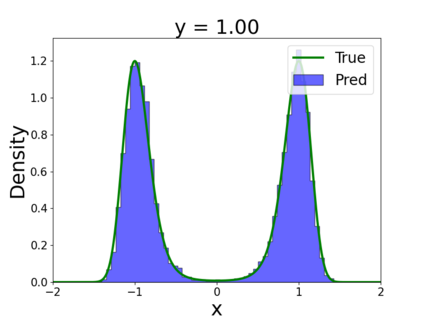
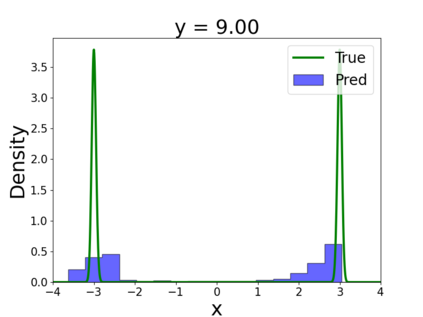
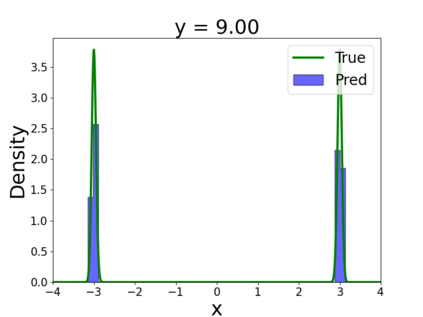
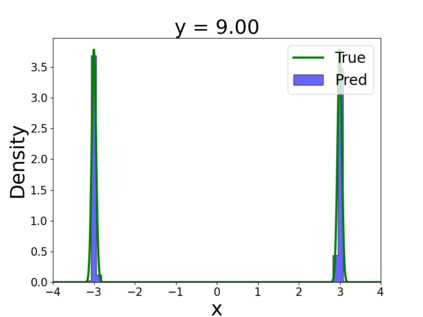
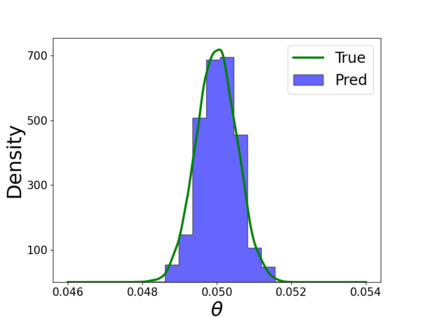
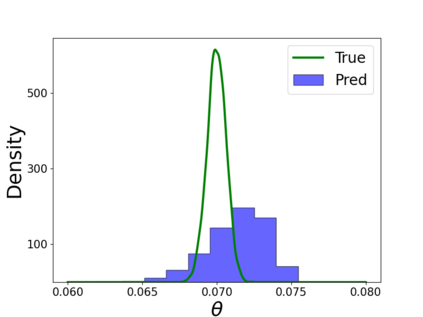
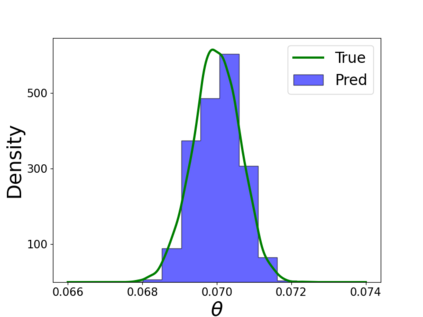
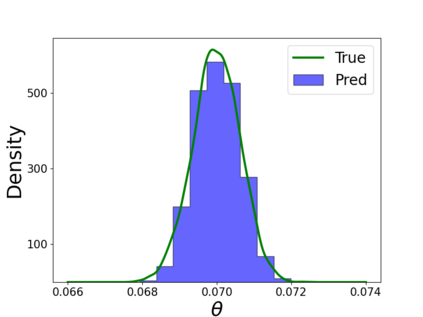
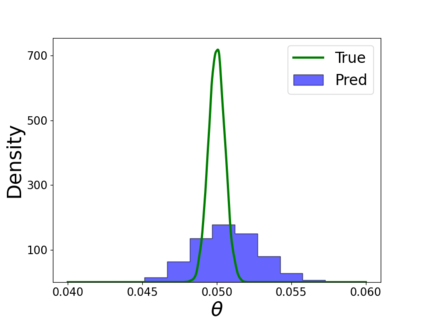
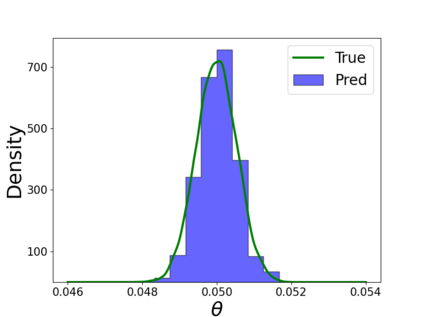
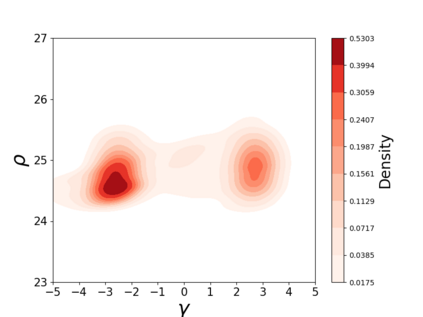
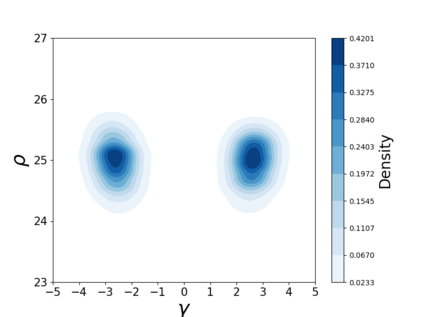
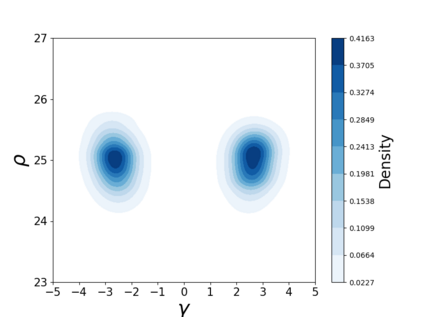
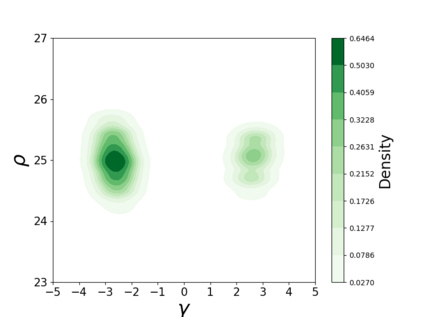
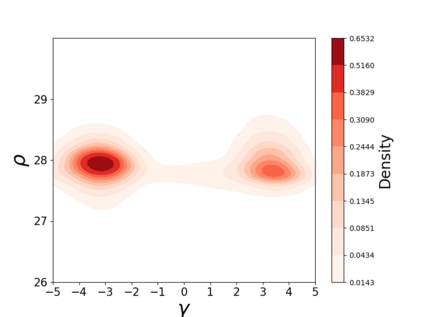
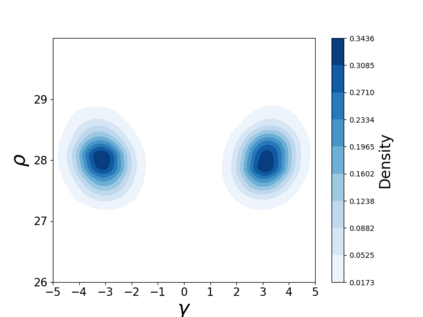
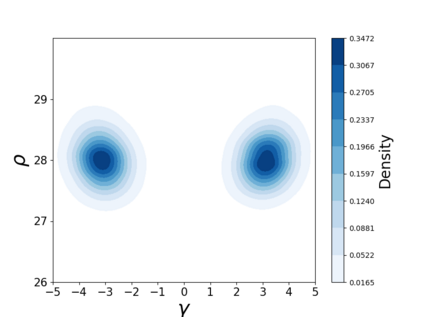
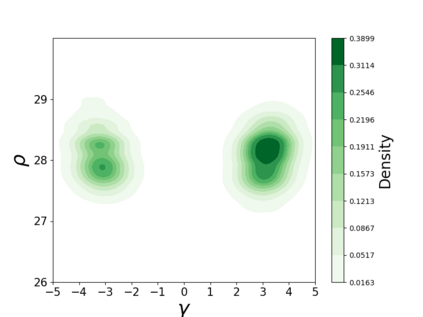
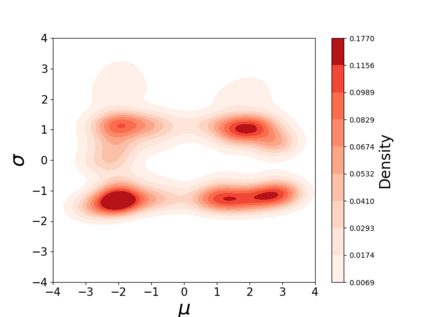
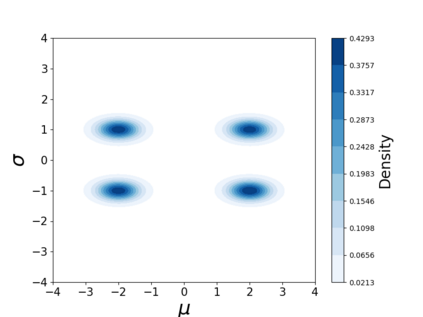
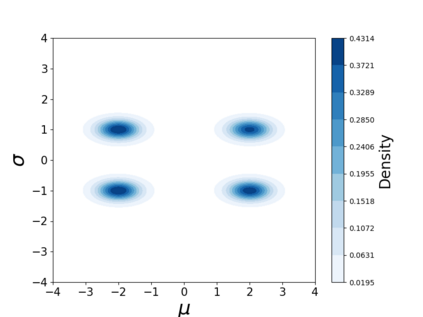
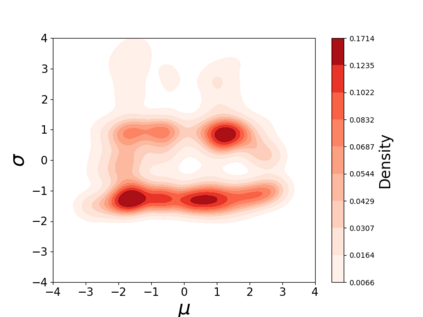
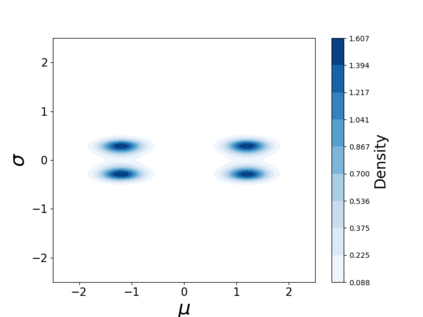
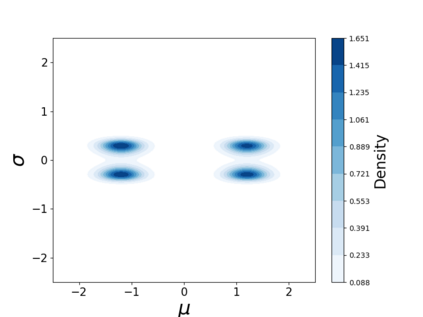
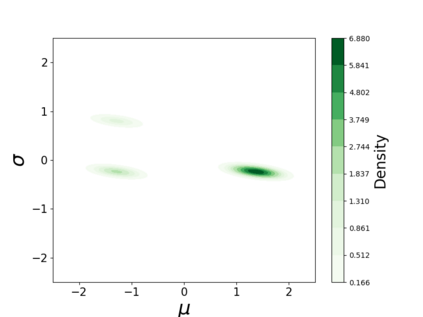
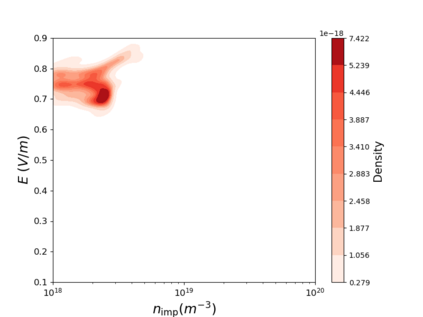
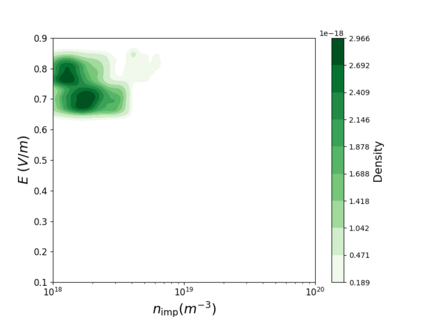
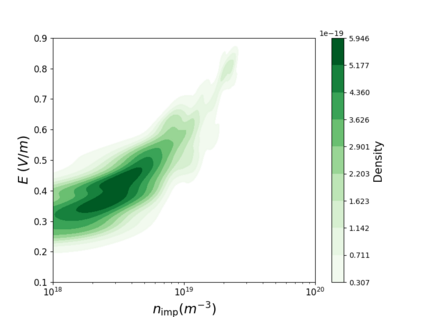
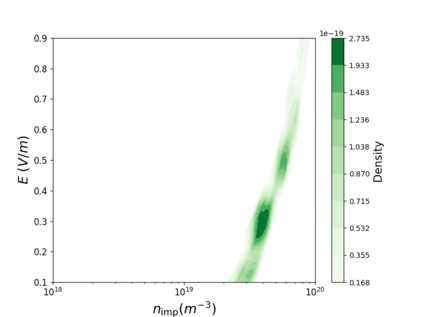
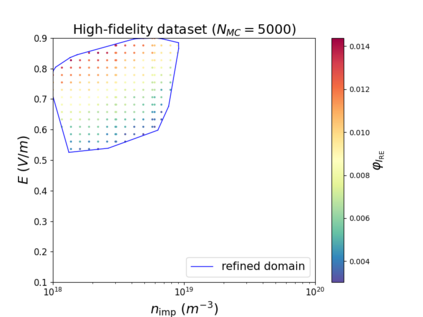
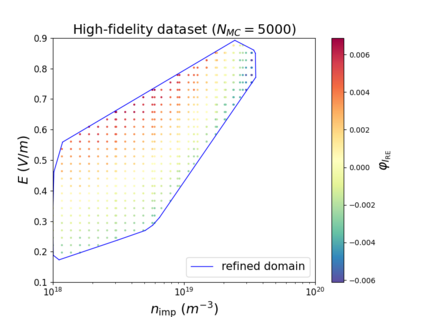
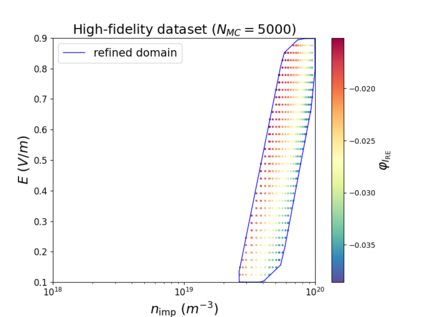
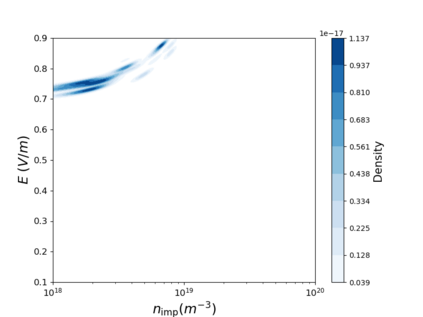
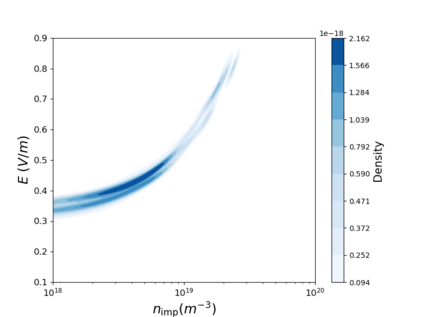
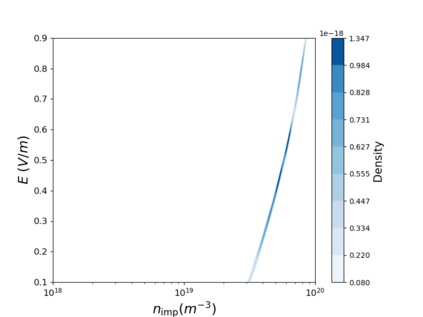
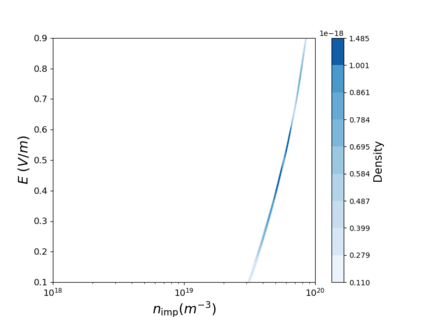
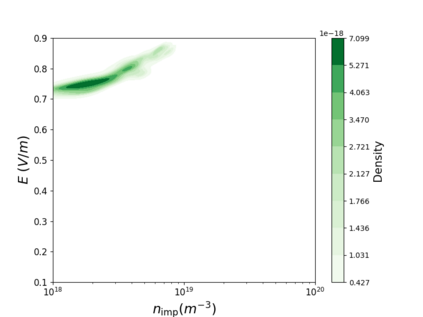
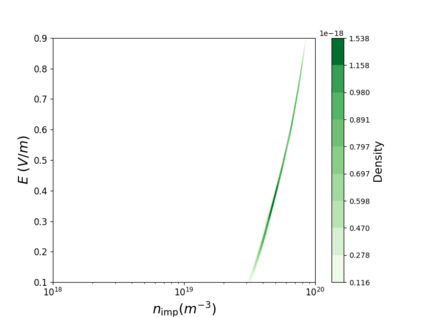
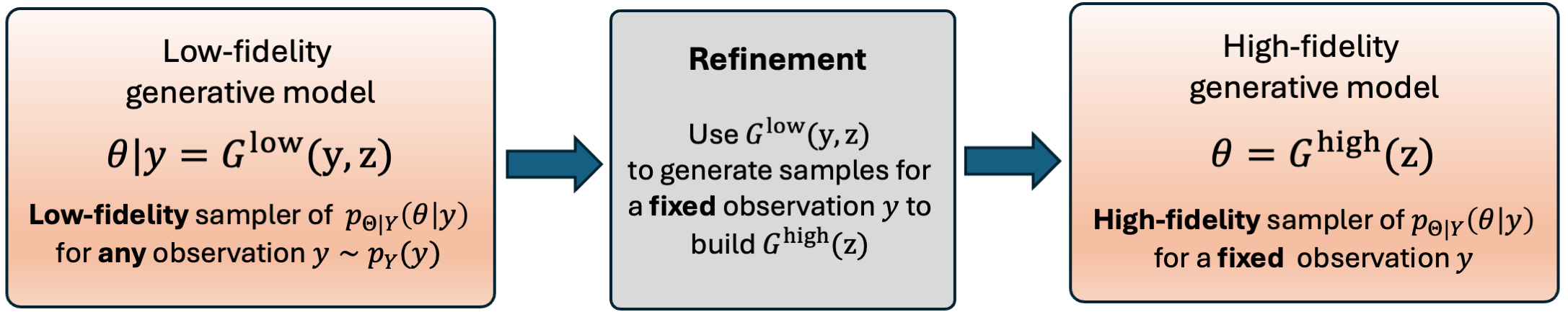
We present a multi-fidelity method for uncertainty quantification of parameter estimates in complex systems, leveraging generative models trained to sample the target conditional distribution. In the Bayesian inference setting, traditional parameter estimation methods rely on repeated simulations of potentially expensive forward models to determine the posterior distribution of the parameter values, which may result in computationally intractable workflows. Furthermore, methods such as Markov Chain Monte Carlo (MCMC) necessitate rerunning the entire algorithm for each new data observation, further increasing the computational burden. Hence, we propose a novel method for efficiently obtaining posterior distributions of parameter estimates for high-fidelity models given data observations of interest. The method first constructs a low-fidelity, conditional generative model capable of amortized Bayesian inference and hence rapid posterior density approximation over a wide-range of data observations. When higher accuracy is needed for a specific data observation, the method employs adaptive refinement of the density approximation. It uses outputs from the low-fidelity generative model to refine the parameter sampling space, ensuring efficient use of the computationally expensive high-fidelity solver. Subsequently, a high-fidelity, unconditional generative model is trained to achieve greater accuracy in the target posterior distribution. Both low- and high- fidelity generative models enable efficient sampling from the target posterior and do not require repeated simulation of the high-fidelity forward model. We demonstrate the effectiveness of the proposed method on several numerical examples, including cases with multi-modal densities, as well as an application in plasma physics for a runaway electron simulation model.
翻译:暂无翻译