Optimal Scoring Rule Design

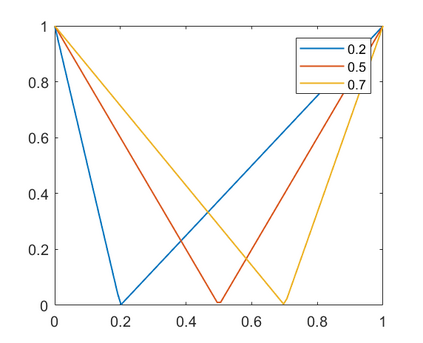
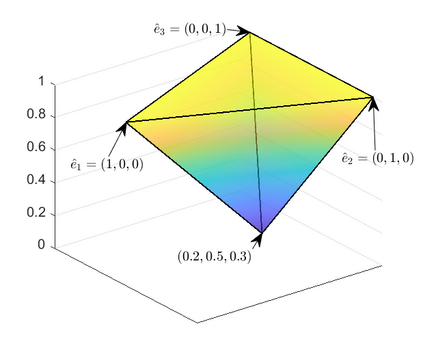
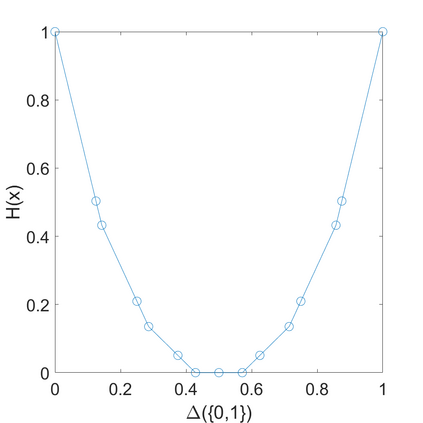
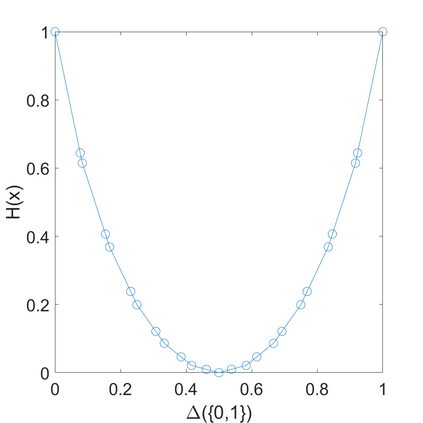
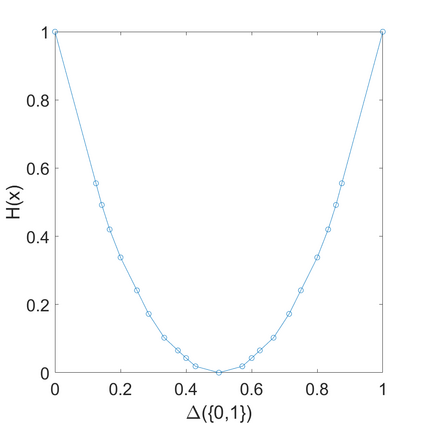
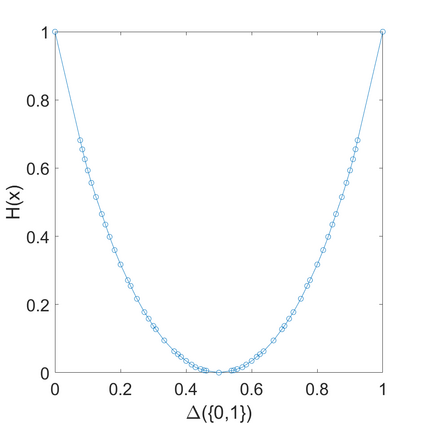
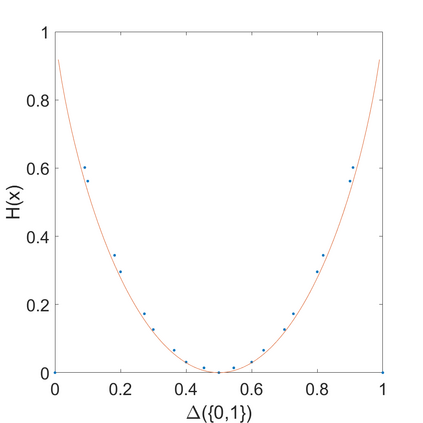
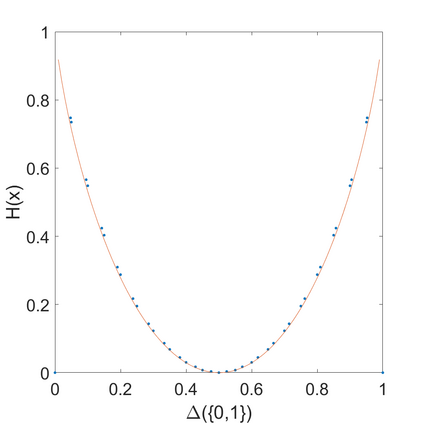
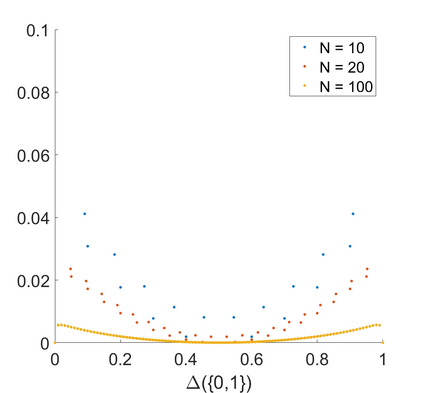
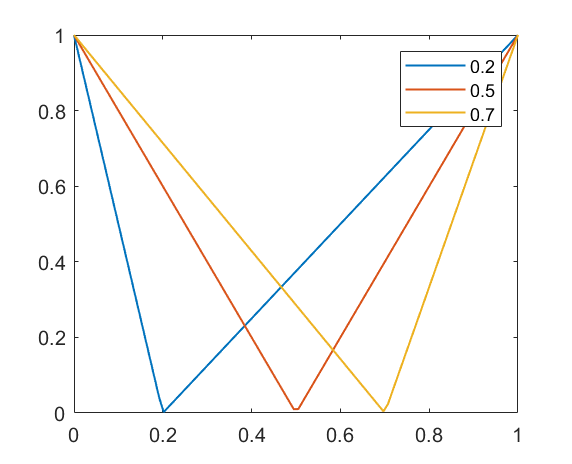
This paper introduces an optimization problem for proper scoring rule design. Consider a principal who wants to collect an agent's prediction about an unknown state. The agent can either report his prior prediction or access a costly signal and report the posterior prediction. Given a collection of possible distributions containing the agent's posterior prediction distribution, the principal's objective is to design a bounded scoring rule to maximize the agent's worst-case payoff increment between reporting his posterior prediction and reporting his prior prediction. We study two settings of such optimization for proper scoring rules: static and asymptotic settings. In the static setting, where the agent can access one signal, we propose an efficient algorithm to compute an optimal scoring rule when the collection of distributions is finite. The agent can adaptively and indefinitely refine his prediction in the asymptotic setting. We first consider a sequence of collections of posterior distributions with vanishing covariance, which emulates general estimators with large samples, and show the optimality of the quadratic scoring rule. Then, when the agent's posterior distribution is a Beta-Bernoulli process, we find that the log scoring rule is optimal. We also prove the optimality of the log scoring rule over a smaller set of functions for categorical distributions with Dirichlet priors.