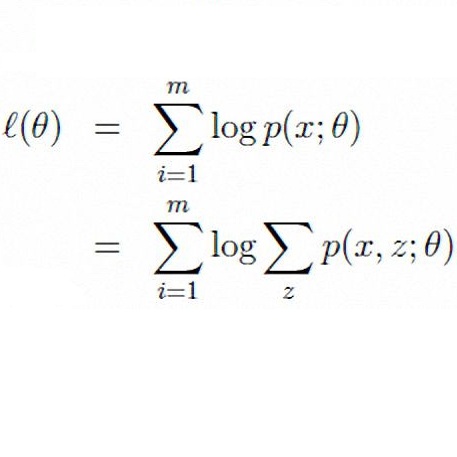模糊假设的统计检验理论和方法研究

项目名称： 模糊假设的统计检验理论和方法研究

项目编号： No.11261044

项目类型： 地区科学基金项目

立项/批准年度： 2013

项目学科： 数理科学和化学

项目作者： 魏立力

作者单位： 宁夏大学

项目金额： 45万元

中文摘要： 本项目拟比较系统地研究参数统计结构中模糊假设的检验问题，将假设命题表示为参数空间的模糊子集，适当定义两类错误的概率，发展相应假设的检验理论与方法。具体包括：研究不精确假设的似然比检验，构建相应假设的一般性检验方法；在精确样本信息和不精确样本信息两种情形下研究该假设的贝叶斯检验，探索贝叶斯因子的解释和集结样本信息的作用；发展和改进损失函数与后验风险等概念；利用可能性理论和非经典逻辑解释不精确假设的检验结果，与经典方法进行比较研究，为统计假设检验理论提供一个扩充和新的研究平台；同时研究不精确假设的序贯检验问题，包括指数分布和几何分布等具体分布参数的时间序贯计划问题，研究其贝叶斯停止法则和判决法则。由于模糊假设在某种意义下是人们真正想要检验的命题的更精准表示，模糊样本能够表示除变异性之外的不精确性，因此本项目研究不仅具有重要的理论意义，还具有现实意义。

中文关键词： 模糊假设；模糊数与区间数；多维标度；EM算法；混合copula模型

英文摘要： This project aims to systematically study imprecise hypothesis test problem in parametric statistical structure. The hypothesis propositions are expressed as the fuzzy subsets of parameter space, and then corresponding statistical testing theory and methods of these hypotheses will be developed based on appropriately defining probabilities of two types of errors. The main contents include in the following. Firstly, we will discuss likelihood ratio test of imprecise hypotheses and construct general testing method with respect to these imprecise hypotheses. Secondly, we will further study Bayesian testing of these hypotheses under the crisp sample data and fuzzy sample data respectively, and explore the interpretation of Bayesian factor and the role of aggregating sample information. The concepts of loss function and posteriori risk will be developed and improved at the same time. Thirdly, we will offer an extension of statistical hypothesis testing in the framework of possibility theory and non classic logics through interpreting the test results of imprecise hypothesis and comparing with the classical methods. Finally, we aim to study sequential testing problem of the imprecise hypothesis, especially Bayesian stopping rule and decision rule of time sequential plan, including the exponential distribution, geometr

英文关键词： fuzzy hypothsis；fuzzy number and interval data；multidimensional scaling；EM algorithm；mixed copula model