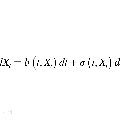二阶随机微分方程的Runge-Kutta方法研究

项目名称： 二阶随机微分方程的Runge-Kutta方法研究

项目编号： No.11301058

项目类型： 青年科学基金项目

立项/批准年度： 2014

项目学科： 数理科学和化学

项目作者： 王志勇

作者单位： 电子科技大学

项目金额： 22万元

中文摘要： 二阶随机微分方程因反映了很多自然现象的动力行为，已在物理、控制、生物和金融等领域有着重要的应用。目前，关于数值解方面的研究成果不多，只能将其转化成一阶系统来计算。但是直接对二阶系统本身进行数值模拟将更加有效。本项目将针对求解二阶随机微分方程，研究随机Runge-Kutta-Nystrom（SRKN）方法，我们将考察随机Nystrom树，发展Faa di Bruno公式，构造一般的SRKN方法的格式，并寻求方法的阶条件。利用嵌入的思想和最小均方误差准则，我们将构造具体的计算方法。进一步，将新算法与化系统为一阶方程的常用计算方法进行比较，分析稳定性和收敛性。同时，我们也将关注长时行为和系统的振荡性，研究随机Hamilton系统的辛方法和对称方法。

中文关键词： 随机微分方程；数值解；彩色树；Runge-Kutta；Parareal

英文摘要： Second-oder stochastic differential equations capture the dynamic behaviour of many natural phenomena, and have found applications in many fields such as Physics,Contorl, Biology and Fiance. Up to now, the outcomes about numerical simulations of second-order system are limited. As a usual way , such a system is transformed into a first order differential equations of doubled dimension by considering a new variable, then the classical methods such as Euler scheme can be uesed to solve the first-order system. But in many cases ,it is more advantageous and efficient to treat the second-order system directly rather than to convert them to first-order version. In this project, we will investigate the stochastic Runge-Kutta-Nystrom（SRKN）method to solve the second-order system directly.At first, the Nystrom tree will be studied, then the Faa di Bruno formula is to be developed. Secondly , we will provide the general SRKN scheme, and search the order condition. By applying the embedded technique and minimum mean-square error principal, we will contruct the concrete methods. Furthermore, comparing the new schemes and the familiar methods for second-order system, the stability and convergence will be investigated. On the other hand, we focus on the long-term behavior and the oscillation of second system, the symplectic a

英文关键词： stochastic differential equations；numerical solutions；color tree；Runge-Kutta；Parareal