Distributed Resource Allocation over Time-varying Balanced Digraphs with Discrete-time Communication

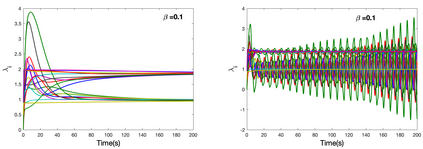
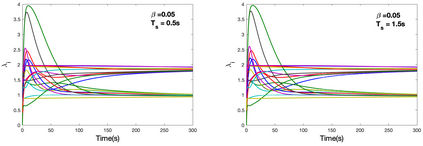
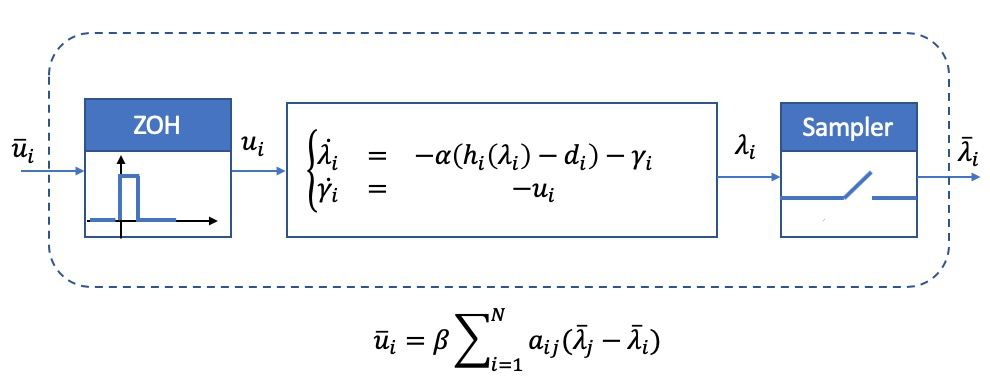
We propose a continuous-time algorithm for solving a resource allocation problem cooperatively and distributedly over a uniformly jointly strongly connected graph. Particularly, a novel passivity-based perspective of the proposed algorithmic dynamic at each individual node is provided, which enables us to analyze the convergence of the overall distributed algorithm over time-varying digraphs. The parameters in the proposed algorithm rely only on local information of each individual nodes, which can be designed in a truly distributed fashion. A periodic communication mechanism is also derived using the passivity degradation over sampling of the distributed dynamics in order to avoid the introduction of the restrictive assumption of continuous-time communication among nodes.