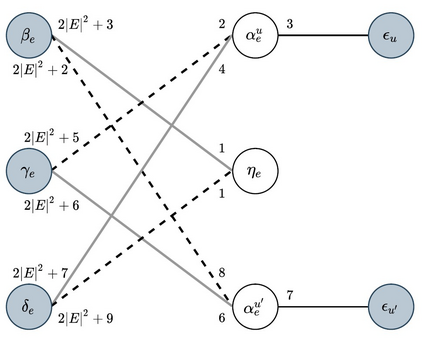
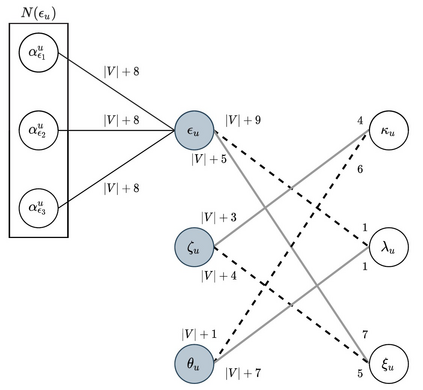
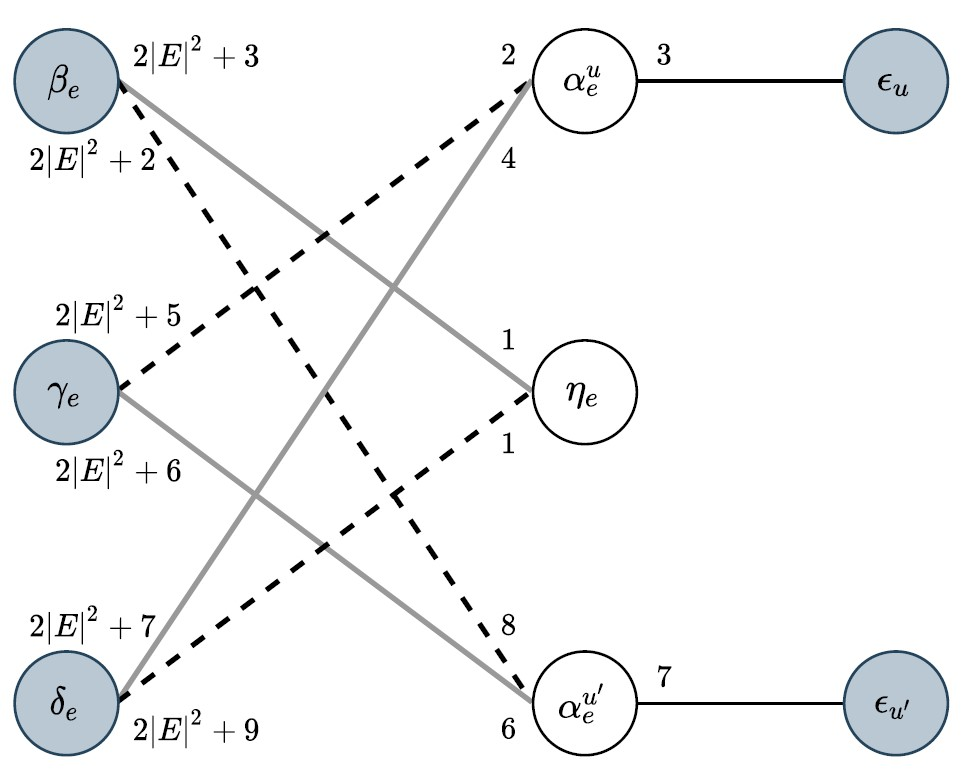
We study markets where a set of indivisible items is sold to bidders with unit-demand valuations, subject to a hard budget limit. Without financial constraints and pure quasilinear bidders, this assignment model allows for a simple ascending auction format that maximizes welfare and is incentive-compatible and core-stable. Introducing budget constraints, the ascending auction requires strong additional conditions on the unit-demand preferences to maintain its properties. We show that, without these conditions, we cannot hope for an incentive-compatible and core-stable mechanism. We design an iterative algorithm that depends solely on a trivially verifiable ex-post condition and demand queries, and with appropriate decisions made by an auctioneer, always yields a welfare-maximizing and core-stable outcome. If these conditions do not hold, we cannot hope for incentive-compatibility and computing welfare-maximizing assignments and core-stable prices is hard: Even in the presence of value queries, where bidders reveal their valuations and budgets truthfully, we prove that the problem becomes NP-complete for the assignment market model. The analysis complements complexity results for markets with more complex valuations and shows that even with simple unit-demand bidders the problem becomes intractable. This raises doubts on the efficiency of simple auction designs as they are used in high-stakes markets, where budget constraints typically play a role.
翻译:我们研究的是将一组不可分割的物品出售给具有单位-需求估值的投标人的市场,但须遵守严格的预算限制。没有财政限制和纯粹的准线性投标人,这种派任模式允许一种简单的上层拍卖形式,这种形式能够最大限度地提高福利,并且具有与激励兼容性和核心稳定。引入预算限制,上层拍卖要求对单位-需求偏好给予强有力的额外条件以维护其属性。我们表明,没有这些条件,我们无法指望一个与单位-需求估值相符的核心稳定机制。我们设计了一个迭代算法,它完全依赖于一个微不足道的可核实的事后条件和需求查询,并且由拍卖人做出适当的决定,这种分配模式总是产生一种福利最大化和核心稳定的结果。如果这些条件不可行,我们就无法指望奖励-兼容性和计算福利-需求偏重的任务和核心-稳定价格来维护其属性。我们证明,即使存在价值查询,而且投标人真实地披露其估值和预算,我们证明问题对于分配市场模式来说已经是全局性的。分析是对市场复杂性结果的补充,对于市场来说总是产生一种福利最大化的复杂的结果,而这种复杂的估价标准则是,在市场中也变得十分复杂,因为通常的市场需要。