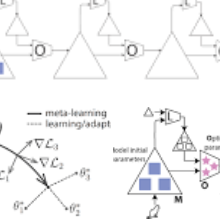
We propose a novel hierarchical Bayesian model for learning with a large (possibly infinite) number of tasks/episodes, which suits well the few-shot meta learning problem. We consider episode-wise random variables to model episode-specific target generative processes, where these local random variables are governed by a higher-level global random variate. The global variable helps memorize the important information from historic episodes while controlling how much the model needs to be adapted to new episodes in a principled Bayesian manner. Within our model framework, the prediction on a novel episode/task can be seen as a Bayesian inference problem. However, a main obstacle in learning with a large/infinite number of local random variables in online nature, is that one is not allowed to store the posterior distribution of the current local random variable for frequent future updates, typical in conventional variational inference. We need to be able to treat each local variable as a one-time iterate in the optimization. We propose a Normal-Inverse-Wishart model, for which we show that this one-time iterate optimization becomes feasible due to the approximate closed-form solutions for the local posterior distributions. The resulting algorithm is more attractive than the MAML in that it is not required to maintain computational graphs for the whole gradient optimization steps per episode. Our approach is also different from existing Bayesian meta learning methods in that unlike dealing with a single random variable for the whole episodes, our approach has a hierarchical structure that allows one-time episodic optimization, desirable for principled Bayesian learning with many/infinite tasks. The code is available at \url{https://github.com/minyoungkim21/niwmeta}.
翻译:暂无翻译