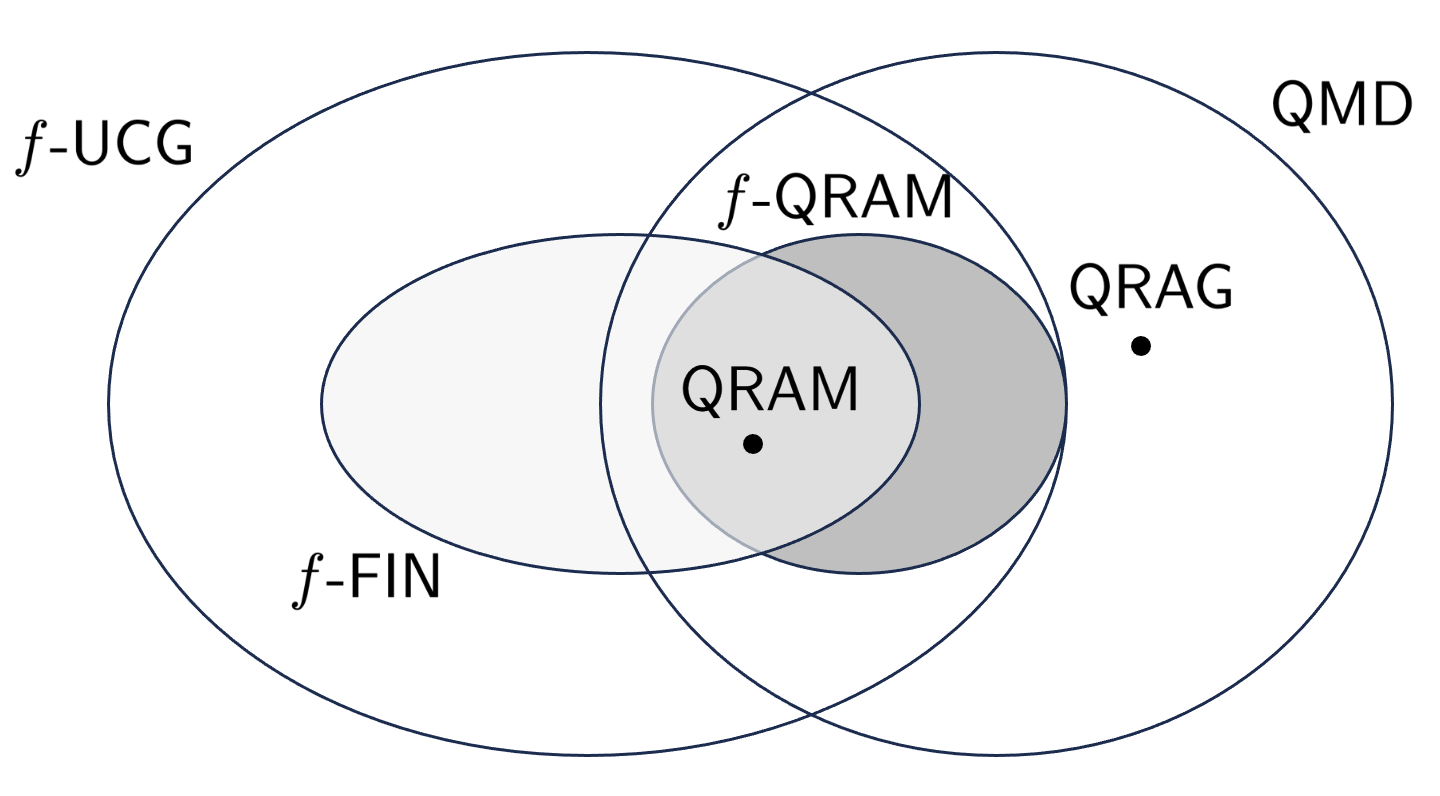
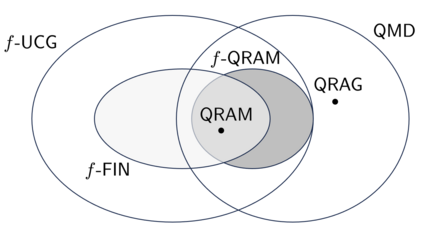
We explore the power of the unbounded Fan-Out gate and the Global Tunable gates generated by Ising-type Hamiltonians in constructing constant-depth quantum circuits, with particular attention to quantum memory devices. We propose two types of constant-depth constructions for implementing Uniformly Controlled Gates. These gates include the Fan-In gates defined by $|x\rangle|b\rangle\mapsto |x\rangle|b\oplus f(x)\rangle$ for $x\in\{0,1\}^n$ and $b\in\{0,1\}$, where $f$ is a Boolean function. The first of our constructions is based on computing the one-hot encoding of the control register $|x\rangle$, while the second is based on Boolean analysis and exploits different representations of $f$ such as its Fourier expansion. Via these constructions, we obtain constant-depth circuits for the quantum counterparts of read-only and read-write memory devices -- Quantum Random Access Memory (QRAM) and Quantum Random Access Gate (QRAG) -- of memory size $n$. The implementation based on one-hot encoding requires either $O(n\log{n}\log\log{n})$ ancillae and $O(n\log{n})$ Fan-Out gates or $O(n\log{n})$ ancillae and $6$ Global Tunable gates. On the other hand, the implementation based on Boolean analysis requires only $2$ Global Tunable gates at the expense of $O(n^2)$ ancillae.
翻译:暂无翻译