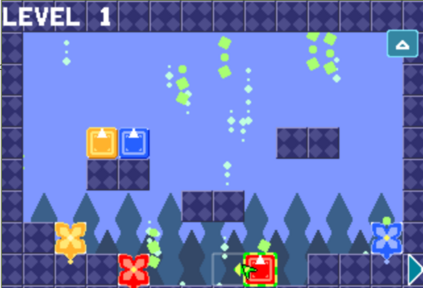
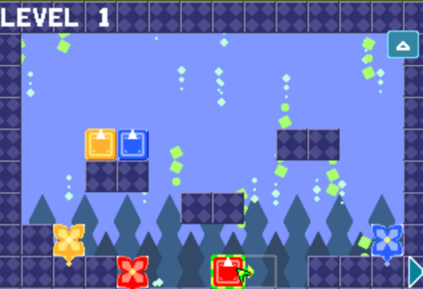
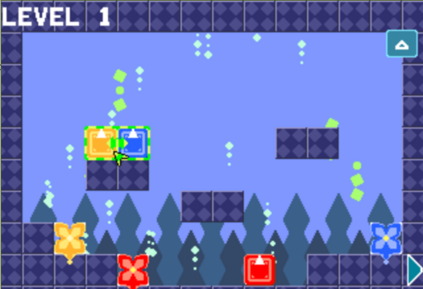
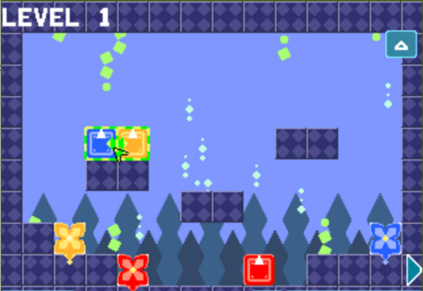
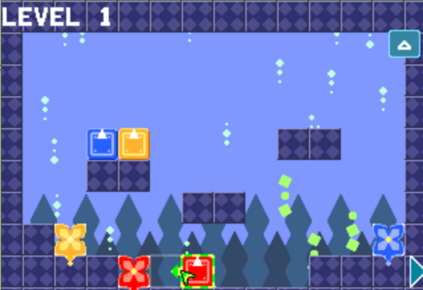
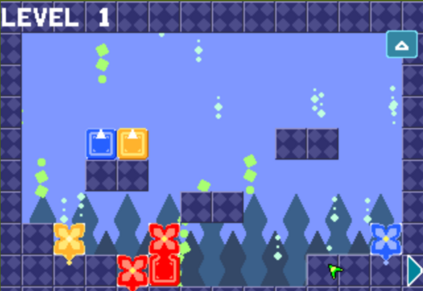
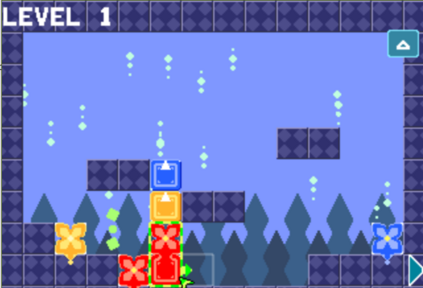
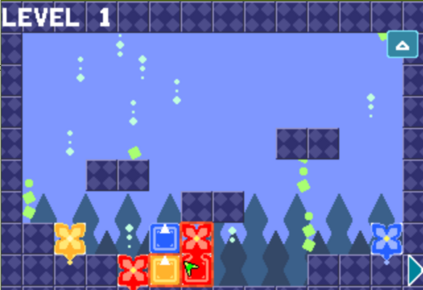
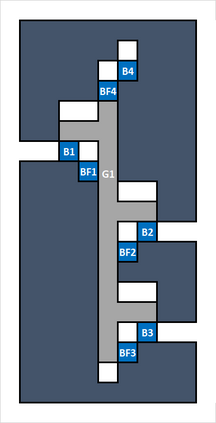
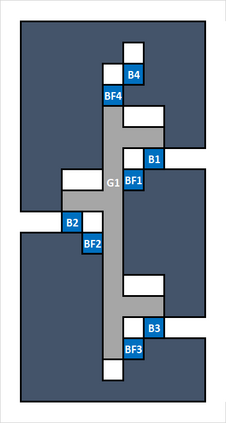
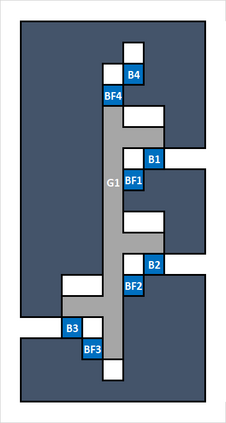
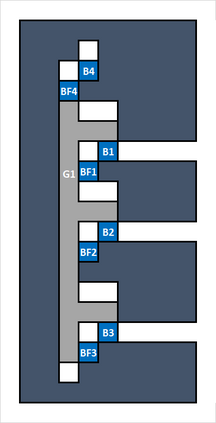
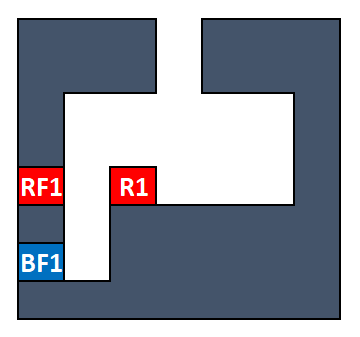
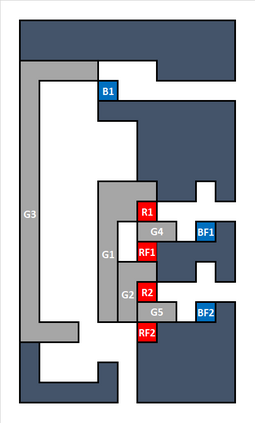
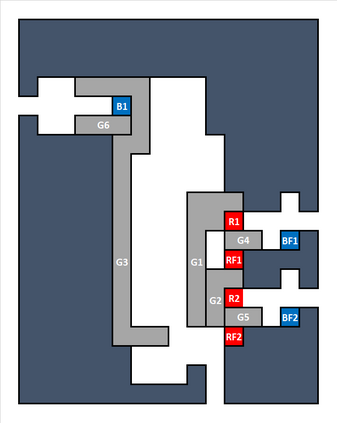
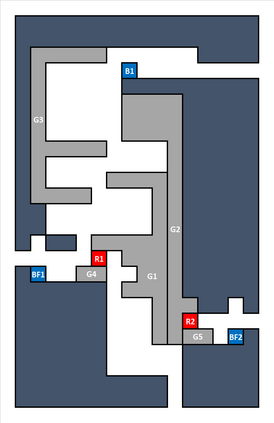
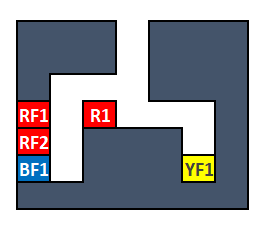
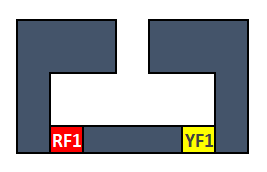
Liu and Yang recently proved the Hanano Puzzle to be ${\rm NP}$-$\leq_m^p$-hard. We prove it is in fact ${\rm PSPACE}$-$\leq_m^p$-complete. Our paper introduces the notion of a planar grid and establishes a relationship between planar grids and instances of the Nondeterministic Constraint Logic (${\rm NCL}$) problem (a known ${\rm PSPACE}$-$\leq_m^p$-complete problem) by using graph theoretic methods, and uses this connection to guide an indirect many-one reduction from the ${\rm NCL}$ problem to the Hanano Puzzle. The technique introduced is versatile and can be reapplied to other games with gravity.
翻译：刘和杨最近证明花野的拼图是$@rm NP}$-$\leq_m ⁇ p$-hard。我们证明它实际上是$@rm PSPACE}$-$\leq_m ⁇ p$-comp$-comple。我们的论文引入了平板电网的概念,并在平板电网和不确定性约束逻辑($rm NCL}$)实例之间建立了关系。通过使用图形方法,我们证明它是$@rm PSPACE}-$$\leq_m ⁇ p$-comple p$-comple roble 方法,我们用这个连接来引导将$@rm NCL}问题间接地从$@rm NCL$降为 Hanano 拼图。 引入的技术是多功能化的, 并且可以重新用于其他具有重力的游戏。
相关内容
微信扫码咨询专知VIP会员