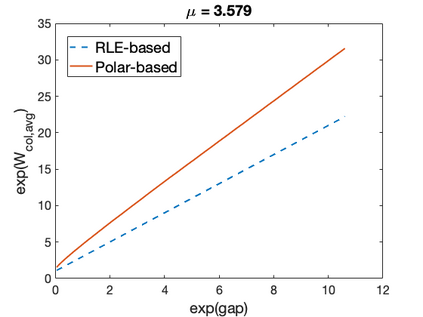
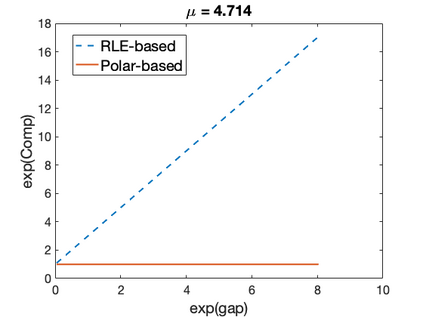
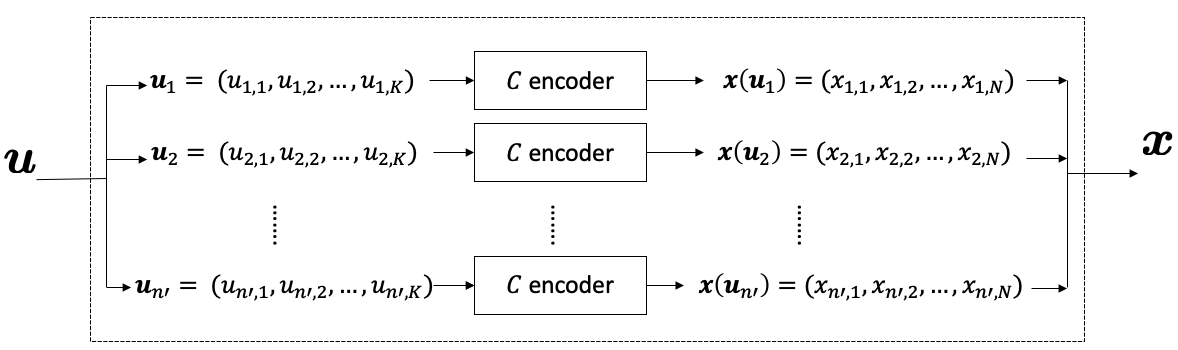
In this paper, we study codes with sparse generator matrices. More specifically, low-density generator matrix (LDGM) codes with a certain constraint on the weight of the columns in the generator matrix are considered. In this paper, it is first shown that when a BMS channel W and a constant s>0 are given, there exists a polarization kernel such that the corresponding polar code is capacity-achieving and the column weights of the generator matrix (GM) are bounded from above by $N^s$. Then, a general construction based on a concatenation of polar codes and a rate-$1$ code, and a new column-splitting algorithm that guarantees a much sparser GM, is given. More specifically, for any BMS channel and any $\epsilon > 2\epsilon^*$, where $\epsilon^* \approx 0.085$, an existence of a sequence of capacity-achieving codes with all the GM column weights upper bounded by $(\log N)^{1+\epsilon}$ is shown. Furthermore, two coding schemes for BEC and BMS channels, based on a second column-splitting algorithm, are devised with low-complexity decoding that uses successive-cancellation. The second splitting algorithm allows for the use of a low-complexity decoder by preserving the reliability of the bit-channels observed by the source bits, and by increasing the code block length. The concatenation-based construction can also be applied to the random linear code ensemble to yield capacity-achieving codes with all the GM column weights being $O(\log N)$ and with (large-degree) polynomial decoding complexity.
翻译:在本文中,我们用零散的发电机矩阵研究代码。 更具体地说, 考虑低密度发电机矩阵( LDGM) 代码, 并在一定程度上限制发电机矩阵中列数的重量。 在本文中, 首先显示, 当给出 BMS 频道 W 和 常数 s> 0 时, 存在一个两极分化内核, 相应的极值代码正在实现能力, 而发电机矩阵( GM) 的列重量从上面限制在$N%s$。 然后, 一个基于极地代码和速- 1美元代码的总结构( LDGM), 以及一个新的分层计算算法, 保证大大分散的 GMT。 更具体地说, 任何 BMS 频道和 $\ epsilon > 2\ epslon $, 其中, 相应的极值代码是 0.85, 与 $( log NN) 和 ral- comlical 代码 一起, 显示BEC 和 com- decal comlial dal dal dal dal 使用 的两种计算方法, 和 正在制成的NCal- decal- decal- dal- dal- dal dal dalbalation, 使用, 数据系统使用, 和 等 和 等 等 等 等 等 的,, 和 等 的计算法,,, 和 等 的 的 的 的 等 的 的 的 等 的 的 的 和, 和 和 和 等 等 等 的 等 等 等 的 的 的 等 的 的 的 的 的 的 的 的 的 的 的 的 的 的 的 的 的 的 的 和 的 的 和 的 和 等的 等的 的 的 的 等的 的 的 的 的 的 的 的 的 的 和 和 等的 等的 的 的 的 的 的 的 等的 等的 的