Torque Computation with the Isogeometric Mortar Method for the Simulation of Electric Machines

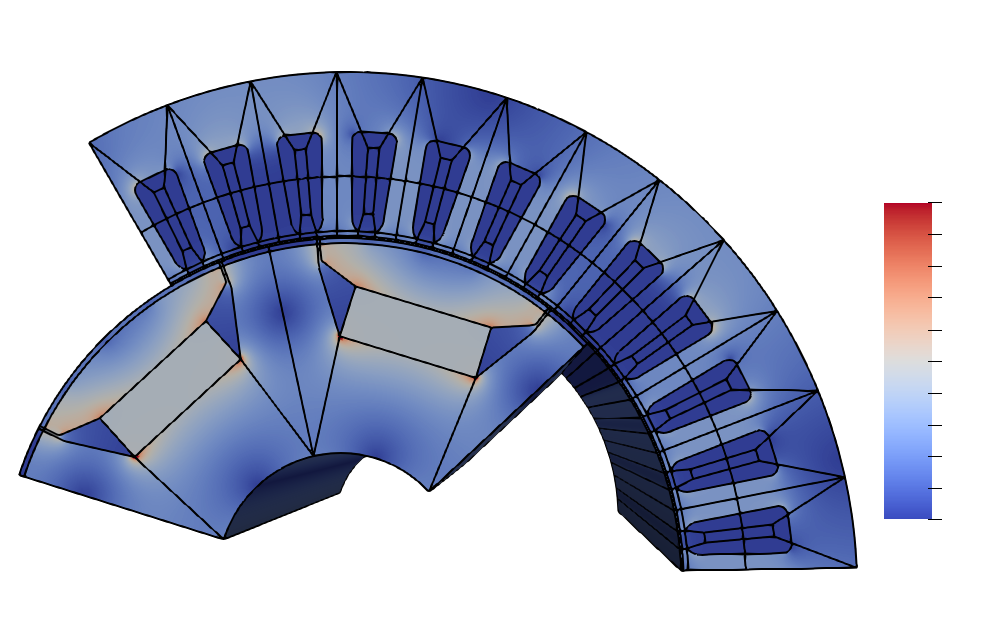
In this work isogeometric mortaring is used for the simulation of a six pole permanent magnet synchronous machine. Isogeometric mortaring is especially well suited for the efficient computation of rotating electric machines as it allows for an exact geometry representation for arbitrary rotation angles without the need of remeshing. The appropriate B-spline spaces needed for the solution of Maxwell's equations and the corresponding mortar spaces are introduced. Unlike in classical finite element methods their construction is straightforward in the isogeometric case. The torque in the machine is computed using two different methods, i.e., Arkkio's method and by using the Lagrange multipliers from the mortaring.