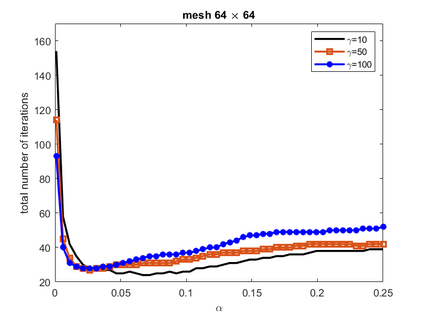
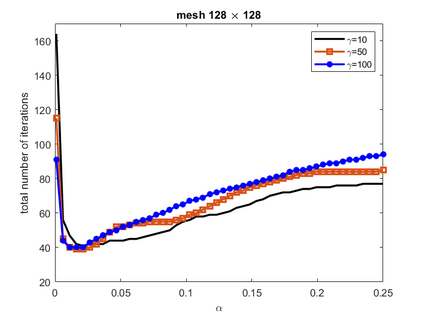
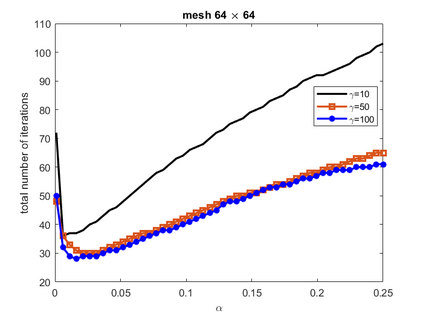
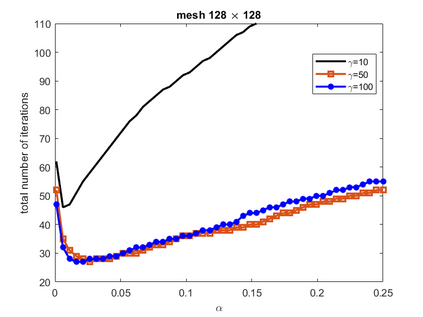
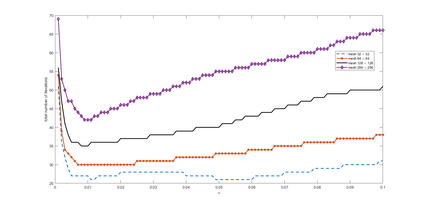
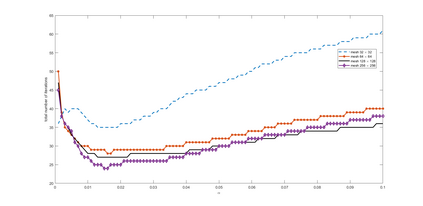
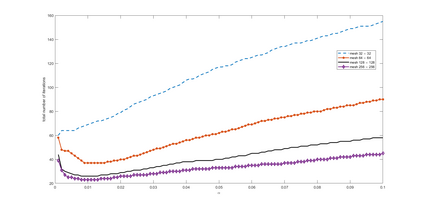
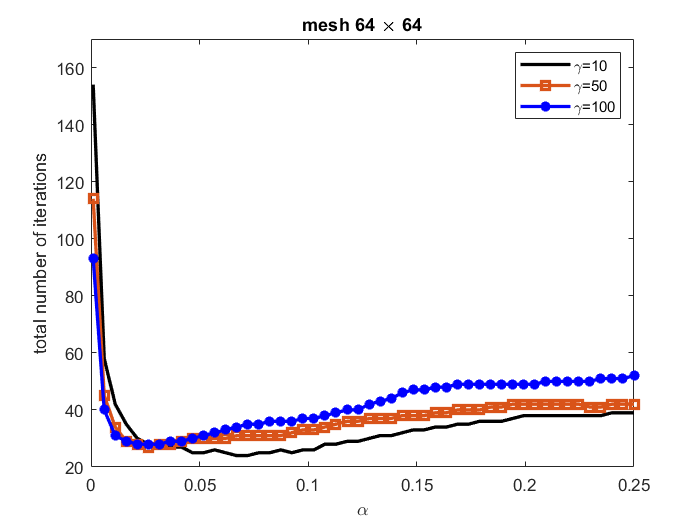
We consider the iterative solution of large linear systems of equations in which the coefficient matrix is the sum of two terms, a sparse matrix $A$ and a possibly dense, rank deficient matrix of the form $\gamma UU^T$, where $\gamma > 0$ is a parameter which in some applications may be taken to be 1. The matrix $A$ itself can be singular, but we assume that the symmetric part of $A$ is positive semidefinite and that $A+\gamma UU^T$ is nonsingular. Linear systems of this form arise frequently in fields like optimization, fluid mechanics, computational statistics, and others. We investigate preconditioning strategies based on an alternating splitting approach combined with the use of the Sherman-Morrison-Woodbury matrix identity. The potential of the proposed approach is demonstrated by means of numerical experiments on linear systems from different application areas.
翻译:我们认为,大型线性方程系统的迭代解决办法是,系数矩阵是两个条件的总和,一个是稀薄的基质$A$,一个可能是密集的、排位不足的基质,形式为$$gamma UU ⁇ T$,在有些应用中,美元 > 0美元是一个参数,在某些应用中可以认为是1. 基质$本身可以是单数,但我们假设,美元基数的对称部分是正半无穷,而美元是非定值的。这种形式的线性系统经常出现在优化、液力力、计算统计等领域。我们调查基于交替分解法的前提条件战略,同时使用谢尔曼-莫里森-沃德伯里矩阵特征。 提议的方法的潜力通过不同应用领域的线性系统的数字实验而得到证明。