Some Properties and Applications of Burr III-Weibull Distribution

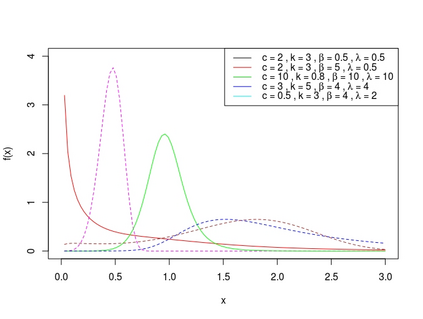
In this paper, we introduce a new distribution called Burr III-Weibull(BW) distribution using the concept of competing risk. We derive moments, conditional moments, mean deviation and quantiles of the proposed distribution. Also the Renyi's entropy and order statistics of the distribution are obtained. Estimation of parameters of the distribution is performed via maximum likelihood method. A simulation study is performed to validate the maximum likelihood estimator (MLE). A real practical data set is analyzed for illustration.