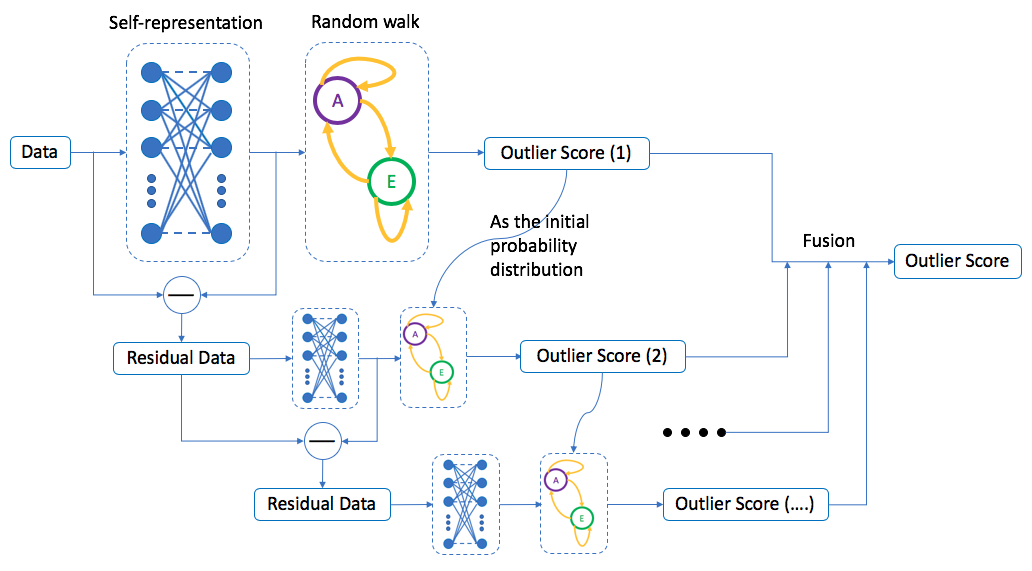
Many methods based on sparse and low-rank representation been developed along with guarantees of correct outlier detection. Self-representation states that a point in a subspace can always be expressed as a linear combination of other points in the subspace. A suitable Markov Chain can be defined on the self-representation and it allows us to recognize the difference between inliers and outliers. However, the reconstruction error of self-representation that is still informative to detect outlier detection, is neglected.Inspired by the gradient boosting, in this paper, we propose a new outlier detection framework that combines a series of weak "outlier detectors" into a single strong one in an iterative fashion by constructing multi-pass self-representation. At each stage, we construct a self-representation based on elastic-net and define a suitable Markov Chain on it to detect outliers. The residual of the self-representation is used for the next stage to learn the next weaker outlier detector. Such a stage will repeat many times. And the final decision of outliers is generated by the previous all results. Experimental results on image and speaker datasets demonstrate its superiority with respect to state-of-the-art sparse and low-rank outlier detection methods.
翻译:暂无翻译