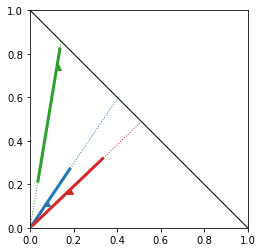
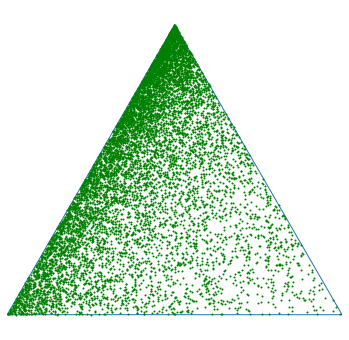
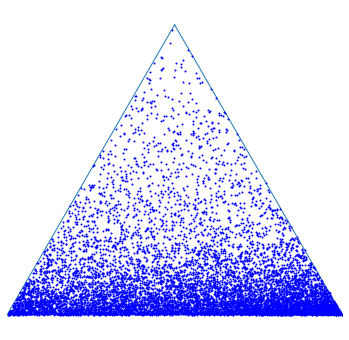
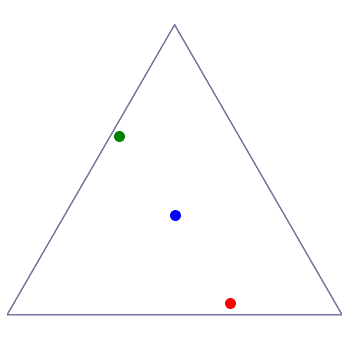
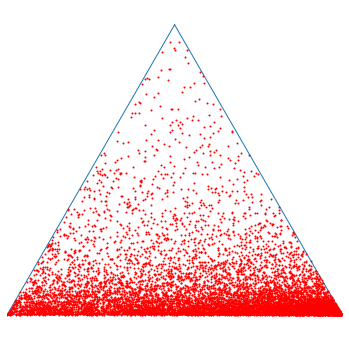
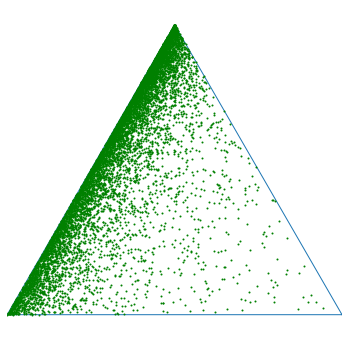
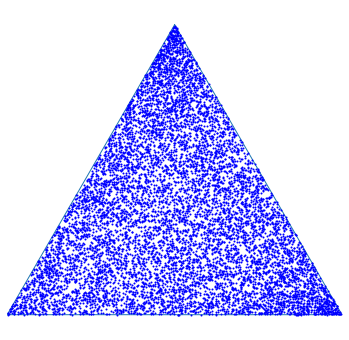
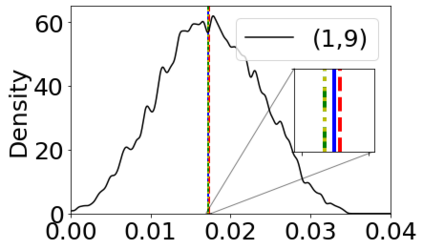
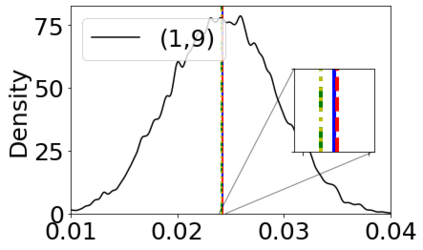
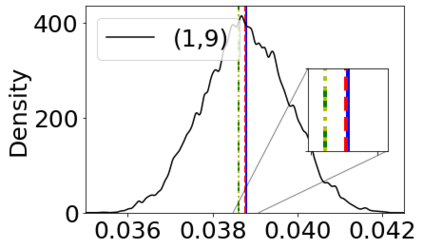
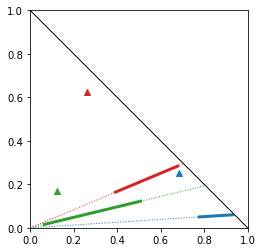
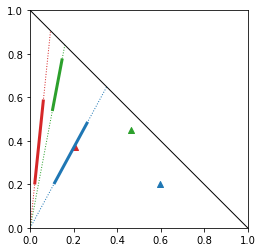
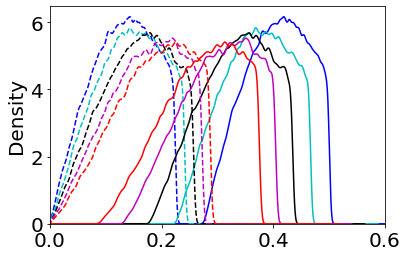
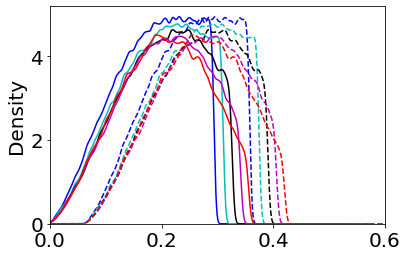
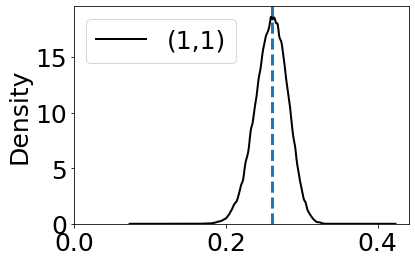
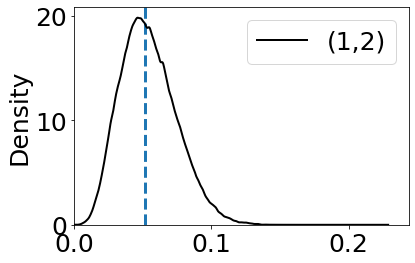
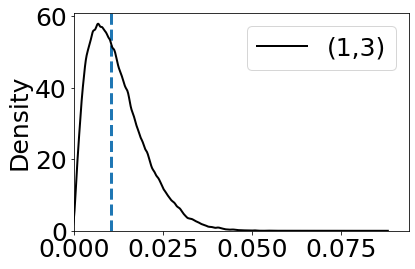
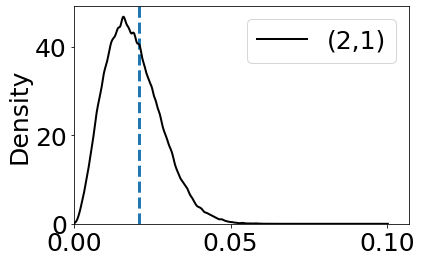
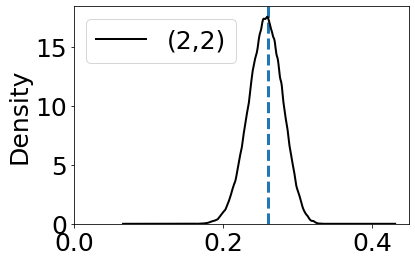
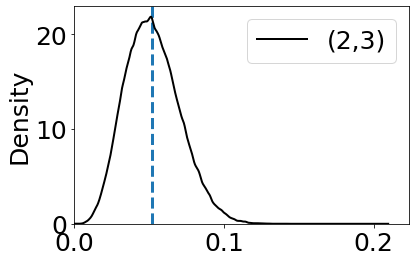
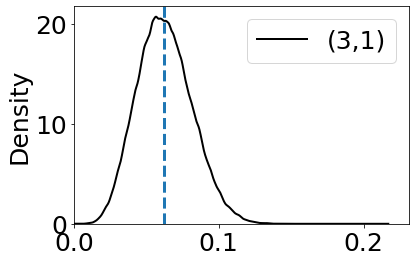
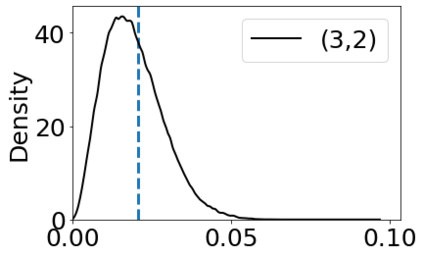
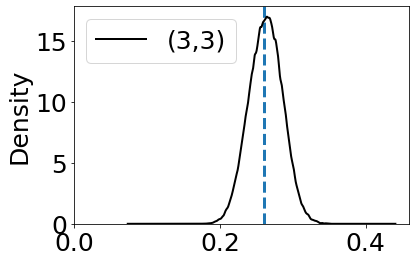
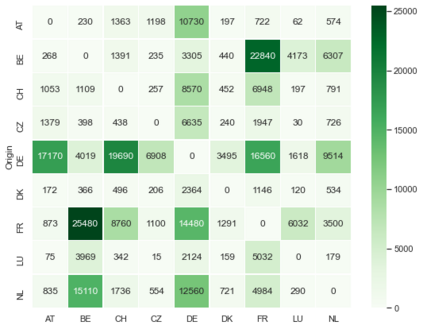
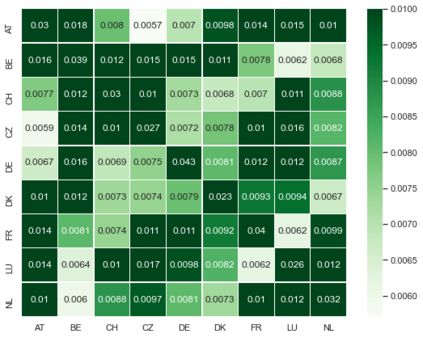
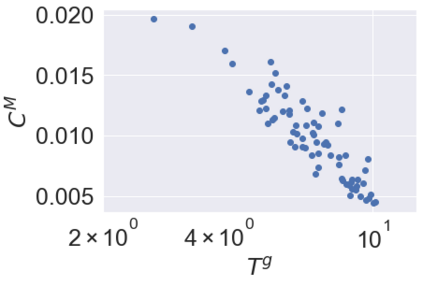
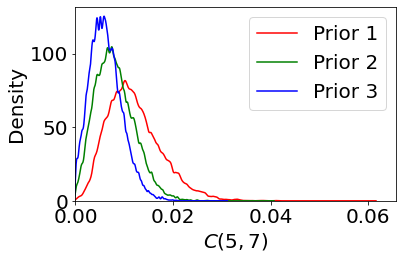
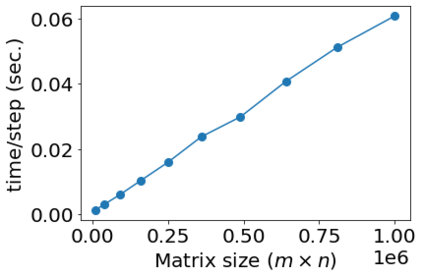
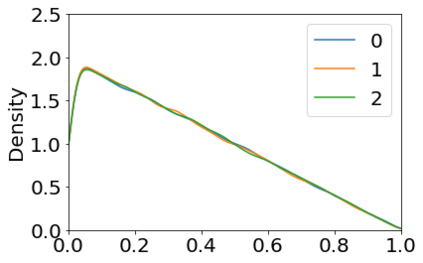
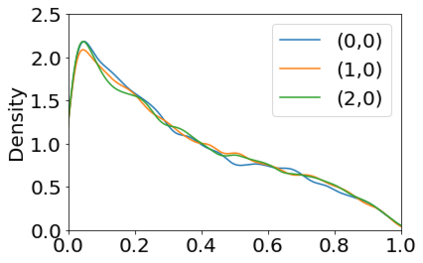
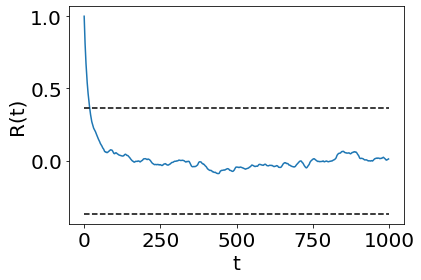
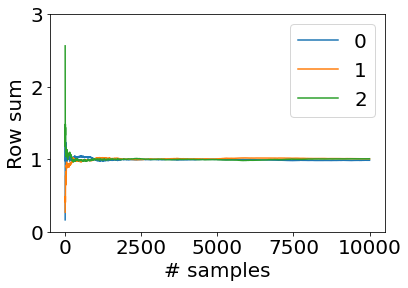
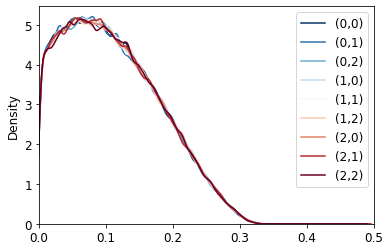
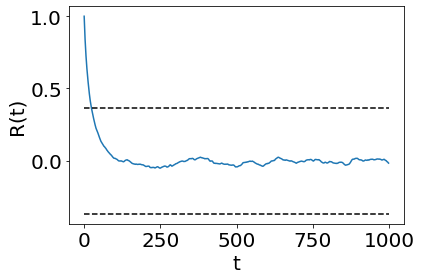
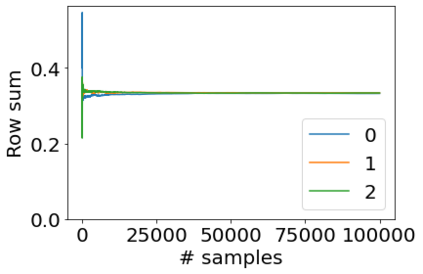
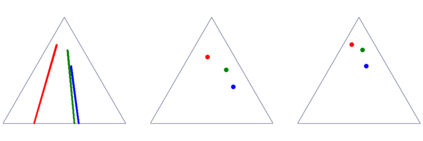
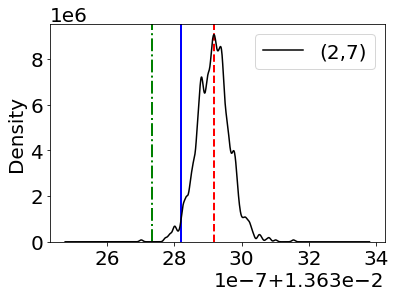
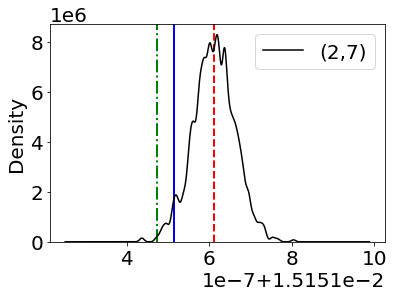
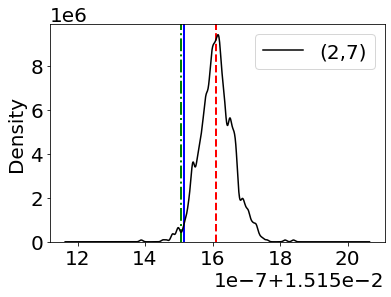
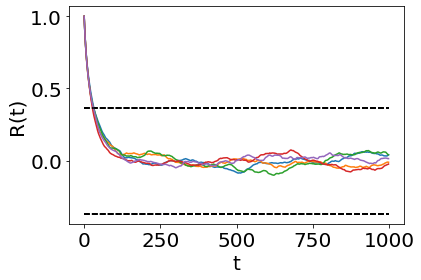
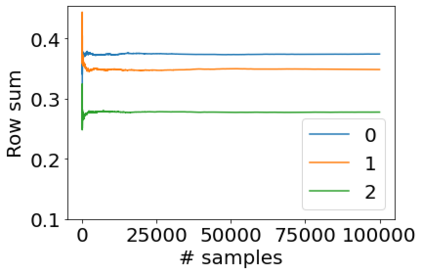
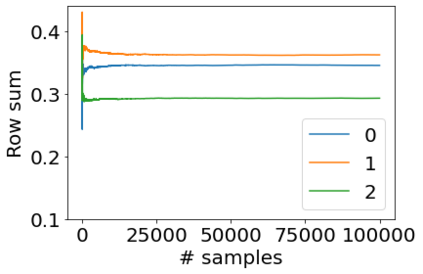
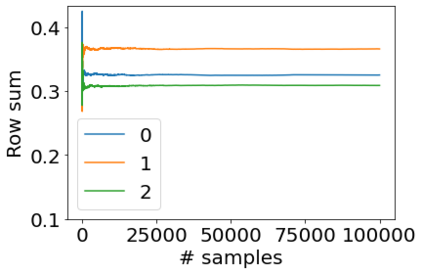
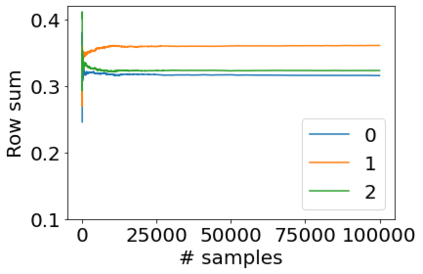
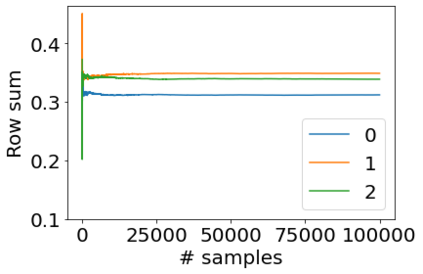
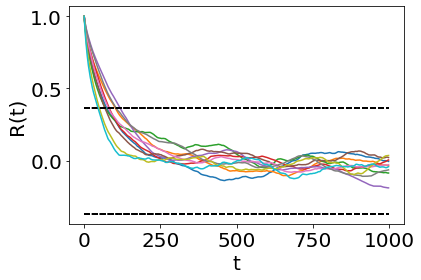
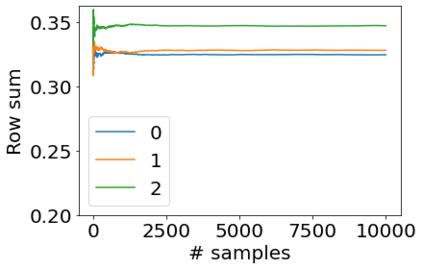
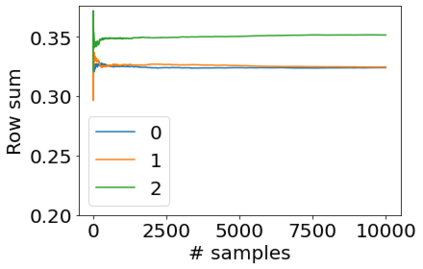
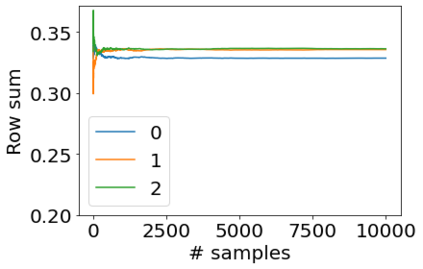
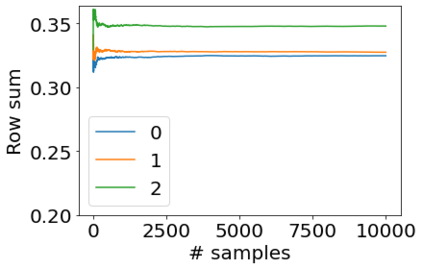
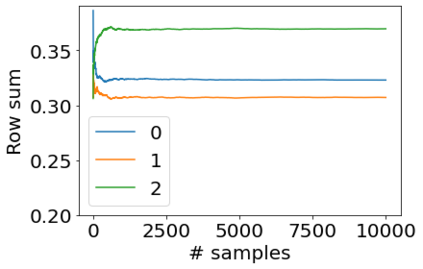
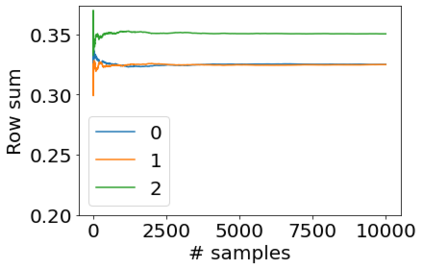
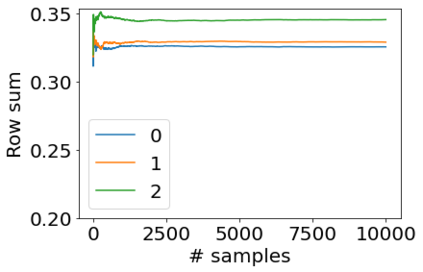
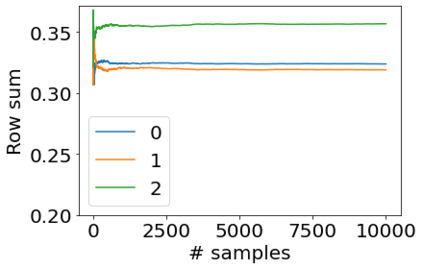
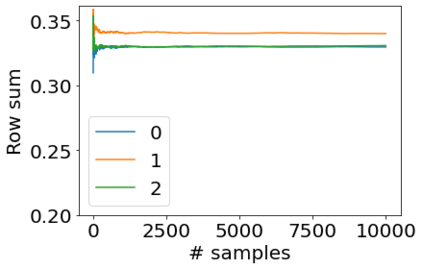
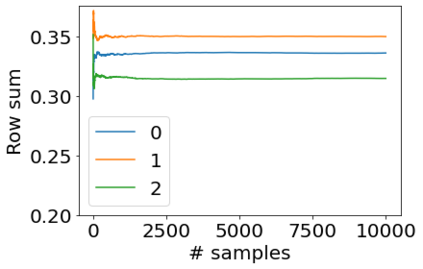
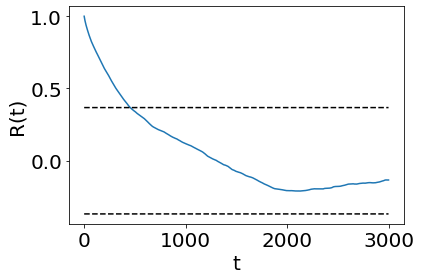
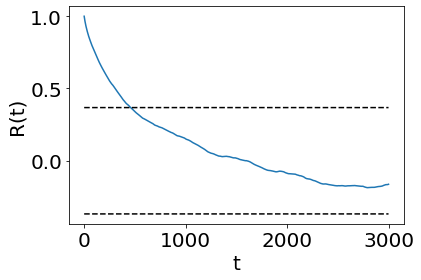
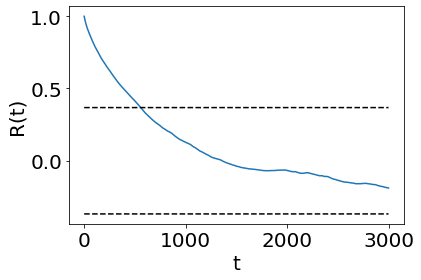
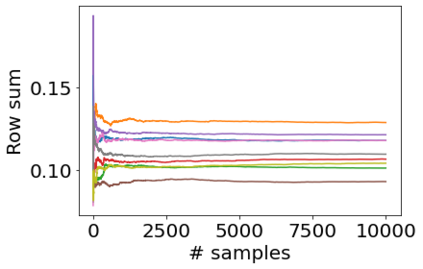
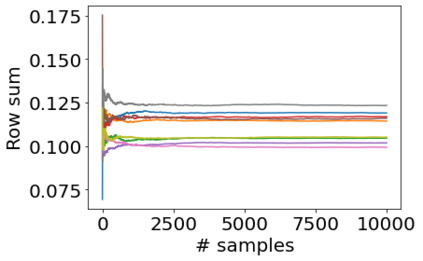
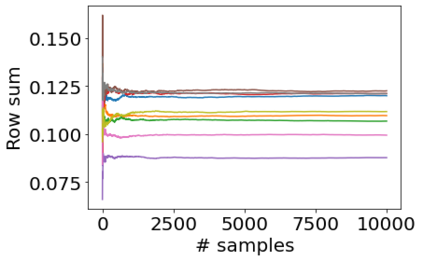
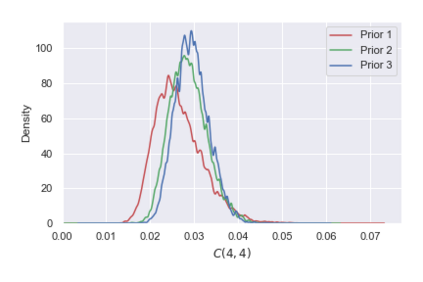
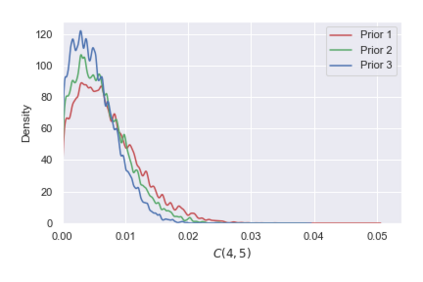
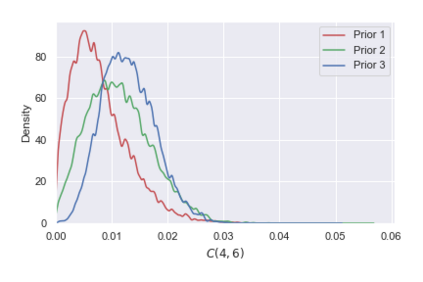
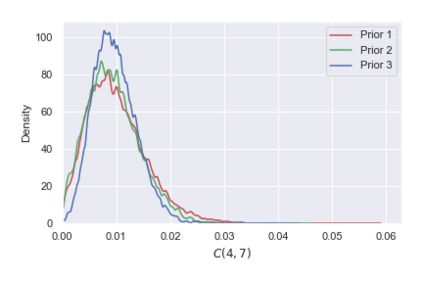
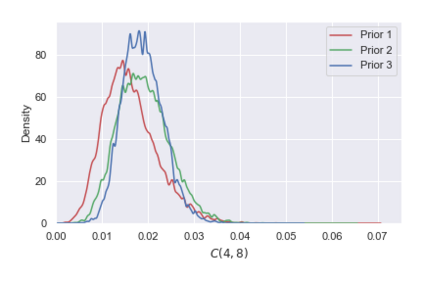
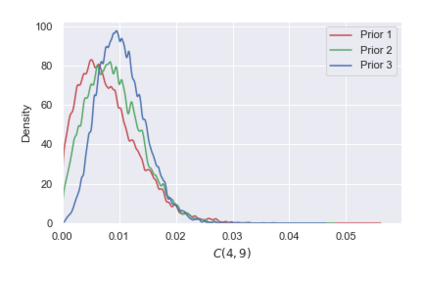
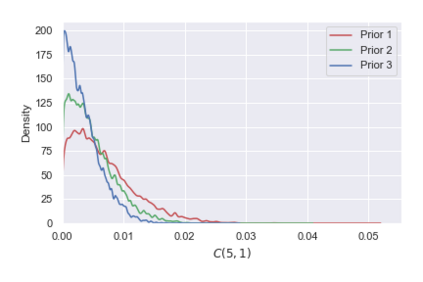
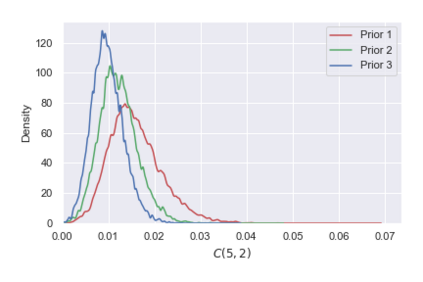
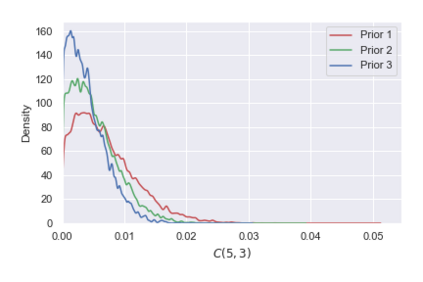
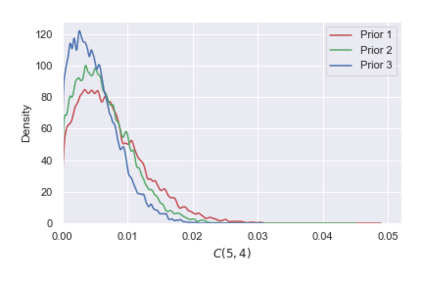
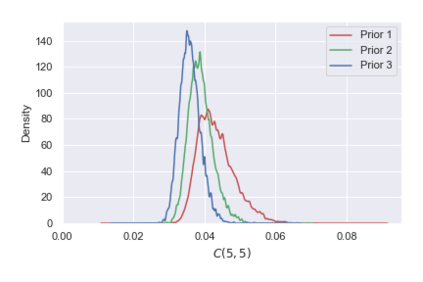
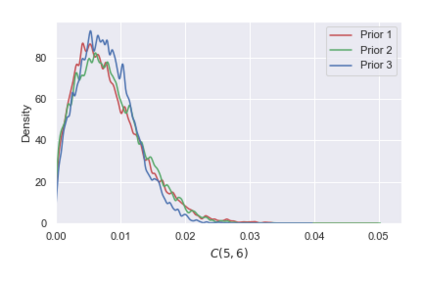
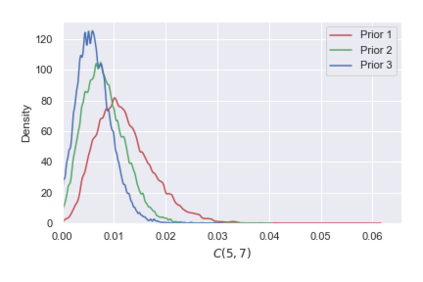
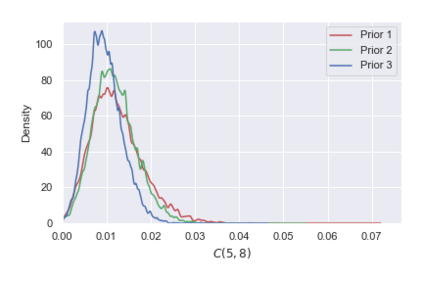
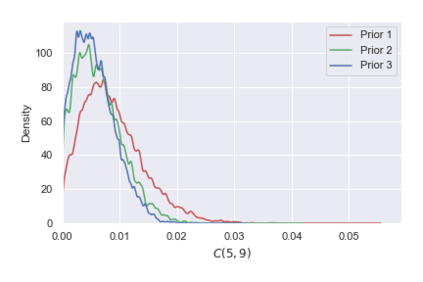
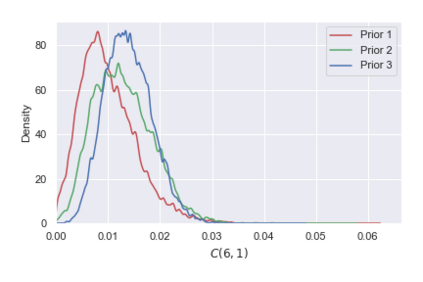
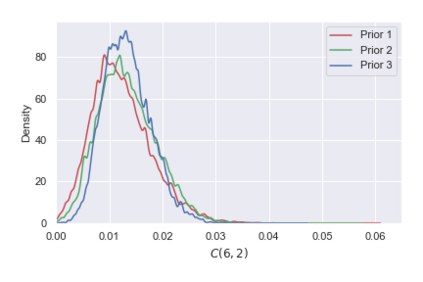
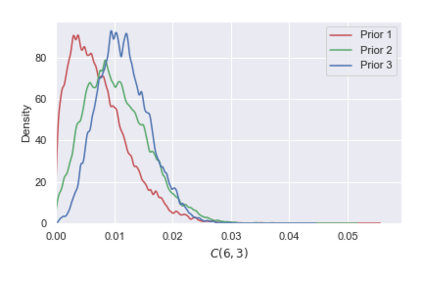
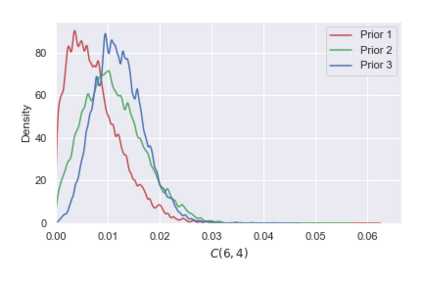
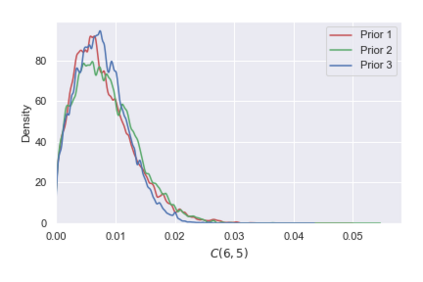
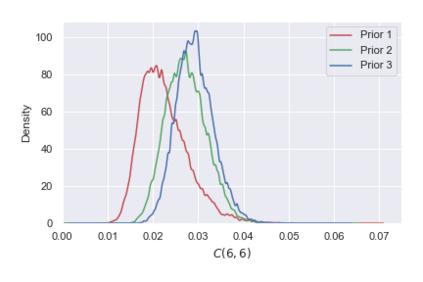
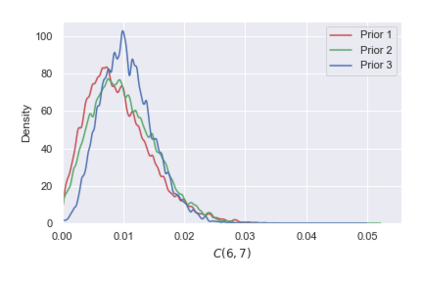
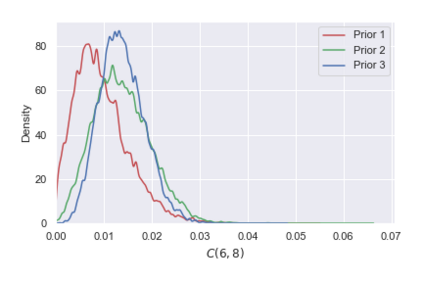
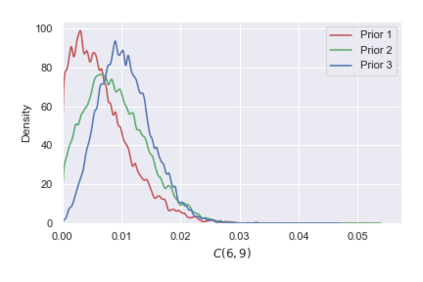
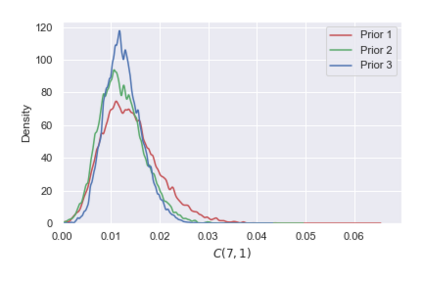
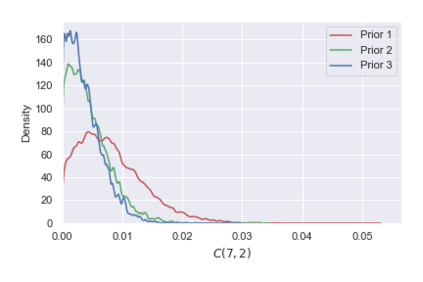
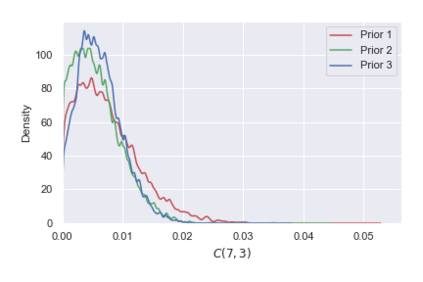
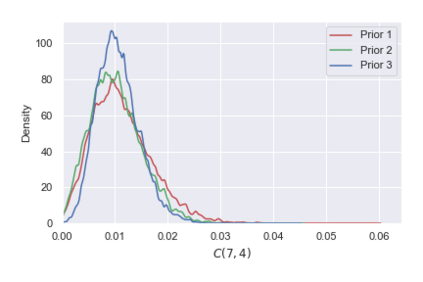
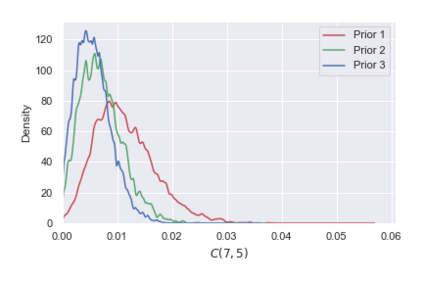
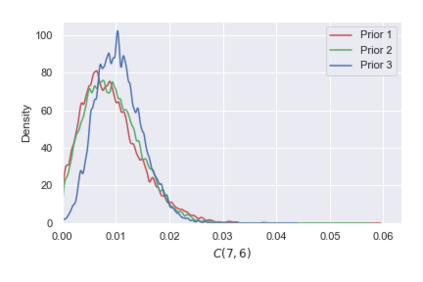
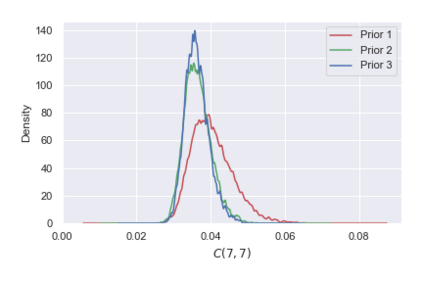
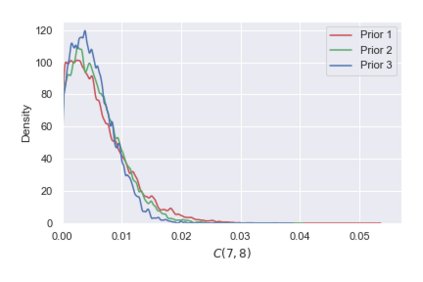
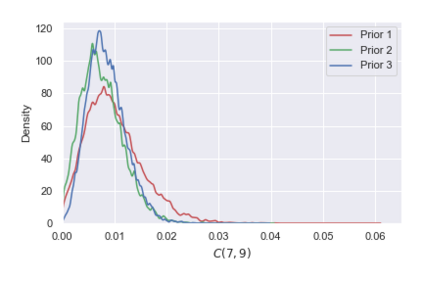
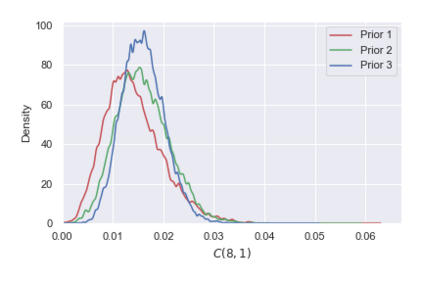
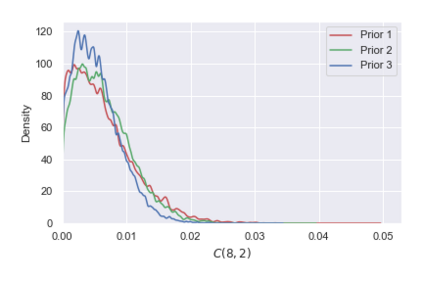
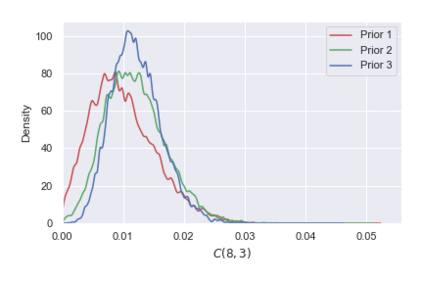
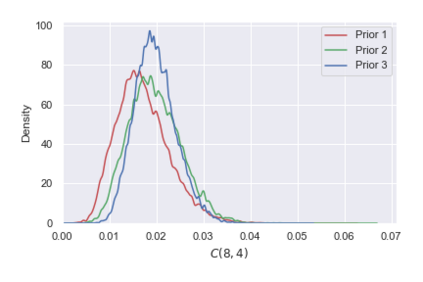
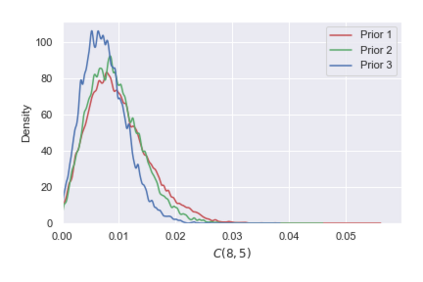
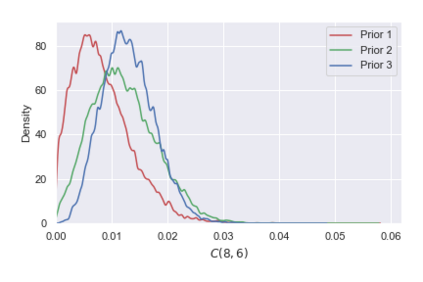
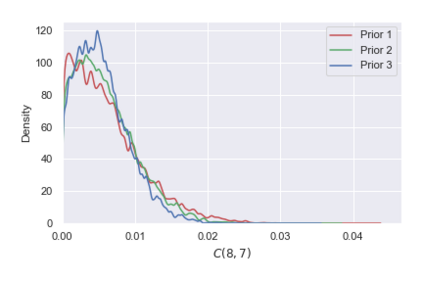
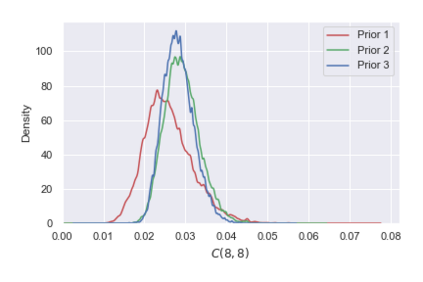
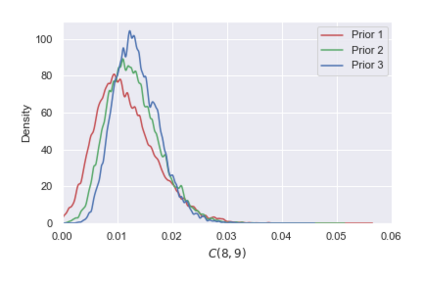
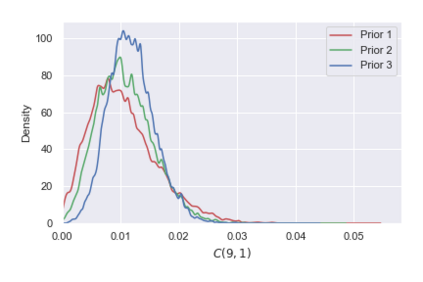
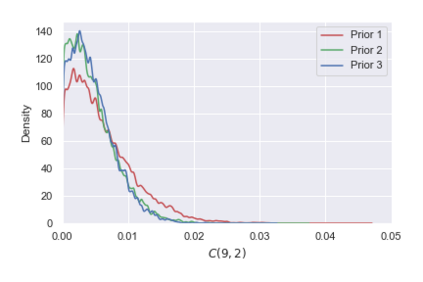
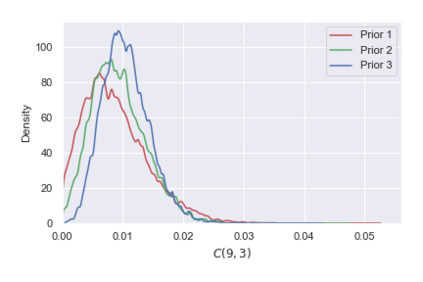
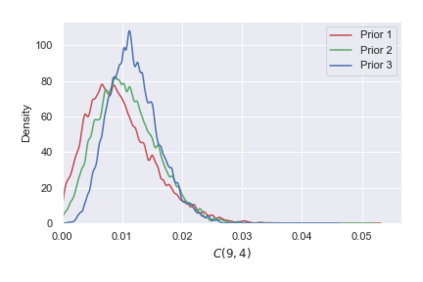
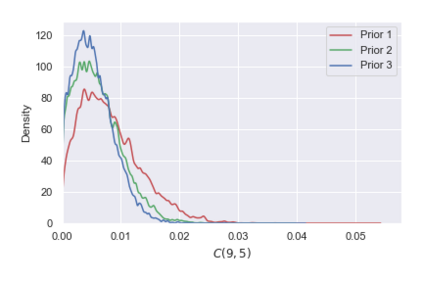
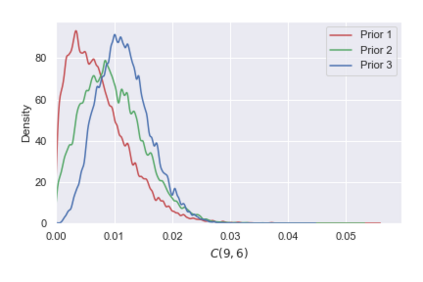
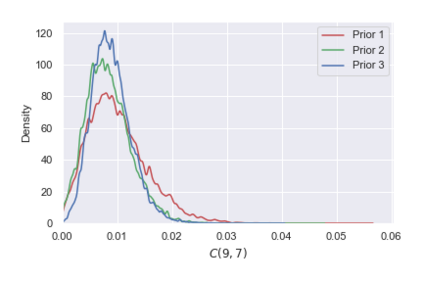
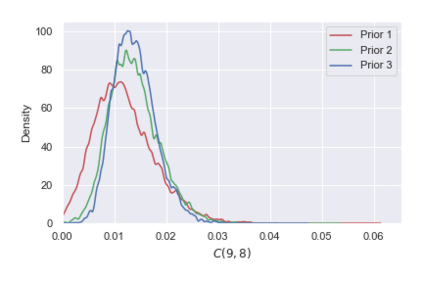
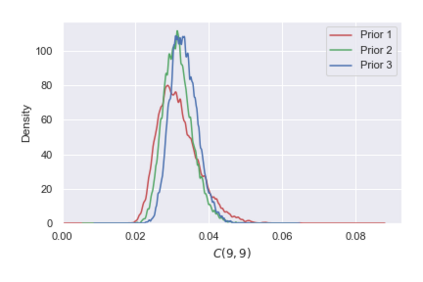
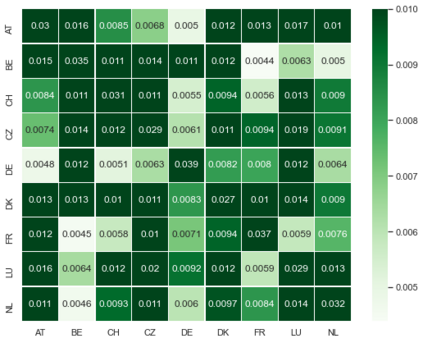
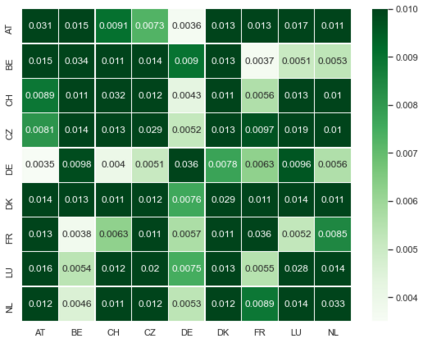
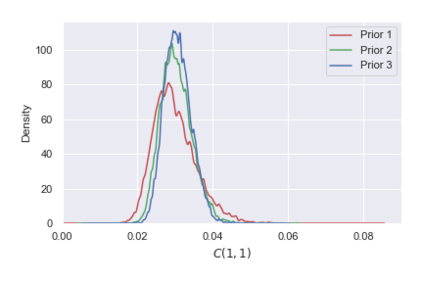
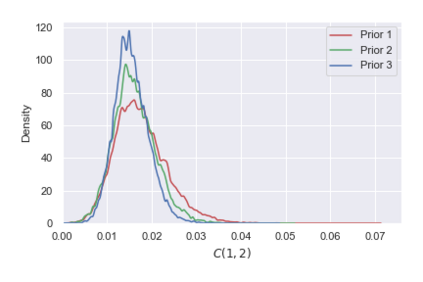
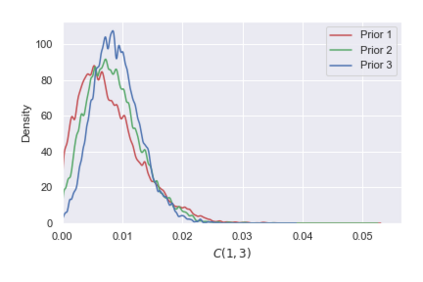
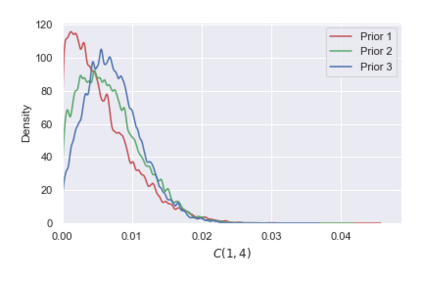
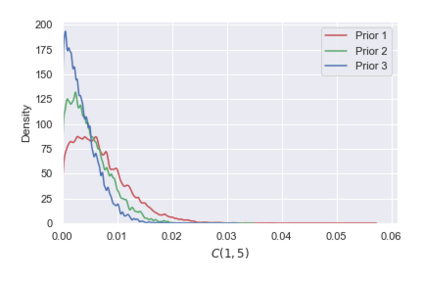
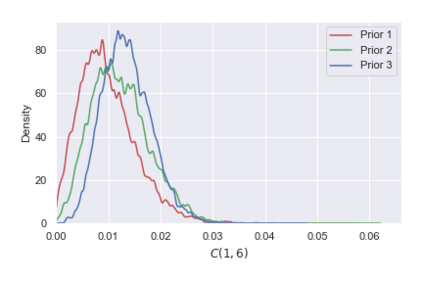
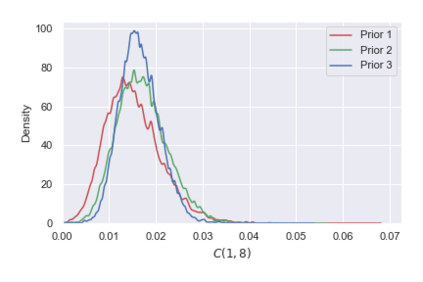
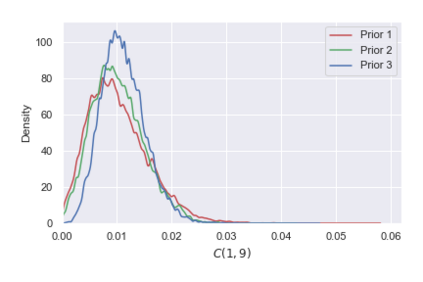
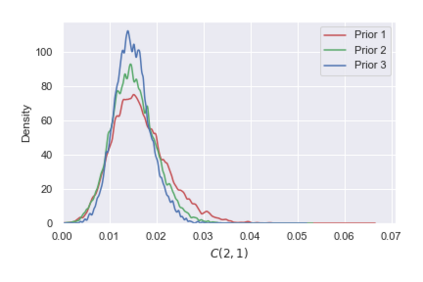
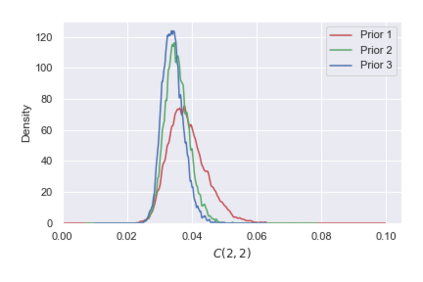
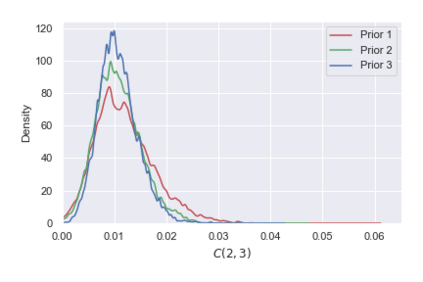
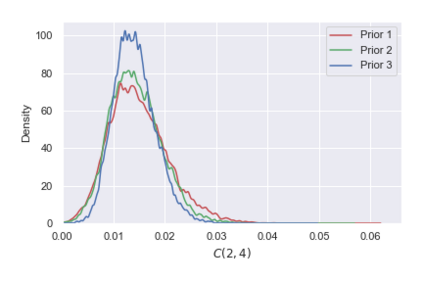
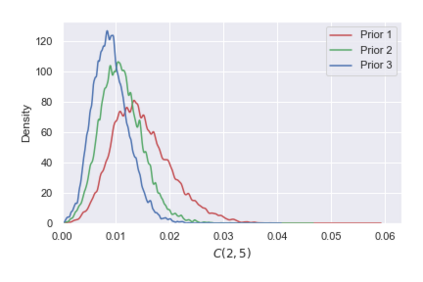
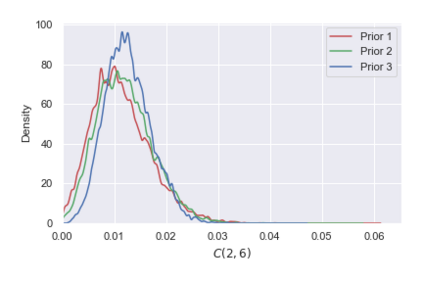
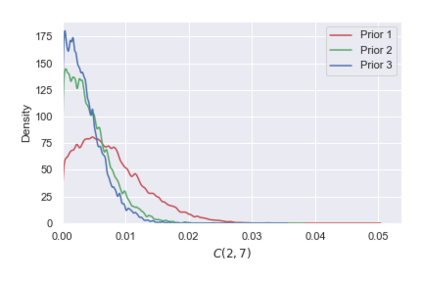
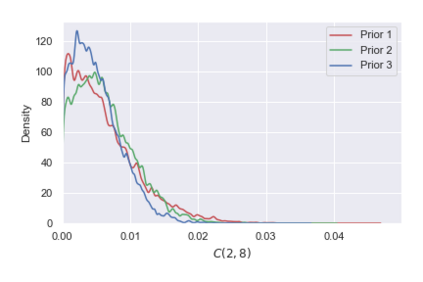
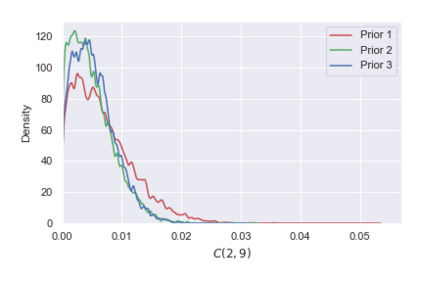
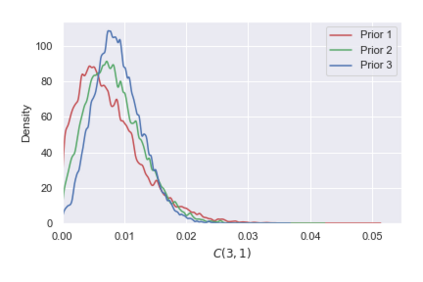
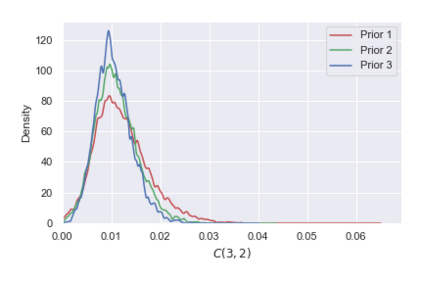
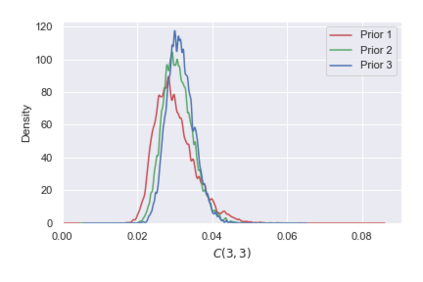
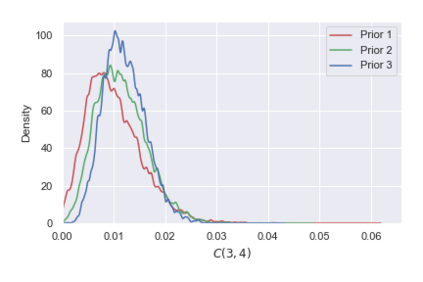
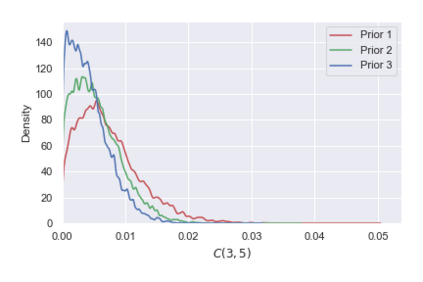
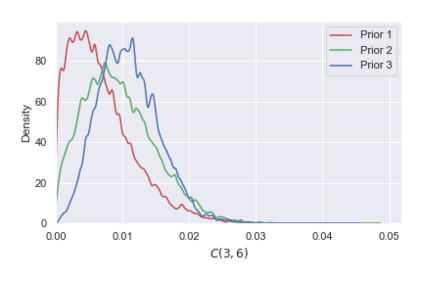
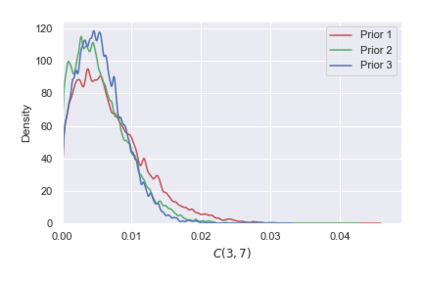
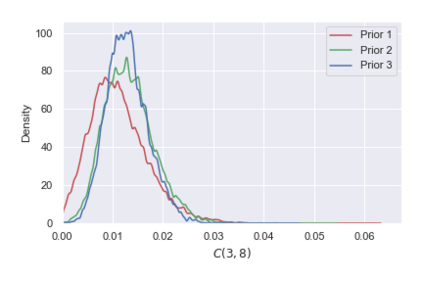
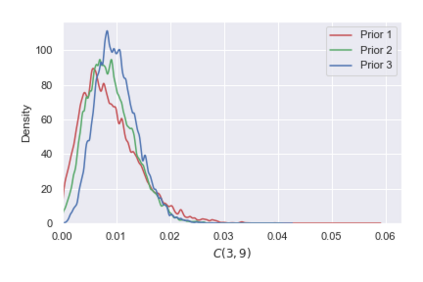
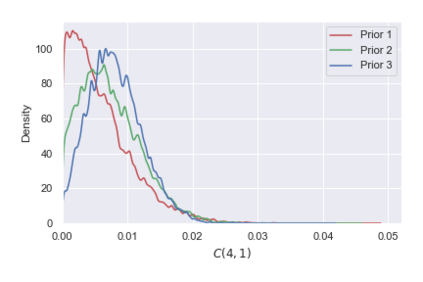
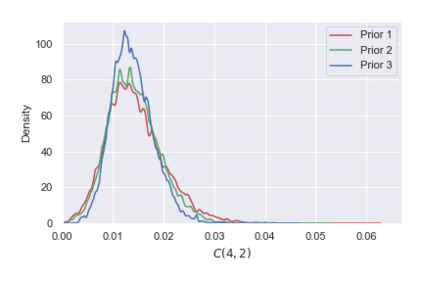
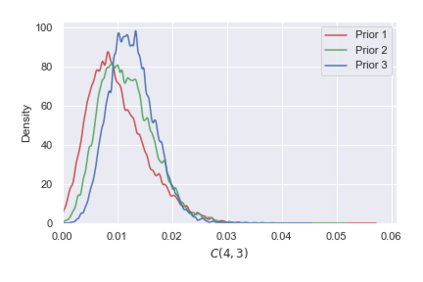
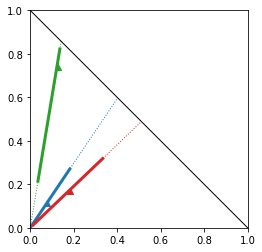
Optimal transport (OT) formalizes the problem of finding an optimal coupling between probability measures given a cost matrix. The inverse problem of inferring the cost given a coupling is Inverse Optimal Transport (IOT). IOT is less well understood than OT. We formalize and systematically analyze the properties of IOT using tools from the study of entropy-regularized OT. Theoretical contributions include characterization of the manifold of cross-ratio equivalent costs, the implications of model priors, and derivation of an MCMC sampler. Empirical contributions include visualizations of cross-ratio equivalent effect on basic examples and simulations validating theoretical results.
翻译:最佳运输(OT)正式确定了在成本矩阵条件下的概率计量方法之间找到最佳组合的问题。假设成本组合的逆向问题是逆向最佳运输(IOT),不甚了解OT。我们利用对英特罗比-正规化的OT的研究工具,正式和系统地分析IOT的特性。理论贡献包括对跨比率等价成本的多重特征描述、模型前科的影响以及一个MCMC取样器的衍生。经验贡献包括对基本实例和模拟验证理论结果的跨比率等价效应的可视化。