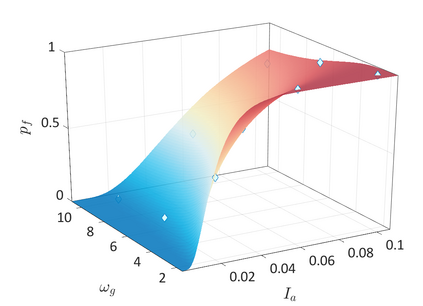
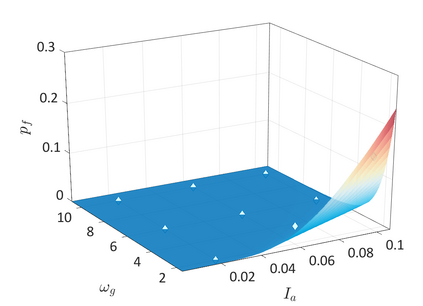
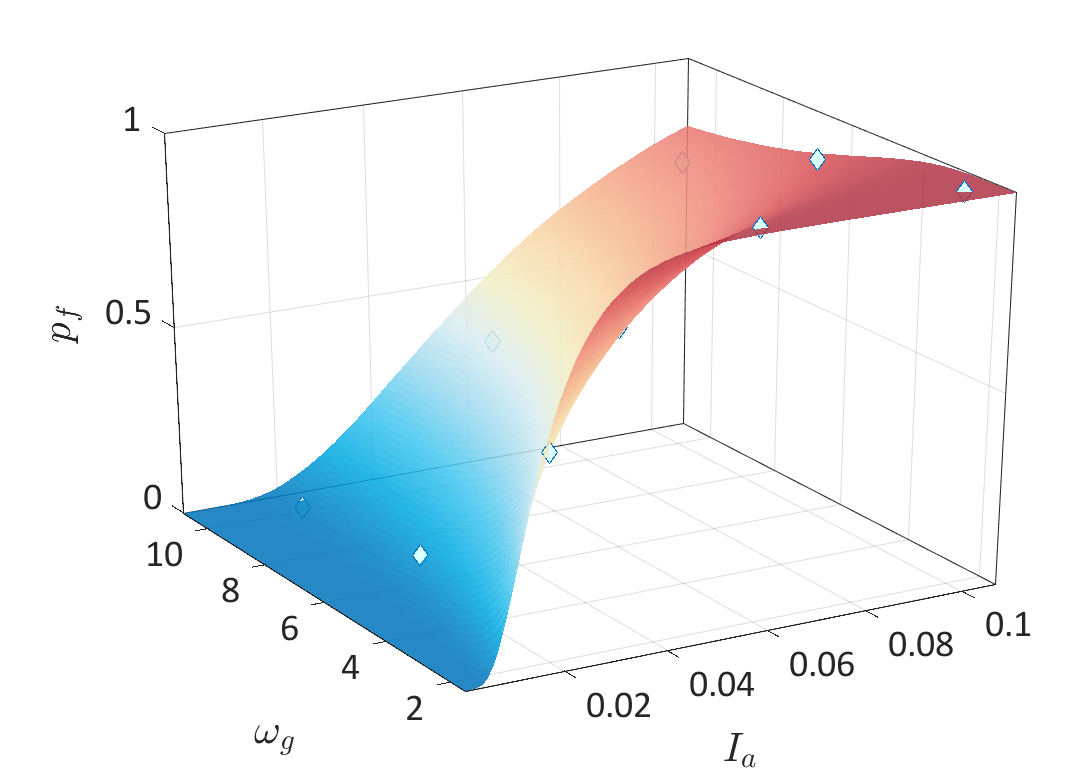
Within the performance-based earthquake engineering (PBEE) framework, the fragility model plays a pivotal role. Such a model represents the probability that the engineering demand parameter (EDP) exceeds a certain safety threshold given a set of selected intensity measures (IMs) that characterize the earthquake load. The-state-of-the art methods for fragility computation rely on full non-linear time-history analyses. Within this perimeter, there are two main approaches: the first relies on the selection and scaling of recorded ground motions; the second, based on random vibration theory, characterizes the seismic input with a parametric stochastic ground motion model (SGMM). The latter case has the great advantage that the problem of seismic risk analysis is framed as a forward uncertainty quantification problem. However, running classical full-scale Monte Carlo simulations is intractable because of the prohibitive computational cost of typical finite element models. Therefore, it is of great interest to define fragility models that link an EDP of interest with the SGMM parameters -- which are regarded as IMs in this context. The computation of such fragility models is a challenge on its own and, despite few recent studies, there is still an important research gap in this domain. This study tackles this computational challenge by using stochastic polynomial chaos expansions to represent the statistical dependence of EDP on IMs. More precisely, this surrogate model estimates the full conditional probability distribution of EDP conditioned on IMs. We compare the proposed approach with some state-of-the-art methods in two case studies. The numerical results show that the new method prevails its competitors in estimating both the conditional distribution and the fragility functions.
翻译:在基于表现的地震工程(PBEE)框架内,脆弱性模型起着关键作用。这种模型代表了工程需求参数(EDP)在地震负荷特点的一组选定强度计量(IMs)中超过一定安全阈值的概率。脆弱性计算的最先进方法依赖于完全的非线性时间历史分析。在此周边,有两种主要方法:第一是选择和缩小已记录的地面运动;第二是随机振动理论,以模拟地面运动模型(SGMMM)为地震输入特征。后一种情况是,鉴于地震风险分析问题被设定为前期不确定性量化问题,因此,典型的全面蒙特卡洛模拟方法取决于完全非线性时间历史分析。因此,非常有必要界定脆弱性模型,将人们感兴趣的电子数据处理模型与该模型的参数联系起来 -- -- 在这种背景下被视为IMMS的参数,这种脆弱性模型的计算方法本身就是一个挑战,尽管最近进行了一些研究,但是在地震风险分析中,地震风险分析问题分析的问题被标定为先期不确定性量化的数值模型,在E-MMS的模型的模型中,这一模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的分布也显示了新的模型的模型的深度分析。我们通过这一模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的模型的尾端径径径径径径径径径。