Physics-informed neural networks for PDE-constrained optimization and control

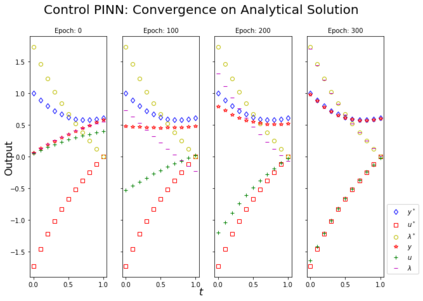
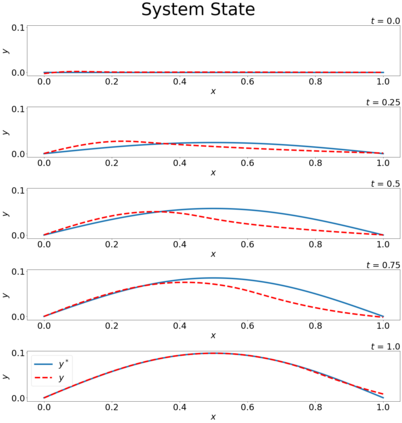
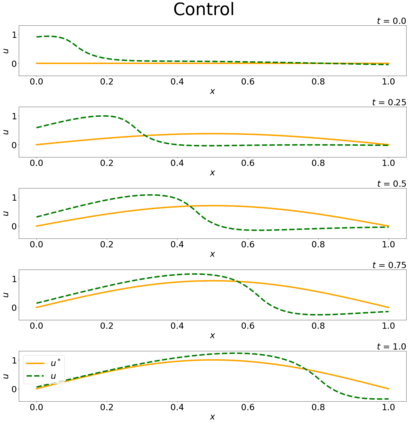
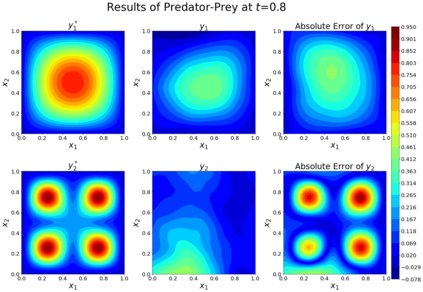
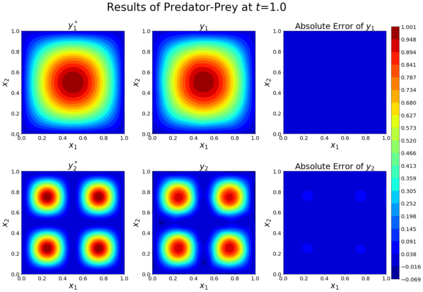
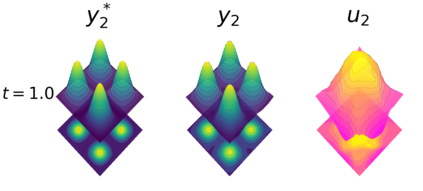
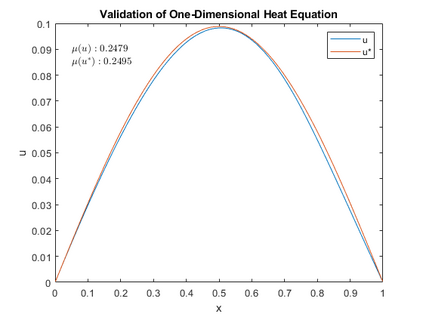
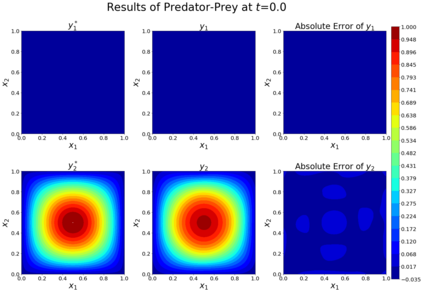
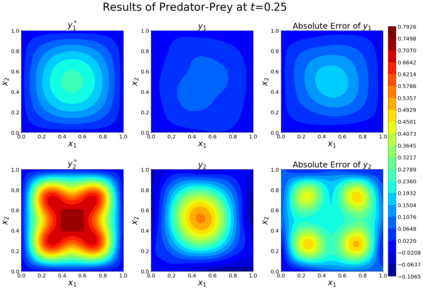
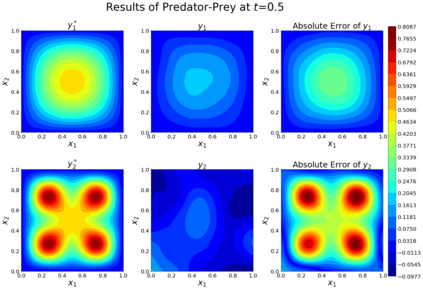
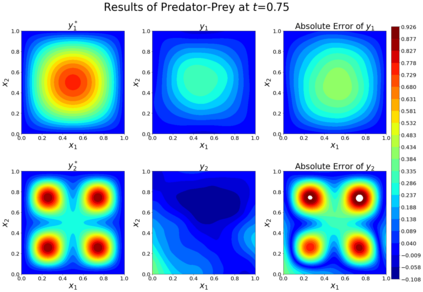
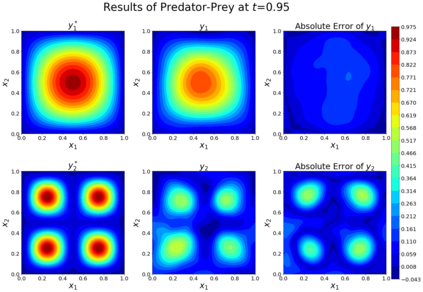
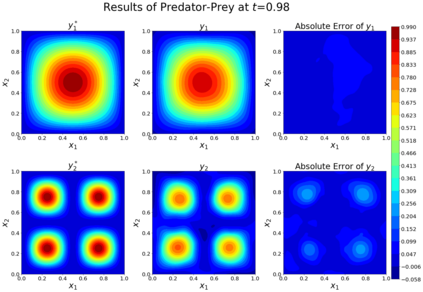
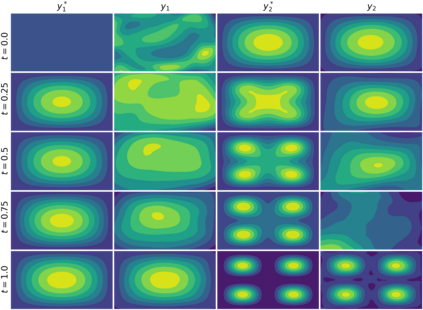
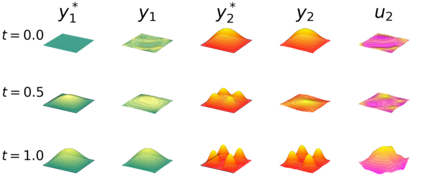
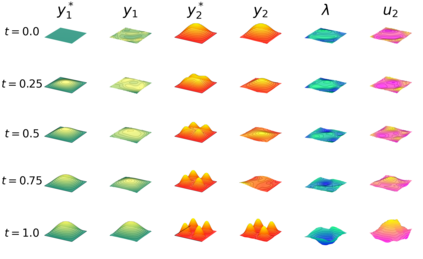
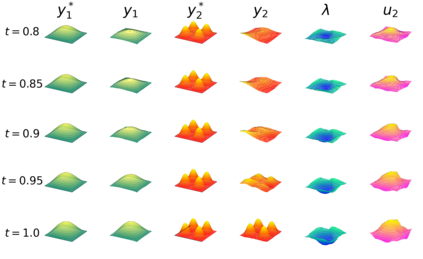
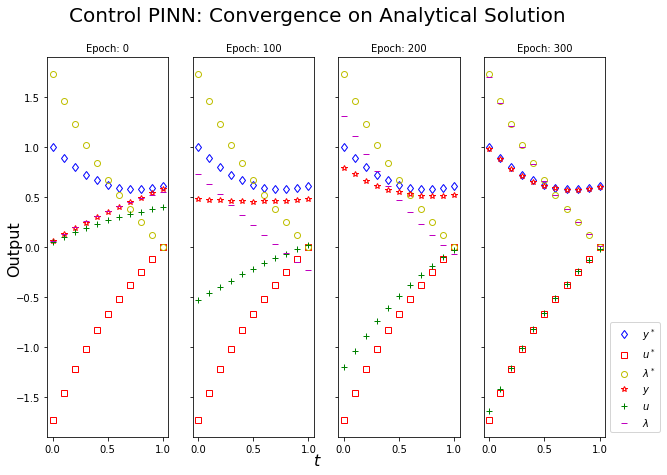
A fundamental problem of science is designing optimal control policies that manipulate a given environment into producing a desired outcome. Control Physics-Informed Neural Networks simultaneously solve a given system state, and its respective optimal control, in a one-stage framework that conforms to physical laws of the system. Prior approaches use a two-stage framework that models and controls a system sequentially, whereas Control PINNs incorporates the required optimality conditions in its architecture and loss function. The success of Control PINNs is demonstrated by solving the following open-loop optimal control problems: (i) an analytical problem (ii) a one-dimensional heat equation, and (iii) a two-dimensional predator-prey problem.