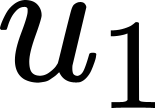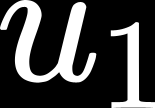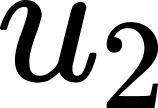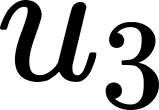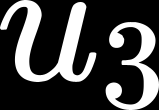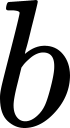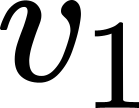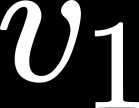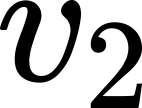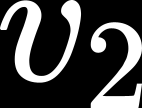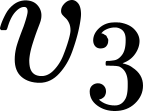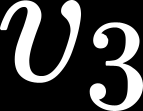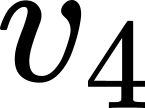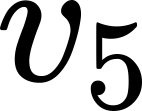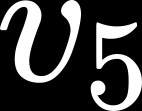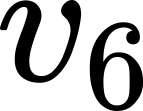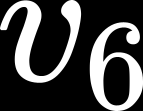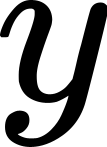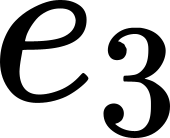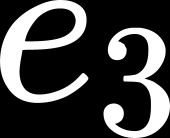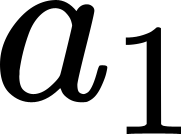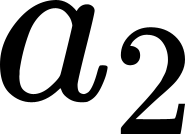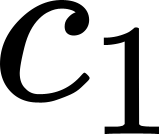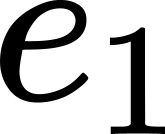The complexity of the Bondage problem in planar graphs

A set $S\subseteq V(G)$ of a graph $G$ is a dominating set if each vertex has a neighbor in $S$ or belongs to $S$. Let $\gamma(G)$ be the cardinality of a minimum dominating set in $G$. The bondage number $b(G)$ of a graph $G$ is the smallest number of edges $A\subseteq E(G)$, such that $\gamma(G-A)=\gamma(G)+1$. The problem of finding $b(G)$ for a graph $G$ is known to be NP-hard even for bipartite graphs. In this paper, we show that deciding if $b(G)=1$ is NP-hard, while deciding if $b(G)=2$ is coNP-hard, even when $G$ is restricted to one of the following classes: planar $3$-regular graphs, planar claw-free graphs with maximum degree $3$, planar bipartite graphs of maximum degree $3$ with girth $k$, for any fixed $k\geq 3$.