A unifying framework for tangential interpolation of structured bilinear control systems

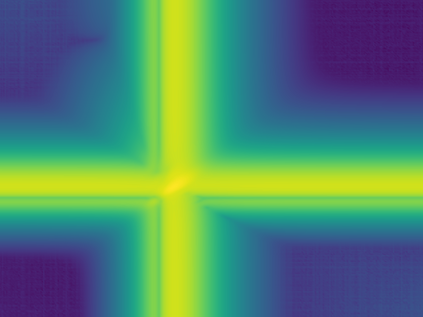
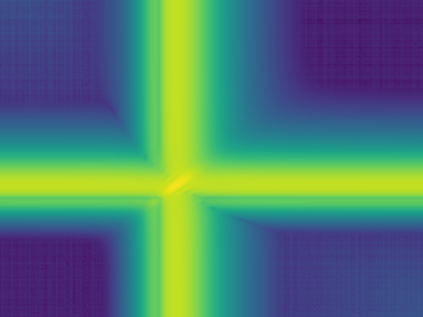
In this paper, we consider the structure-preserving model order reduction problem for multi-input/multi-output bilinear control systems by tangential interpolation. We propose a new type of tangential interpolation problem for structured bilinear systems, for which we develop a new structure-preserving interpolation framework. This new framework extends and generalizes different formulations of tangential interpolation for bilinear systems from the literature and also provides a unifying framework. We then derive explicit conditions on the projection spaces to enforce tangential interpolation in different settings, including conditions for tangential Hermite interpolation. The analysis is illustrated by means of three numerical examples.