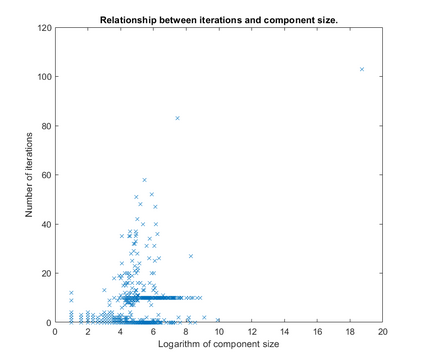
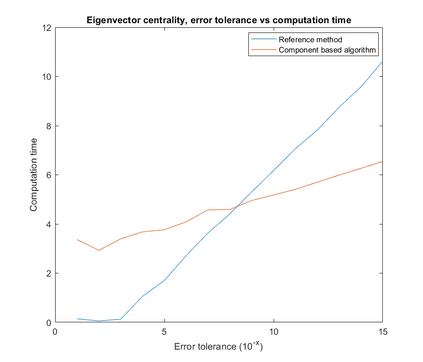
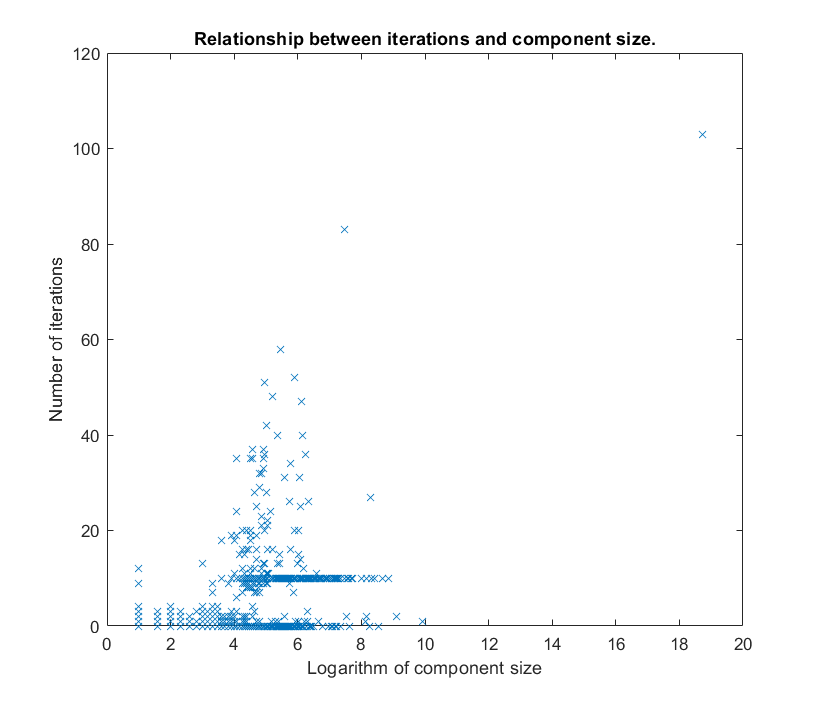
Eigenvector centrality is one of the outstanding measures of central tendency in graph theory. In this paper we consider the problem of calculating eigenvector centrality of graph partitioned into components and how this partitioning can be used. Two cases are considered; first where the a single component in the graph has the dominant eigenvalue, secondly when there are at least two components that share the dominant eigenvalue for the graph. In the first case we implement and compare the method to the usual approach (power method) for calculating eigenvector centrality while in the second case with shared dominant eigenvalues we show some theoretical and numerical results. Keywords: Eigenvector centrality, power iteration, graph, strongly connected component.
翻译:Eigenvector Central 是图形理论中中心趋势的杰出衡量标准之一。 在本文中, 我们考虑了计算图解分割成元件以及如何使用这种分区的问题。 考虑了两个案例: 首先, 图形中的单个元件具有支配的源值, 第二, 至少有两个元件分享图中的主要源值。 在第一个案例中, 我们实施该方法, 并将该方法与通常计算源代码中心的方法( 权力方法) 进行比较, 在第二个案例中, 我们用共享的主要源值来显示一些理论和数字结果 。 关键词 : Eigenvictor Centrity、 动力迭代、 图形、 紧密关联的元件 。